Question: Q. 8. When a parallel beam of monochromatic source of light of wavelength $\lambda$ is incident on a single slit of width $a$, show how the diffraction pattern is formed at the screen by the interference of the wavelets from the slit.
Show that, besides the central maxima at $\theta=0$, secondary maxima are observed at
$$ \theta=\left(n+\frac{1}{2}\right) \frac{\lambda}{a} $$
and the minima at $\theta=\frac{n \lambda}{a}$.
Why do secondary maxima get weaker in intensity with increasing $n$ ?
A
Show Answer
Solution:
Ans. (i)
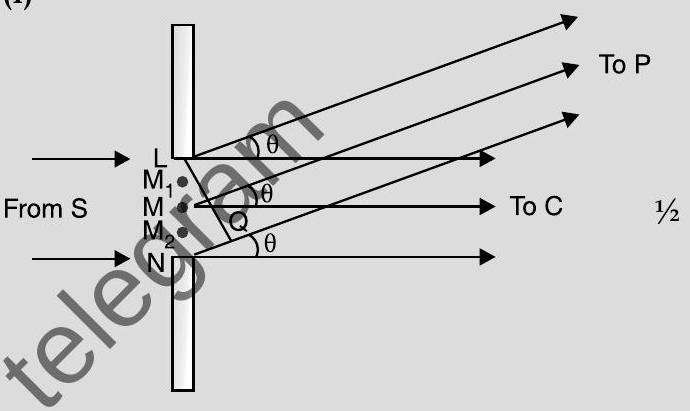
The diffraction pattern formed can be understood by adding the contributions from the different wavelets of the incident wavefront, with their proper phase differences.
For the central point, we imagine the slit to be divided into two equal halves. The contribution of corresponding wavelets, in the two halves, are in phase with each other. Hence we get a maxima at the central point. The entire incident wavefront contributes to this maxima.
$1 / 2$
All other points, for which $\theta=\left(n+\frac{1}{2}\right) \frac{\lambda}{a}$, get a net non zero contribution from all the wavelets. Hence all such points are at the points of maxima. Points for which $\theta=\frac{n \lambda}{a}$, the net contribution, from all the wavelets, is zero. Hence these points are point of minima.
$1 / 2$
We thus get a diffraction pattern on the screen, made up of points of maxima and minima. $1 / 2$
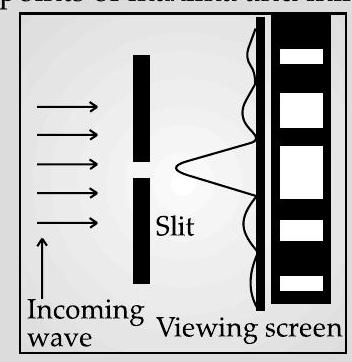
Secondary maxima keep on getting weaker in intensity, with increasing $n$. This is because, at the
(i) First secondary maxima, the net contribution is only from (effectively) $\frac{1}{3}^{\text {rd }}$ of the incident wavefront on the slit. (ii) Second secondary maxima, the net contribution is only from (effectively) $1 / 5^{\text {th }}$ of the incident wavefront on the slit and so on.
[CBSE Marking Scheme 2015]
