Question: Q. 5. (a) Define a wavefront. Using Huygens’ principle, verify the laws of reflection at a plane surface.
(b) In a single slit diffraction experiment, the width of the slit is made double the original width. How does this affect the size and intensity of the central diffraction band ? Explain.
(c) When a tiny circular obstacle is placed in the path of light from a distant source, a bright spot is seen at the centre of the obstacle. Explain why.
[Delhi & OD, 2018]
Show Answer
Solution:
Ans. (a) Definition of wavefront Verification of laws of reflection
(b) Explanation of the effect on the size and intensity of central maxima
(c) Explanation of the bright spot in the shadow of the obstacle
(a) The wavefront may be defined as a surface of constant phase.
[Alternatively : The wave front is the locii of all points that are in the same phase]
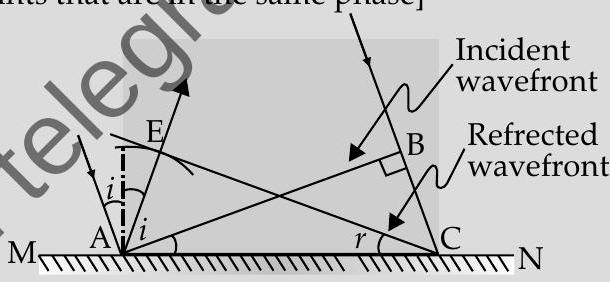
Let speed of the wave in the medium be ’
Let the time taken by the wave front, to advance from point
Let
Distance
(b) Size of central maxima reduces to half,
Intensity increases.
This is because the amount of light, entering the slit, has increased and the area, over which it falls, decreases.
(Also accept if the student just writes that the intensity becomes four fold)
(c) This is because of diffraction of light.
[Alternatively : Light gets diffracted by the tiny circular obstacle and reaches the centre of the shadow of the obstacle.]
[Alternatively : There is a maxima, at the centre of the obstacle, in the diffraction pattern produced by it.]
[CBSE Marking Scheme 2018]
AI Q. 6. (i) Derive an expression for path difference in Young’s double slit experiment and obtain the condition for constructive and destructive interference at a point on the screen.
(ii) The intensity at the central maxima in Young’s double slit experiment is
U] [OD North 2016]
Ans. (i) Try yourself, Similar to Q. 2 (i) LAT Questions.
(ii) Try yourself, Similar to Q. 2 SAT Questions-I






