Solution:

Ans.

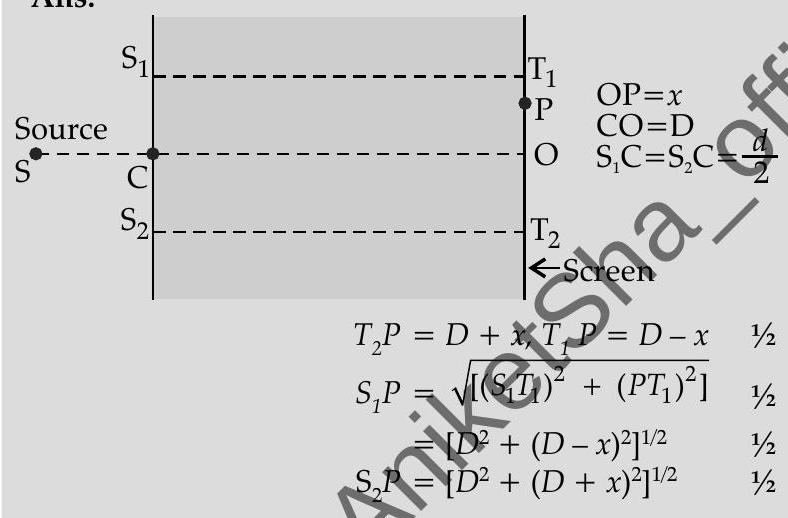
Minima will occur when

$$ \begin{align*} & =\left[D^{2}+(D+x)^{2}\right]^{1 / 2} \ & \quad-\left[D^{2}+(D-x)^{2}\right]^{1 / 2} \ & =\frac{\lambda}{2} \end{align*} $$

If

$\left(D^{2}+4 D^{2}\right)^{1 / 2}-\left(D^{2}\right)^{1 / 2}=\frac{\lambda}{2}$

$\left(5 D^{2}\right)^{1 / 2}-\left(D^{2}\right)^{1 / 2}=\frac{\lambda}{2}$

$D(\sqrt{5}-1)=\frac{\lambda}{2}$

$$ \therefore \quad D=\frac{\lambda}{2(\sqrt{5}-1)} $$

[CBSE Marking Scheme 2016]

[II Q. 7. Explain by drawing a suitable diagram that the interference pattern in a double slit is actually a superposition of single slit diffraction from each slit. Write two basic features which distinguish the interference pattern from those seen in a coherently illuminated single slit. $U$ [Delhi 2015]

Ans. The diagram, given here, shows several fringes, due to double slit interference, ‘contained’ in a broad diffraction peak. When the separation between the slits is large compared to their width, the diffraction pattern becomes very flat and we observe the two slit interference pattern.

1

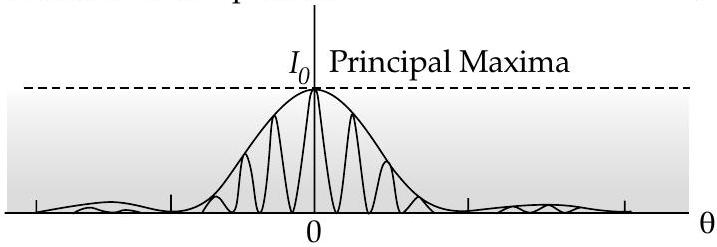
Two basic features :

(i) The interference pattern has a number of equally spaced bright and dark bands while diffraction pattern has a central bright maxima which is twice as wide as the other maxima.

(ii) Interference pattern is the superposition of two waves originating from two narrow slits. The diffraction pattern is a superposition of a continuous family of waves originating from each point on a single slit.

(iii) For a single slit of width ’ $a$ ’ the first null of

2 diffraction pattern occurs at an angle of $\frac{\lambda}{a}$. At the same angle of $\lambda / a$, we get a maxima for two narrow slits separated by a distance $a$.

(Any two) 1