Question: Q. 9. (i) Derive the mathematical expression for the radioactive decay for a sample of a radioactive nucleus.
(ii) How is the mean life of a given radioactive nucleus related to the decay constant? $U$ [OD North 2016]
Show Answer
Solution:
Ans. (i) Let there be $N_{0}$ radioactive nuclei at $t=0$
If $N$ is the number of nuclei left over at $t=t$, we get
or
$$ \begin{aligned} & \frac{-d N}{d t} \propto N \ & \frac{-d N}{d t}=\lambda N \end{aligned} $$
$(\lambda=$ decay constant $) \frac{1}{2}$
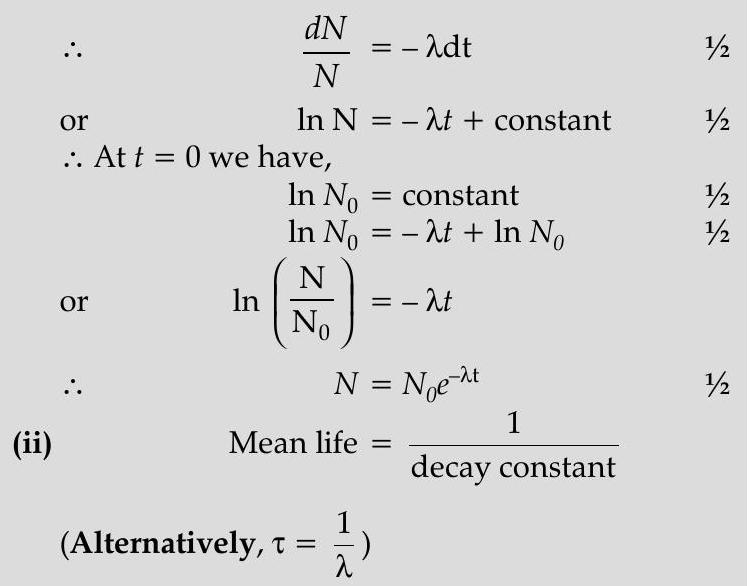
(ii) The half life of ${ }{92}^{238} \mathrm{U}$ undergoing $\alpha$-decay is $4.5 \times$ $10^{9}$ years. Determine the activity of $10 \mathrm{~g}$ sample of ${ }{92}^{238} \mathrm{U}$. Given that $1 \mathrm{~g}$ of ${ }_{92}^{238} \mathrm{U}$ contains $25.3 \times 10^{20}$ atóms.
R A [OD Comptt. I, II, III 2014]
(i) Try yourself, Similar to Q. 7, Short Answer Type Questions-II of this topic.
The S.I. unit of ‘activity’ is becquerel.
(ii) Try yourself, Similar to Q. 8, Short Answer Type Questions-II
Commonly Made Errors
- Few students don’t recall the correct formula.
- A number of students do calculation mistake/put wrong values in the formula.
