Question: Q. 1. The following table shows some measurements of the decay rate of a radio nuclei sample. Find the disintegration constant.
| Time (min) | $\ln \mathbf{R}(\mathbf{B q})$ |
|---|---|
| 36 | 5.08 |
| 100 | 3.29 |
| 164 | 1.52 |
| 218 | 1.00 |
| [SQP 2016] |
Show Answer
Solution:
Ans.
Or
$\ln R=-\lambda t+\ln R_{0}$
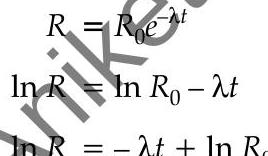
comparing the above equation with line equation $y=m x+c$ where $m$ is slope of line and $c$ is constant.
$$ \begin{aligned} y & =\ln R \ x & =t \ c & =\ln R_{0} \ \text { Slope } & =-\lambda \ \text { Slope } & =\frac{y_{2}-y_{1}}{x_{2}-x_{1}} \ -\lambda & =\frac{1.52-3.29}{164-100} \end{aligned} $$
$$ \begin{align*} -\lambda & =-\frac{1.77}{64} \ \lambda & =0.0280 \text { minutes }^{-1} \tag{1} \end{align*} $$
