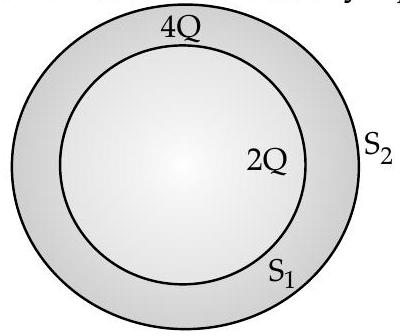
SATHEE: electric-charges-and-fields Question 51

विद्युत आवेश और क्षेत्र

Question: Q. 10. Consider two hollow concentric spheres, $S_{1}$ and $S_{2}$ enclosing charges $2 Q$ and $4 Q$ respectively as shown in the figure. (i) Find out the ratio of the electric flux through them. (ii) How will the electric flux through the sphere $S_{1}$ change if a medium of dielectric constant ’ $ε_{r}$ ’ is introduced in the space inside $S_{1}$ in place of air ? Deduce the necessary expression.

[O.D. I, II, III 2014]

Show Answer

Solution:

Ans. According to the Gauss’s law,

$1 / 2$

$ϕ = \oint \vec{E} \cdot \vec{d S} = \frac{q_{enclosed}}{ε_{0}}$ $∴$ Forsphere $S_{1}$ , flux enclosed $= ϕ_{1} = \frac{2 Q}{ε_{0}} 1 / 2$ nd for sphere $S_{2}$ , flux enclosed $= ϕ_{2} = \frac{2 Q + 4 Q}{ε_{0}}_{1 / 2}$

$\begin{aligned} ϕ_{2} & = \frac{6 Q}{ε_{0}} \frac{ϕ_{1}}{ϕ_{2}} & = \frac{1}{3} \end{aligned}$

$1 / 2$

When a medium of dielectric constant $ε_{r}$ is introduced in the space inside sphere $S_{1}$ , the flux through $S_{1}$ would be $ϕ_{1}^{'} = \frac{2 Q}{ε_{r}}$ .

[CBSE Marking Scheme, 2014]

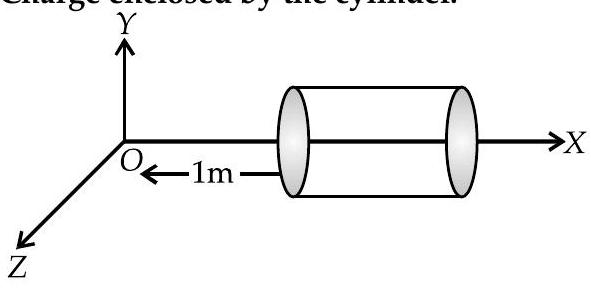
AI Q. 11. A hollow cylindrical box of length $1 m$ and area of cross-section $25 {cm}^{2}$ is placed in a three dimensional coordinate system as shown in the figure. The electric field in the region is given by $\vec{E} = 50 x \hat{i}$ , where $E$ is in ${NC}^{- 1}$ and $x$ is in metres. Find

(i) Net flux through the cylinder.

(ii) Charge enclosed by the cylinder.

A&E [Delhi I, II, III 2013]

Ans. (i) Given : $\vec{E} = 50 x \hat{i}$ and $Δ S = 25 {cm}^{2}$ or $25 \times 10^{- 4} m^{2}$

As the electric field is only along the $x$ -axis, hence, flux will pass only through the cross-section of cylinder.

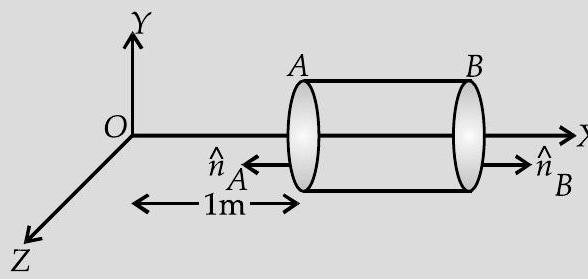
Magnitude of electric field at cross-section $A$ ,

$E_{A} = 50 \times 1 = 50 N / C .$

Magnitude of electric field at cross-section $B$ ,

$E_{B} = 50 \times 2 = 100 N / C$

The corresponding electric fluxes are

$\begin{aligned} \oint ϕ_{A} & = \vec{E} \cdot \vec{Δ S} & = 50 \times 25 \times 10^{- 4} \times \cos 180^{\circ} & = - 0.125 {Nm}^{2} / C^{2} \oint ϕ_{B} & = \vec{E} \cdot \vec{Δ S} & = 100 \times 25 \times 10^{- 4} \times \cos 0^{\circ} & = 0.25 {Nm}^{2} / C^{2} \end{aligned}$

So, the net flux through the cylinder,

$\begin{aligned} \oint ϕ & = \oint ϕ_{A} + \oint ϕ_{B} & = 0.125 + 0.25 & = 0.375 {Nm}^{2} / C^{2} \end{aligned}$

(ii) Using the Gauss’s law,

$\begin{aligned} ϕ & = \oint \vec{E} \cdot \vec{d s} = \frac{q}{ε_{0}} \Rightarrow 0.375 & = \frac{q}{8.85 \times 10^{- 12}} \Rightarrow q & = 8.85 \times 10^{- 12} \times 0.375 & = 3.3 \times 10^{- 12} C . & [C B S E Marking Scheme, 2013] \end{aligned}$

विद्युत आवेश और क्षेत्र

विषयसूची

इंजीनियरिंग की तैयारी

चिकित्सा तैयारी

एनसीईआरटी पुस्तकें और समाधान

महत्वपूर्ण संसाधन

अन्य संसाधन

पाठ्यक्रम

परीक्षा मंच

नमूना मॉक टेस्ट

सदस्यता

एनसीईआरटी व्यायाम वीडियो समाधान

विद्युत आवेश और क्षेत्र

विषयसूची

इंजीनियरिंग की तैयारी

चिकित्सा तैयारी

एनसीईआरटी पुस्तकें और समाधान

महत्वपूर्ण संसाधन

अन्य संसाधन

पाठ्यक्रम

परीक्षा मंच

नमूना मॉक टेस्ट

सदस्यता

एनसीईआरटी व्यायाम वीडियो समाधान

Menu