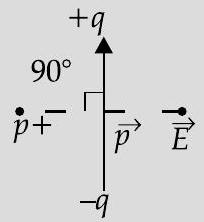
Question: Q. 4. An electric dipole is placed in a uniform electric
field $\vec{E}$ with its dipole moment $\vec{p}$ parallel to the field. Find
(i) the work done in turning the dipole till its dipole moment points in the direction opposite to $\vec{E}$.
(ii) the orientation of the dipole for which the torque acting on it becomes maximum.
A [Comptt. O.D. I, II, III 2014]
Show Answer
Solution:
Ans. (i) We have $\quad W=\int_{\theta_{1}}^{\theta_{2}} \tau d \theta$
$\therefore \quad W=\int_{0}^{\pi} p E \sin \theta d \theta$
$W=p E[-\cos \theta]_{0}^{\pi}$
$W=-2 p E$
$\tau=p E \sin \theta$
(ii) For $\theta=\frac{\pi}{2}, \tau$ is maximum.
[CBSE Marking Scheme, 2014]
