Question: Q. 6. (i) The potential difference applied across a given resistor is altered so that the heat produced per second increases by a factor of 9 . By what factor does the applied potential difference change?
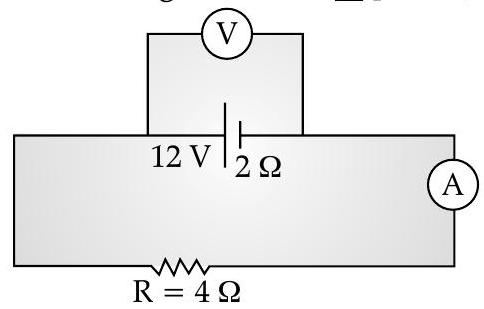
(ii) In the figure shown, an ammeter
R [O.D. I, II, III 2017]
Show Answer
Solution:
Ans. (i) The factor by which the potential difference changes
(ii) Ammeter Reading 1
(iii) Voltmeter Reading
(iii) Voltmeter Reading,
(Alternatively,
[CBSE Marking Scheme 2017]
Detailed Answer :
(i) Let the original potential difference be V volts with heat generated as
Now heat generated will be :
Take the new potential difference as
Change in heat produced :
But from the question, if the heat produced by a factor of 9 , so
Hence, it is clear that the applied potential difference increases by factor 3 .
[AI Q. 7. Define relaxation time of the free electrons drifting in a conductor. How is it related to the drift velocity of free electrons? Use this relation to deduce the expression for the electrical resistivity of the material.
R [O.D. I, II, III 2012]
Ans. Relaxation time
Relation
1
[CBSE Marking Scheme, 2012]






