Question: Q. 5. (i) Draw a labelled diagram of
(ii) A circular coil of cross-sectional area
U] [Delhi I, II, III 2017]
Show Answer
Solution:
Ans. (i) Labelled diagram of
(ii) Calculation of maximum value of current
(i) Labelled diagram :
Try yourself, Similar to Q. 3(a), Long Answer Type Questions
(ii) Maximum value of emf

Maximum induced current,
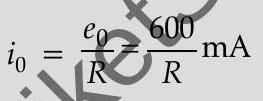
[Note 1 : If the student calculates the value of the maximum induced emf and says that “since
[Note 2 : The direction of magnetic field has not been given. If the student takes this direction along the axis of rotation and hence obtains the value of induced emf and, therefore, maximum current, as zero, award full marks for this part.]
[CBSE Marking Scheme 2017]
Detailed Answer :
(i) Expression :
If at any moment,
If
Maximum value or peak value of instantaneous induced emf
So,
As value of sine function varies from +1 to -1 , polarity of emf changes with time. Also the output voltage is sinusoidalin nature.
1






