Question: Q. 4. A device
(a) Identify the device
(b) Draw graphs showing variation of voltage and current with time over one cycle of
(c) How does the reactance of the device
Show Answer
Solution:
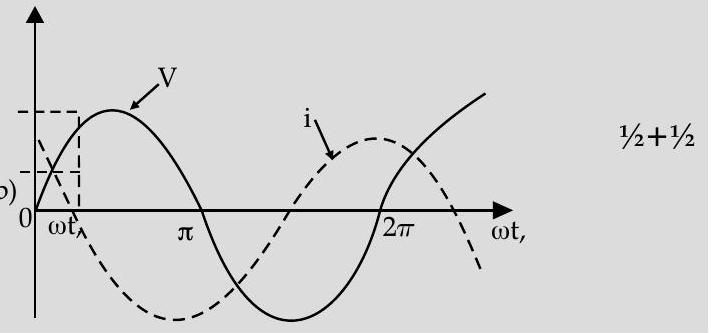
Ans. (b) Graphs of voltage and current with time 1+1
(c) Variation of reactance with frequency (Graphical variation)
(d) Phasor Diagram
(a)
Reactance,
(b)
(c) Reactance of the capacitor varies in inverse proportion to the frequency i.e. , 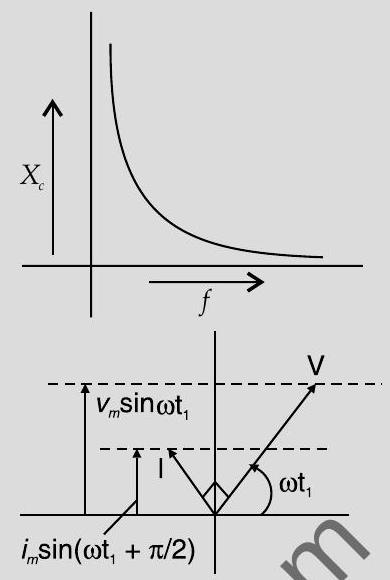
[CBSE Marking Scheme 2018]
TOPIC-2
LCR Series Circuit
Revision Notes
- In an LCR series circuit with resistor, inductor andcapacitor, the expression for the instantaneous potential difference between the terminals
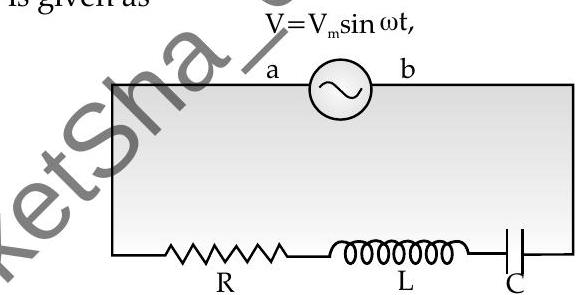
The potential difference in this will be equal to the sum of the potential differences across
where,
The steady state solution will be
where,
From the equation, steady-state current like terminal voltage, varies sinusoidal with time, so steady-state current can be written as
- In an LCR circuit :
Here,
For steady-state currents, maximum current
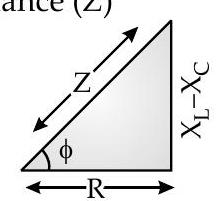
Total effective resistance of
The angle by which alternating voltage leads the alternating current in
In an
When a condenser of capacity
In LCR circuit, if there is no loss of energy, then totalenergy in
and at every instant will remain constant.
Resonance
Circuit in which inductance L,Capacitance
The necessary conditionfor resonance in
series circuit is :
In this, frequency of
The sharpness of tuning at resonance is measured by
At series LCR resonance or acceptor circuit, current is maximum.
Power in AC circuits
where
where,
Power factor
- The value of power factor varies from 0 to 1 .
The instantaneous rate at which energy is supplied to an electrical device by ac circuit is
Average power in
Wattless Current
- The average power associated over a complete cycle with pure inductor or pare capacitor is zero which makes current through
In
circuit at resonance, the power loss is maximum, so
Dattless component of current
Phase angle : It is the amount by which the voltage and current are of phase with each other in a circuit.
Power factor : It is the amount by which the power delivered in the circuit is less than the theoretical maximum of the circuit due to voltage and current being out of phase.
Quality factor : It is a dimensionless quantity that shows sharphess of the peak of bandwidth.
Resonant frequency : It is the frequency at which the amplitude of the current is maximum where circuit oscillates when not driven by voltage source.
Know the Formulae
- Impedance for a series
Average power,
Power factor,
Quality factor






