Chapter-06 Application of Derivatives
With the Calculus as a key, Mathematics can be successfully applied to the explanation of the course of Nature." - WHITEHEAD
6.1 Introduction
In Chapter 5, we have learnt how to find derivative of composite functions, inverse trigonometric functions, implicit functions, exponential functions and logarithmic functions. In this chapter, we will study applications of the derivative in various disciplines, e.g., in engineering, science, social science, and many other fields. For instance, we will learn how the derivative can be used (i) to determine rate of change of quantities, (ii) to find the equations of tangent and normal to a curve at a point, (iii) to find turning points on the graph of a function which in turn will help us to locate points at which largest or smallest value (locally) of a function occurs. We will also use derivative to find intervals on which a function is increasing or decreasing. Finally, we use the derivative to find approximate value of certain quantities.
6.2 Rate of Change of Quantities
Recall that by the derivative $\\ \frac{ds}{dt} $, we mean the rate of change of distance $s$ with respect to the time $t$. In a similar fashion, whenever one quantity $y$ varies with another quantity $x$, satisfying some rule $y=f(x)$, then $\frac{d y}{d x}$ (or $f^{\prime}(x)$ ) represents the rate of change of $y$ with respect to $x$ and $\frac{d y}{d x} _{x=x_0}(.$ or $.f^{\prime}(x_0))$ represents the rate of change of $y$ with respect to $x$ at $x=x_0$.
Further, if two variables $x$ and $y$ are varying with respect to another variable $t$, i.e., if $x=f(t)$ and $y=g(t)$, then by Chain Rule
$$ \frac{d y}{d x}=\frac{d y}{d t} / \frac{d x}{d t}, \text{ if } \frac{d x}{d t} \neq 0 $$
Thus, the rate of change of $y$ with respect to $x$ can be calculated using the rate of change of $y$ and that of $x$ both with respect to $t$.
Let us consider some examples.
Example 1 Find the rate of change of the area of a circle per second with respect to its radius $r$ when $r=5 cm$.
Solution The area A of a circle with radius $r$ is given by $A=\pi r^{2}$. Therefore, the rate of change of the area A with respect to its radius $r$ is given by $\frac{d A}{d r}=\frac{d}{d r}(\pi r^{2})=2 \pi r$. When $r=5 cm, \frac{d A}{d r}=10 \pi$. Thus, the area of the circle is changing at the rate of $10 \pi cm^{2} / s$.
Example 2 The volume of a cube is increasing at a rate of 9 cubic centimetres per second. How fast is the surface area increasing when the length of an edge is 10 centimetres?
Solution Let $x$ be the length of a side, $V$ be the volume and $S$ be the surface area of the cube. Then, $V=x^{3}$ and $S=6 x^{2}$, where $x$ is a function of time $t$.
Now $ \qquad \frac{d V}{d t}=9 cm^{3} / s$ (Given)
Therefore $ \qquad 9=\frac{d V}{d t}=\frac{d}{d t}(x^{3})=\frac{d}{d x}(x^{3}) \cdot \frac{d x}{d t} \quad(\text{ By Chain Rule })$
or $ \qquad =3 x^{2} \cdot \frac{d x}{d t} $
Now $ \qquad \frac{d x}{d t}=\frac{3}{x^{2}} \tag{1}$
$$ \begin{array}{rlr} \frac{d S}{d t} & =\frac{d}{d t}\left(6 x^{2}\right)=\frac{d}{d x}\left(6 x^{2}\right) \cdot \frac{d x}{d t} & \text { (By Chain Rule) } \\ & =12 x \cdot\left(\frac{3}{x^{2}}\right)=\frac{36}{x} & \text { (Using (1) ) } \end{array} $$
Hence, when $ x=10 \mathrm{~cm}, \frac{d S}{d t}=3.6 \mathrm{~cm}^{2} / \mathrm{s} $
Example 3 A stone is dropped into a quiet lake and waves move in circles at a speed of $4 cm$ per second. At the instant, when the radius of the circular wave is $10 cm$, how fast is the enclosed area increasing?
Solution The area $A$ of a circle with radius $r$ is given by $A=\pi r^{2}$. Therefore, the rate of change of area A with respect to time $t$ is
$$ \frac{d \mathrm{~A}}{d t}=\frac{d}{d t}\left(\pi r^{2}\right)=\frac{d}{d r}\left(\pi r^{2}\right) \cdot \frac{d r}{d t}=2 \pi r \frac{d r}{d t} $$
It is given that $\frac{d r}{d t}=4 \mathrm{~cm}$
Therefore, $ r=10 \mathrm{~cm} $ $ \frac{d \mathrm{~A}}{d t}=2 \pi(10)(4)=80 \pi $
Thus, the enclosed area is increasing at the rate of $80 \pi cm^{2} / s$, when $r=10 cm$.
Note $\frac{d y}{d x}$ is positive if $y$ increases as $x$ increases and is negative if $y$ decreases as $x$ increases.
Example 4 The length $x$ of a rectangle is decreasing at the rate of $3 cm /$ minute and the width $y$ is increasing at the rate of $2 cm /$ minute. When $x=10 cm$ and $y=6 cm$, find the rates of change of (a) the perimeter and (b) the area of the rectangle.
Solution Since the length $x$ is decreasing and the width $y$ is increasing with respect to time, we have
$$ \frac{d x}{d t}=-3 \mathrm{~cm} / \mathrm{min} \text { or } \frac{d y}{d t}=2 \mathrm{~cm} / \mathrm{min} $$
(a) The perimeter $P$ of a rectangle is given by
$$ \mathrm{P}=2(x+y) $$
Therefore $ \frac{d \mathrm{P}}{d t}=2\left(\frac{d x}{d t}+\frac{d y}{d t}\right)=2(-3+2)=-2 \mathrm{~cm} / \mathrm{min} $
(b) The area $A$ of the rectangle is given by
$ A=x \cdot y $
Therefore $ \begin{aligned} \frac{d \mathrm{~A}}{d t} & =\frac{d x}{d t} \cdot y+x \cdot \frac{d y}{d t} \\ & =-3(6)+10(2)(\text { क्योंकि } x=10 \mathrm{~cm} \text { और } y=6 \mathrm{~cm}) \\ & =2 \mathrm{~cm}^{2} / \mathrm{min} \end{aligned} $
Example 5 The total cost $C(x)$ in Rupees, associated with the production of $x$ units of an item is given by
$$ C(x)=0.005 x^{3}-0.02 x^{2}+30 x+5000 $$
Find the marginal cost when 3 units are produced, where by marginal cost we mean the instantaneous rate of change of total cost at any level of output.
Solution Since marginal cost is the rate of change of total cost with respect to the output, we have
$ \begin{aligned} \text{ Marginal } \qquad \mathrm{MC} & =\frac{d \mathrm{C}}{d x}=0.005\left(3 x^{2}\right)-0.02(2 x)+30 \\ \text{ When } \qquad \mathrm{MC} & =0.015\left(3^{2}\right)-0.04(3)+30 \\ & =0.135-0.12+30=30.015 \end{aligned} $
Hence, the required marginal cost is ₹ 30.02 (nearly).
Example 6 The total revenue in Rupees received from the sale of $x$ units of a product is given by $R(x)=3 x^{2}+36 x+5$. Find the marginal revenue, when $x=5$, where by marginal revenue we mean the rate of change of total revenue with respect to the number of items sold at an instant.
Solution Since marginal revenue is the rate of change of total revenue with respect to the number of units sold, we have
$ \begin{aligned} \text{ Marginal Revenue } \qquad (MR) & =\frac{d R}{d x}=6 x+36 \end{aligned} $ $ \begin{aligned} \text{ when } \qquad x & =5, MR=6(5)+36=66 \end{aligned} $
Hence, the required marginal revenue is ₹ 66 .
EXERCISE 6.1
1. Find the rate of change of the area of a circle with respect to its radius $r$ when
$\quad\quad$(a) $r=3 cm$ $\quad\quad$ (b) $r=4 cm$
Show Answer
Solution
The area of a circle ( $A$ ) with radius $(r)$ is given by,
$A=\pi r^{2}$
Now, the rate of change of the area with respect to its radius is given by,
$\frac{d A}{d r}=\frac{d}{d r}(\pi r^{2})=2 \pi r$
1. When $r=3 cm$,
$\frac{d A}{d r}=2 \pi(3)=6 \pi$
Hence, the area of the circle is changing at the rate of $6 cm^{2} / s$ when its radius is $3 cm$.
2. When $r=4 cm$,
$\frac{d A}{d r}=2 \pi(4)=8 \pi$
Hence, the area of the circle is changing at the rate of $8 cm^{2} / s$ when its radius is $4 cm$.
2. The volume of a cube is increasing at the rate of $8 cm^{3} / s$. How fast is the surface area increasing when the length of an edge is $12 cm$ ?
Show Answer
Solution
Let $x$ be the length of a side, $V$ be the volume, and $s$ be the surface area of the cube.
Then, $V=x^{3}$ and $S=6 x^{2}$ where $x$ is a function of time $t$.
It is given that $\frac{d V}{d t}=8 cm^{3} / s$.
Then, by using the chain rule, we have:
$ \therefore=\frac{d V}{d t}=\frac{d}{d t}(x^{3})=\frac{d}{d x}(x^{3}) \cdot \frac{d x}{d t}=3 x^{2} \cdot \frac{d x}{d t} $
$ \begin{equation*} \Rightarrow \frac{d x}{d t}=\frac{8}{3 x^{2}} \tag{1} \end{equation*} $
$ \begin{aligned} & \text{ Now, } \frac{d S}{d t}=\frac{d}{d t}(6 x^{2})=\frac{d}{d x}(6 x^{2}) \cdot \frac{d x}{d t} \quad \text{ [By chain rule] } \\ & =12 x \cdot \frac{d x}{d t}=12 x \cdot(\frac{8}{3 x^{2}})=\frac{32}{x} \end{aligned} $
Hence, if the length of the edge of the cube is $12 cm$, then the surface area is increasing
at the rate of $\frac{8}{3} cm^{2} / s$.
3. The radius of a circle is increasing uniformly at the rate of $3 cm / s$. Find the rate at which the area of the circle is increasing when the radius is $10 cm$.
Show Answer
Solution
The area of a circle $(A)$ with radius $(r)$ is given by,
$A=\pi r^{2}$
Now, the rate of change of area $(A)$ with respect to time $(t)$ is given by,
$\frac{d A}{d t}=\frac{d}{d t}(\pi r^{2}) \cdot \frac{d r}{d t}=2 \pi r \frac{d r}{d t} \quad$ [By chain rule]
It is given that,
$\frac{d r}{d t}=3 cm / s$ $\therefore \frac{d A}{d t}=2 \pi r(3)=6 \pi r$
Thus, when $r=10 cm$,
$\frac{d A}{d t}=6 \pi(10)=60 \pi cm^{2} / s$
Hence, the rate at which the area of the circle is increasing when the radius is $10 cm$ is $60 п cm^{2} / s$.
4. An edge of a variable cube is increasing at the rate of $3 cm / s$. How fast is the volume of the cube increasing when the edge is $10 cm$ long?
Show Answer
Solution
Let $x$ be the length of a side and $V$ be the volume of the cube. Then,
$V=x^{3}$.
$\therefore \frac{d V}{d t}=3 x^{2} \cdot \frac{d x}{d t}$ (By chain rule)
It is given that,
$\frac{d x}{d t}=3 cm / s$
$\therefore \frac{d V}{d t}=3 x^{2}(3)=9 x^{2}$
Thus, when $x=10 cm$,
$\frac{d V}{d t}=9(10)^{2}=900 cm^{3} / s$
Hence, the volume of the cube is increasing at the rate of $900 cm^{3} / s$ when the edge is 10 $cm$ long.
5. A stone is dropped into a quiet lake and waves move in circles at the speed of $5 cm / s$. At the instant when the radius of the circular wave is $8 cm$, how fast is the enclosed area increasing?
Show Answer
Solution
The area of a circle (A) with radius $(r)$ is given by $A=\pi r^{2}$.
Therefore, the rate of change of area $(A)$ with respect to time $(t)$ is given by,
$\frac{d A}{d t}=\frac{d}{d t}(\pi r^{2})=\frac{d}{d r}(\pi r^{2}) \frac{d r}{d t}=2 \pi r \frac{d r}{d t}$ [By chain rule]
It is given that $\frac{d r}{d t}=5 cm / s$.
Thus, when $r=8 cm$,
$\frac{d A}{d t}=2 \pi(8)(5)=80 \pi$
Hence, when the radius of the circular wave is $8 cm$, the enclosed area is increasing at the rate of $80 \pi cm^{2} / s$.
6. The radius of a circle is increasing at the rate of $0.7 cm / s$. What is the rate of increase of its circumference?
Show Answer
Solution
The circumference of a circle $(C)$ with radius $(r)$ is given by $C=2 \pi r$.
Therefore, the rate of change of circumference (C) with respect to time $(t)$ is given by, $\frac{d C}{d t}=\frac{d C}{d r} \cdot \frac{d r}{d t}$ (By chain rule) $=\frac{d}{d r}(2 \pi r) \frac{d r}{d t}$
$=2 \pi \cdot \frac{d r}{d t}$
It is given that $\frac{d r}{d t}=0.7 cm / s$.
Hence, the rate of increase of the circumference is $2 \pi(0.7)=1.4 \pi cm / s$.
7. The length $x$ of a rectangle is decreasing at the rate of $5 cm /$ minute and the width $y$ is increasing at the rate of $4 cm /$ minute. When $x=8 cm$ and $y=6 cm$, find the rates of change of (a) the perimeter, and (b) the area of the rectangle.
Show Answer
Solution
Since the length $(x)$ is decreasing at the rate of $5 cm /$ minute and the width $(y)$ is increasing at the rate of $4 cm /$ minute, we have:
$\frac{d x}{d t}=-5 cm / min$ and $\frac{d y}{d t}=4 cm / min$
(a) The perimeter $(P)$ of a rectangle is given by,
$P=2(x+y)$
$\therefore \frac{d P}{d t}=2(\frac{d x}{d t}+\frac{d y}{d t})=2(-5+4)=-2 cm / min$
Hence, the perimeter is decreasing at the rate of $2 cm / min$.
(b) The area (A) of a rectangle is given by,
$A=x \times y$
$\therefore \frac{d A}{d t}=\frac{d x}{d t} \cdot y+x \cdot \frac{d y}{d t}=-5 y+4 x$
When $x=8 cm$ and $y=6 cm, \frac{d A}{d t}=(-5 \times 6+4 \times 8) cm^{2} / min=2 cm^{2} / min$
Hence, the area of the rectangle is increasing at the rate of $2 cm^{2} / min$.
8. A balloon, which always remains spherical on inflation, is being inflated by pumping in 900 cubic centimetres of gas per second. Find the rate at which the radius of the balloon increases when the radius is $15 cm$.
Show Answer
Solution
The volume of a sphere $(V)$ with radius $(r)$ is given by,
$V=\frac{4}{3} \pi r^{3}$
$\therefore$ Rate of change of volume $(V)$ with respect to time $(t)$ is given by,
$\frac{d V}{d t}=\frac{d V}{d r} \cdot \frac{d r}{d t} _{\text{[By chain rule] }}$
$=\frac{d}{d r}(\frac{4}{3} \pi r^{3}) \cdot \frac{d r}{d t}$
$=4 \pi r^{2} \cdot \frac{d r}{d t}$
It is given that $\frac{d V}{d t}=900 cm^{3} / s$.
$\therefore 900=4 \pi r^{2} \cdot \frac{d r}{d t}$
$\Rightarrow \frac{d r}{d t}=\frac{900}{4 \pi r^{2}}=\frac{225}{\pi r^{2}}$
Therefore, when radius $=15 cm$,
$\frac{d r}{d t}=\frac{225}{\pi(15)^{2}}=\frac{1}{\pi}$
Hence, the rate at which the radius of the balloon increases when the radius is $15 cm$
$1 cm / s$.
is $\pi$
9. A balloon, which always remains spherical has a variable radius. Find the rate at which its volume is increasing with the radius when the later is $10 cm$.
Show Answer
Solution
The volume of a sphere $(V)$ with radius $(r)$ is given by $V=\frac{4}{3} \pi r^{3}$.
Rate of change of volume $(V)$ with respect to its radius $(r)$ is given by,
$\frac{d V}{d r}=\frac{d}{d r}(\frac{4}{3} \pi r^{3})=\frac{4}{3} \pi(3 r^{2})=4 \pi r^{2}$
Therefore, when radius $=10 cm$,
$\frac{d V}{d r}=4 \pi(10)^{2}=400 \pi$
Hence, the volume of the balloon is increasing at the rate of $400 cm^{3} / s$.
10. A ladder $5 m$ long is leaning against a wall. The bottom of the ladder is pulled along the ground, away from the wall, at the rate of $2 cm / s$. How fast is its height on the wall decreasing when the foot of the ladder is $4 m$ away from the wall?
Show Answer
Solution
Let $y m$ be the height of the wall at which the ladder touches. Also, let the foot of the ladder be $x$ maway from the wall.
Then, by Pythagoras theorem, we have:
$x^{2}+y^{2}=25$ [Length of the ladder $=5 m$ ]
$\Rightarrow y=\sqrt{25-x^{2}}$
Then, the rate of change of height $(y)$ with respect to time $(t)$ is given by,
$\frac{d y}{d t}=\frac{-x}{\sqrt{25-x^{2}}} \cdot \frac{d x}{d t}$
It is given that $\frac{d x}{d t}=2 cm / s$. $\therefore \frac{d y}{d t}=\frac{-2 x}{\sqrt{25-x^{2}}}$
Now, when $x=4 m$, we have:
$\frac{d y}{d t}=\frac{-2 \times 4}{\sqrt{25-4^{2}}}=-\frac{8}{3}$
Hence, the height of the ladder on the wall is decreasing at the rate of $\frac{8}{3} cm / s$.
11. A particle moves along the curve $6 y=x^{3}+2$. Find the points on the curve at which the $y$-coordinate is changing 8 times as fast as the $x$-coordinate.
Show Answer
Solution
The equation of the curve is given as:
$6 y=x^{3}+2$
The rate of change of the position of the particle with respect to time $(t)$ is given by,
$6 \frac{d y}{d t}=3 x^{2} \frac{d x}{d t}+0$
$\Rightarrow 2 \frac{d y}{d t}=x^{2} \frac{d x}{d t}$
When the $y$-coordinate of the particle changes 8 times as fast as the
$x$-coordinate i.e., $(\frac{d y}{d t}=8 \frac{d x}{d t})$, we have:
$2(8 \frac{d x}{d t})=x^{2} \frac{d x}{d t}$
$\Rightarrow 16 \frac{d x}{d t}=x^{2} \frac{d x}{d t}$
$\Rightarrow(x^{2}-16) \frac{d x}{d t}=0$
$\Rightarrow x^{2}=16$
$\Rightarrow x= \pm 4$
When $x=4, y=\frac{4^{3}+2}{6}=\frac{66}{6}=11$.
When $x=-4, y=\frac{(-4)^{3}+2}{6}=-\frac{62}{6}=-\frac{31}{3}$.
Hence, the points required on the curve are $(4,11)$ and $(-4, \frac{-31}{3})$.
12. The radius of an air bubble is increasing at the rate of $\frac{1}{2} cm / s$. At what rate is the volume of the bubble increasing when the radius is $1 cm$ ?
Show Answer
Solution
The air bubble is in the shape of a sphere.
Now, the volume of an air bubble $(V)$ with radius $(r)$ is given by,
$V=\frac{4}{3} \pi r^{3}$
The rate of change of volume $(V)$ with respect to time $(t)$ is given by,
$ \begin{matrix} \frac{d V}{d t} & =\frac{4}{3} \pi \frac{d}{d r}(r^{3}) \cdot \frac{d r}{d t} & \text{ [By chain rule] } \\ & =\frac{4}{3} \pi(3 r^{2}) \frac{d r}{d t} & \\ & =4 \pi r^{2} \frac{d r}{d t} & \end{matrix} $
It is given that $\frac{d r}{d t}=\frac{1}{2} cm / s$.
Therefore, when $r=1 cm$,
$\frac{d V}{d t}=4 \pi(1)^{2}(\frac{1}{2})=2 \pi cm^{3} / s$
Hence, the rate at which the volume of the bubble increases is $2 \pi cm^{3} / s$.
13. A balloon, which always remains spherical, has a variable diameter $\frac{3}{2}(2 x+1)$. Find the rate of change of its volume with respect to $x$.
Show Answer
Solution
The volume of a sphere $(V)$ with radius $(r)$ is given by,
$V=\frac{4}{3} \pi r^{3}$
It is given that:
Diameter $=\frac{3}{2}(2 x+1)$
$\Rightarrow r=\frac{3}{4}(2 x+1)$
$\therefore V=\frac{4}{3} \pi(\frac{3}{4})^{3}(2 x+1)^{3}=\frac{9}{16} \pi(2 x+1)^{3}$
Hence, the rate of change of volume with respect to $x$ is as
$ \frac{d V}{d x}=\frac{9}{16} \pi \frac{d}{d x}(2 x+1)^{3}=\frac{9}{16} \pi \times 3(2 x+1)^{2} \times 2=\frac{27}{8} \pi(2 x+1)^{2} . $
14. Sand is pouring from a pipe at the rate of $12 cm^{3} / s$. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is $4 cm$ ?
Show Answer
Solution
The volume of a cone $(V)$ with radius $(r)$ and height $(h)$ is given by,
$V=\frac{1}{3} \pi r^{2} h$
It is given that,
$h=\frac{1}{6} r \Rightarrow r=6 h$
$\therefore V=\frac{1}{3} \pi(6 h)^{2} h=12 \pi h^{3}$
The rate of change of volume with respect to time $(t)$ is given by,
$\frac{d V}{d t}=12 \pi \frac{d}{d h}(h^{3}) \cdot \frac{d h}{d t}$ [By chain rule]
$=12 \pi(3 h^{2}) \frac{d h}{d t}$
$=36 \pi h^{2} \frac{d h}{d t}$
It is also given that $\frac{d V}{d t}=12 cm^{3} / s$.
Therefore, when $h=4 cm$, we have:
$ \begin{aligned} & 12=36 \pi(4)^{2} \frac{d h}{d t} \\ & \Rightarrow \frac{d h}{d t}=\frac{12}{36 \pi(16)}=\frac{1}{48 \pi} \end{aligned} $
Hence, when the height of the sand cone is $4 cm$, its height is increasing at the rate
of $\frac{1}{48 \pi} cm / s$.
15. The total cost $C(x)$ in Rupees associated with the production of $x$ units of an item is given by
$\quad\quad$$ C(x)=0.007 x^{3}-0.003 x^{2}+15 x+4000 . $
Find the marginal cost when 17 units are produced.
Show Answer
Solution
Marginal cost is the rate of change of total cost with respect to output.
$\therefore$ Marginal cost (MC) $=\frac{d C}{d x}=0.007(3 x^{2})-0.003(2 x)+15$
$=0.021 x^{2}-0.006 x+15$
When $x=17, M C=0.021(17^{2})-0.006(17)+15$ $=0.021(289)-0.006(17)+15$
$=6.069-0.102+15$
$=20.967$
Hence, when 17 units are produced, the marginal cost is Rs. 20.967.
16. The total revenue in Rupees received from the sale of $x$ units of a product is given by
$\quad\quad$$ R(x)=13 x^{2}+26 x+15 . $
Find the marginal revenue when $x=7$.
Show Answer
Solution
Marginal revenue is the rate of change of total revenue with respect to the number of units sold.
$\therefore$ Marginal Revenue (MR) $=\frac{d R}{d x}=13(2 x)+26=26 x+26$
When $x=7$,
$M R=26(7)+26=182+26=208$
Hence, the required marginal revenue is Rs 208.
Choose the correct answer for questions 17 and 18.
17. The rate of change of the area of a circle with respect to its radius $r$ at $r=6 cm$ is
(A) $10 \pi$
(B) $12 \pi$
(C) $8 \pi$
(D) $11 \pi$
Show Answer
Solution
The area of a circle ( $A$ ) with radius $(r)$ is given by,
$A=\pi r^{2}$
Therefore, the rate of change of the area with respect to its radius $r$ is
$\frac{d A}{d r}=\frac{d}{d r}(\pi r^{2})=2 \pi r$. $\therefore$ When $r=6 cm$,
$\frac{d A}{d r}=2 \pi \times 6=12 \pi cm^{2} / s$
Hence, the required rate of change of the area of a circle is $12 \pi cm^{2} / s$.
The correct answer is $B$.
18. The total revenue in Rupees received from the sale of $x$ units of a product is given by
$R(x)=3 x^{2}+36 x+5$. The marginal revenue, when $x=15$ is
(A) 116
(B) 96
(C) 90
(D) 126
Show Answer
Solution
Marginal revenue is the rate of change of total revenue with respect to the number of units sold.
$\therefore$ Marginal Revenue (MR) $=\frac{d R}{d x}=3(2 x)+36=6 x+36$
$\therefore$ When $x=15$,
$M R=6(15)+36=90+36=126$
Hence, the required marginal revenue is Rs 126 .
The correct answer is D.
6.3 Increasing and Decreasing Functions
In this section, we will use differentiation to find out whether a function is increasing or decreasing or none.
Consider the function $f$ given by $f(x)=x^{2}, x \in \mathbf{R}$. The graph of this function is a parabola as given in Fig 6.1.
Values left to origin
| $x$ | $f(x)=x^{2}$ |
|---|---|
| -2 | 4 |
| $-\frac{3}{2}$ | $\frac{9}{4}$ |
| -1 | 1 |
| $-\frac{1}{2}$ | $\frac{1}{4}$ |
| 0 | 0 |
as we move from left to right, the height of the graph decreases

as we move from left to right, the height of the graph increases Values right to origin
| x | $ f(x)=x^2 $ |
|---|---|
| 0 | 0 |
| $ \frac{1}{4} $ | $ \frac{1}{4} $ |
| 1 | 1 |
| $ \frac{3}{2} $ | $ \frac{9}{4} $ |
| 2 | 4 |
First consider the graph (Fig 6.1) to the right of the origin. Observe that as we move from left to right along the graph, the height of the graph continuously increases. For this reason, the function is said to be increasing for the real numbers $x>0$.
Now consider the graph to the left of the origin and observe here that as we move from left to right along the graph, the height of the graph continuously decreases. Consequently, the function is said to be decreasing for the real numbers $x<0$.
We shall now give the following analytical definitions for a function which is increasing or decreasing on an interval.
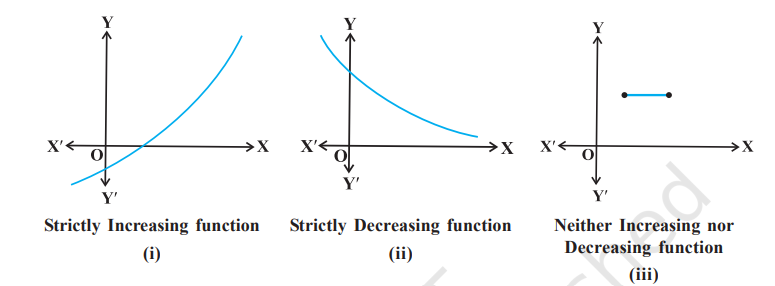
Definition 1 Let I be an interval contained in the domain of a real valued function $f$. Then $f$ is said to be
(i) increasing on I if $x_1<x_2$ in $I \Rightarrow f(x_1)<f(x_2)$ for all $x_1, x_2 \in I$.
(ii) decreasing on $I$, if $x_1, x_2$ in $I \Rightarrow f(x_1)<f(x_2)$ for all $x_1, x_2 \in I$.
(iii) constant on $I$, if $f(x)=c$ for all $x \in I$, where $c$ is a constant.
(iv) decreasing on I if $x_1<x_2$ in $I \Rightarrow f(x_1) \geq f(x_2)$ for all $x_1, x_2 \in I$.
(v) strictly decreasing on I if $x_1<x_2$ in $I \Rightarrow f(x_1)>f(x_2)$ for all $x_1, x_2 \in I$.
For graphical representation of such functions see Fig 6.2.
We shall now define when a function is increasing or decreasing at a point.
Definition 2 Let $x_0$ be a point in the domain of definition of a real valued function $f$. Then $f$ is said to be increasing, decreasing at $x_0$ if there exists an open interval I containing $x_0$ such that $f$ is increasing, decreasing, respectively, in I.
Let us clarify this definition for the case of increasing function.
Example 7 Show that the function given by $f(x)=7 x-3$ is increasing on $\mathbf{R}$.
Solution Let $x_1$ and $x_2$ be any two numbers in $\mathbf{R}$. Then
$$ \begin{aligned} x _{1}<x _{2} & \Rightarrow 7 x _{1}<7 x _{2} \\ & \Rightarrow 7 x _{1}-3<7 x _{2}-3 \\ & \Rightarrow f\left(x _{1}\right)<f\left(x _{2}\right) \end{aligned} $$
Thus, by Definition 1, it follows that $f$ is strictly increasing on $\mathbf{R}$.
We shall now give the first derivative test for increasing and decreasing functions. The proof of this test requires the Mean Value Theorem studied in Chapter 5.
Theorem 1 Let $f$ be continuous on $[a, b]$ and differentiable on the open interval $(a, b)$. Then
(a) $f$ is increasing in $[a, b]$ if $f^{\prime}(x)>0$ for each $x \in(a, b)$
(b) $f$ is decreasing in $[a, b]$ if $f^{\prime}(x)<0$ for each $x \in(a, b)$
(c) $f$ is a constant function in $[a, b]$ if $f^{\prime}(x)=0$ for each $x \in(a, b)$
Proof (a) Let $x_1, x_2 \in[a, b]$ be such that $x_1<x_2$.
Then, by Mean Value Theorem (Theorem 8 in Chapter 5), there exists a point $c$ between $x_1$ and $x_2$ such that
$$ f\left(x _{2}\right)-f\left(x _{1}\right)=f^{\prime}(c)\left(x _{2}-x _{1}\right) $$
i.e. $\begin{array}{ll} f\left(x _{2}\right)-f\left(x _{1}\right)>0 & \left(\text { given } f^{\prime}(c)>0\right) \end{array}$
i.e. $f(x_2)>f(x_1)$
Thus, we have $x_1<x_2 \quad f(x_1) \quad f(x_2), \text{ for all } x_1, x_2 \quad[a, b]$
Hence, $f$ is an increasing function in $[a, b]$.
The proofs of part (b) and (c) are similar. It is left as an exercise to the reader.
Remarks
There is a more generalised theorem, which states that if $f \phi(x)>0$ for $x$ in an interval excluding the end points and $f$ is continuous in the interval, then $f$ is increasing. Similarly, if $f \phi(x)<0$ for $x$ in an interval excluding the end points and $f$ is continuous in the interval, then $f$ is decreasing.
Example 8 Show that the function $f$ given by
is increasing on $\mathbf{R}$.
$$ f(x)=x^{3}-3 x^{2}+4 x, x \in \mathbf{R} $$
Solution Note that
$$ \begin{aligned} f^{\prime}(x) & =3 x^{2}-6 x+4 \\ & =3(x^{2}-2 x+1)+1 \\ & =3(x-1)^{2}+1>0, \text{ in every interval of } \mathbf{R} \end{aligned} $$
Therefore, the function $f$ is increasing on $\mathbf{R}$.
Example 9 Prove that the function given by $f(x)=\cos x$ is
(a) decreasing in $(0, \pi)$
(b) increasing in $(\pi, 2 \pi)$, and
(c) neither increasing nor decreasing in $(0,2 \pi)$.
Solution Note that $f^{\prime}(x)=-\sin x$
(a) Since for each $x \in(0, \pi), \sin x>0$, we have $f^{\prime}(x)<0$ and so $f$ is decreasing in $(0, \pi)$.
(b) Since for each $x \in(\pi, 2 \pi)$, $\sin x<0$, we have $f^{\prime}(x)>0$ and so $f$ is increasing in $(\pi, 2 \pi)$.
(c) Clearly by (a) and (b) above, $f$ is neither increasing nor decreasing in $(0,2 \pi)$.
Example 10 Find the intervals in which the function $f$ given by $f(x)=x^{2}-4 x+6$ is (a) increasing (b) decreasing
Solution We have
$$ f(x)=x^{2}-4 x+6 $$ $ or \qquad f^{\prime}(x)=2 x-4 $
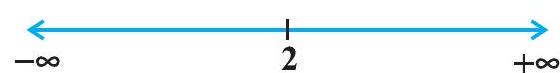
Therefore, $f^{\prime}(x)=0$ gives $x=2$. Now the point $x=2$ divides the real line into two disjoint intervals namely, $(-\infty, 2)$ and $(2, \infty)$ (Fig 6.3). In the interval $(-\infty, 2), f^{\prime}(x)=2 x$ $-4<0$.
Therefore, $f$ is decreasing in this interval. Also, in the interval $(2, \infty), f^{\prime}(x)>0$ and so the function $f$ is increasing in this interval.
Example 11 Find the intervals in which the function $f$ given by $f(x)=4 x^{3}-6 x^{2}-72 x$ +30 is (a) increasing (b) decreasing.
Solution We have
$$ \text{ or } \quad \begin{aligned} f(x) & =4 x^{3}-6 x^{2}-72 x+30 \\ f^{\prime}(x) & =12 x^{2}-12 x-72 \\ & =12(x^{2}-x-6) \\ & =12(x-3)(x+2) \end{aligned} $$
Therefore, $f^{\prime}(x)=0$ gives $x=-2,3$. The points $x=-2$ and $x=3$ divides the real line into three disjoint intervals, namely, $(-\infty,-2),(-2,3)$

Fig 6.4 and $(3, \infty)$.
In the intervals $(-\infty,-2)$ and $(3, \infty), f^{\prime}(x)$ is positive while in the interval $(-2,3)$, $f^{\prime}(x)$ is negative. Consequently, the function $f$ is increasing in the intervals $(-\infty,-2)$ and $(3, \infty)$ while the function is decreasing in the interval $(-2,3)$. However, $f$ is neither increasing nor decreasing in $\mathbf{R}$.
| Interval | Sign of $ f’(x) $ | Nature of function $ f $ |
|---|---|---|
| $ (\infty, -2) $ | (-)(-)>0 | $ f $ is increasing |
| (-2,3) | (-)(+)<0 | $ f $ is decreasing |
| $ (3,\infty)$ | (+)(+)>0 | $ f $ is increasing |
Example 12 Find intervals in which the function given by $f(x)=\sin 3 x, x \in 0, \frac{\pi}{2}$ is (a) increasing (b) decreasing.
Solution We have
$f(x) =\sin 3 x $
or $\quad f(x) =3 \cos 3 x$
Therefore, $f^{\prime}(x)=0$ gives $\cos 3 x=0$ which in turn gives $3 x=\frac{\pi}{2}, \frac{3 \pi}{2}$ (as $x \in 0, \frac{\pi}{2}$ implies $3 x \in[0, \frac{3 \pi}{2}]$ ). So $x=\frac{\pi}{6}$ and $\frac{\pi}{2}$. The point $x=\frac{\pi}{6}$ divides the interval $0, \frac{\pi}{2}$ into two disjoint intervals $[0, \frac{\pi}{6})$ and $\frac{\pi}{6}, \frac{\pi}{2}$.
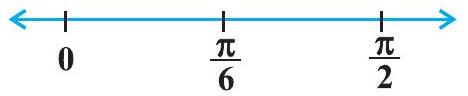
Fig 6.5
Now, $f^{\prime}(x)>0$ for all $x \in[0, \frac{\pi}{6})$ as $0 \leq x<\frac{\pi}{6} \Rightarrow 0 \leq 3 x<\frac{\pi}{2}$ and $f^{\prime}(x)<0$ for all $x \in(\frac{\pi}{6}, \frac{\pi}{2})$ as $\frac{\pi}{6}<x<\frac{\pi}{2} \Rightarrow \frac{\pi}{2}<3 x<\frac{3 \pi}{2}$.
Therefore, $f$ is increasing in $[0, \frac{\pi}{6})$ and decreasing in $(\frac{\pi}{6}, \frac{\pi}{2})$.
Also, the given function is continuous at $x=0$ and $x=\frac{\pi}{6}$. Therefore, by Theorem 1 , $f$ is increasing on $ [0, \frac{\pi}{6}]$ and decreasing on $[\frac{\pi}{6}, \frac{\pi}{2}]$.
Example 13 Find the intervals in which the function $f$ given by
$ f(x)=\sin x+\cos x, 0 \leq x \leq 2 \pi $
is increasing or decreasing.
Solution We have
$$ \begin{array}{lrlr} & f(x) & =\sin x+\cos x, \quad 0 \leq x \leq 2 \pi \\ \text{or }&f^{\prime}(x) & =\cos x-\sin x & \end{array} $$
Now $f^{\prime}(x)=0$ gives $\sin x=\cos x$ which gives that $x=\frac{\pi}{4}, \frac{5 \pi}{4}$ as $0 \leq x \leq 2 \pi$
The points $x=\frac{\pi}{4}$ and $x=\frac{5 \pi}{4}$ divide the interval $[0,2 \pi]$ into three disjoint intervals,
namely, $[0, \frac{\pi}{4}), \frac{\pi}{4}, \frac{5 \pi}{4}$ and $(\frac{5 \pi}{4}, 2 \pi]$.
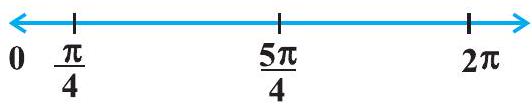
Fig 6.6
Note that $f^{\prime}(x)>0$ if $x \in[0, \frac{\pi}{4}) \cup(\frac{5 \pi}{4}, 2 \pi]$
or $\quad f$ is increasing in the intervals $[0, \frac{\pi}{4})$ and $(\frac{5 \pi}{4}, 2 \pi]$
Also $\quad f^{\prime}(x)<0$ if $x \in \frac{\pi}{4}, \frac{5 \pi}{4}$
or $\quad f$ is decreasing in $\frac{\pi}{4}, \frac{5 \pi}{4}$
| Interval | Sign of $ f $ ‘(x) | Nature of function |
|---|---|---|
| [$ 0, \frac{\pi}{4} $] | >0 | $ f $ is increasing |
| $ (\frac{\pi}{4}, \frac{5\pi}{4}) $ | <0 | $ f $ is decreasing |
| $ (\frac{5 \pi}{4},2\pi $ | >0 | $ f $ is increasing |
EXERCISE 6.2
1. Show that the function given by $f(x)=3 x+17$ is increasing on $\mathbf{R}$.
Show Answer
Solution
Let ${ }^{x_1}$ and $x_2$ be any two numbers in $\mathbf{R}$.
Then, we have:
$x_1<x_2 \Rightarrow 3 x_1<3 x_2 \Rightarrow 3 x_1+17<3 x_2+17 \Rightarrow f(x_1)<f(x_2)$
Hence, $f$ is strictly increasing on $\mathbf{R}$.
Alternate method:
$f(x)=3>0$, in every interval of $\mathbf{R}$.
Thus, the function is strictly increasing on $\mathbf{R}$.
2. Show that the function given by $f(x)=e^{2 x}$ is increasing on $\mathbf{R}$.
Show Answer
Solution
Let ${ }^{x_1}$ and $x_2$ be any two numbers in $\mathbf{R}$.
Then, we have:
$x_1<x_2 \Rightarrow 2 x_1<2 x_2 \Rightarrow e^{2 x_1}<e^{2 x_2} \Rightarrow f(x_1)<f(x_2)$
Hence, $f$ is strictly increasing on $\mathbf{R}$.
3. Show that the function given by $f(x)=\sin x$ is
(a) increasing in $(0, \frac{\pi}{2})$
(b) decreasing in $(\frac{\pi}{2}, \pi)$
(c) neither increasing nor decreasing in $(0, \pi)$
Show Answer
Solution
The given function is $f(x)=\sin x$.
$\therefore f^{\prime}(x)=\cos x$ (a) Since for each $x \in(0, \frac{\pi}{2}), \cos x>0$, we have $f^{\prime}(x)>0$.
Hence, $f$ is strictly increasing in $(0, \frac{\pi}{2})$.
(b) Since for each $x \in(\frac{\pi}{2}, \pi), \cos x<0$, we have $f^{\prime}(x)<0$.
Hence, $f$ is strictly decreasing in $(\frac{\pi}{2}, \pi)$.
(c) From the results obtained in (a) and (b), it is clear that $f$ is neither increasing nor decreasing in $(0, \pi)$.
4. Find the intervals in which the function $f$ given by $f(x)=2 x^{2}-3 x$ is
(a) increasing
(b) decreasing
Show Answer
Solution
The given function is $f(x)=2 x^{2}-3 x$.
$f^{\prime}(x)=4 x-3$
$\therefore f^{\prime}(x)=0 \Rightarrow x=\frac{3}{4}$
Now, the point $\frac{3}{4}$ divides the real line into two disjoint intervals i.e., $(-\infty, \frac{3}{4})$ and $(\frac{3}{4}, \infty)$.

In interval $(-\infty, \frac{3}{4}), f^{\prime}(x)=4 x-3<0$.
Hence, the given function $(f)$ is strictly decreasing in interval $(-\infty, \frac{3}{4})$.
In interval $(\frac{3}{4}, \infty), f^{\prime}(x)=4 x-3>0$.
Hence, the given function $(f)$ is strictly increasing in interval $(\frac{3}{4}, \infty)$.
5. Find the intervals in which the function $f$ given by $f(x)=2 x^{3}-3 x^{2}-36 x+7$ is
(a) increasing
(b) decreasing
Show Answer
Solution
The given function is $f(x)=2 x^{3}-3 x^{2}-36 x+7$.
$f^{\prime}(x)=6 x^{2}-6 x-36=6(x^{2}-x-6)=6(x+2)(x-3)$
$\therefore f^{\prime}(x)=0 \Rightarrow x=-2,3$
The points $x=-2$ and $x=3$ divide the real line into three disjoint intervals i.e., $(-\infty,-2),(-2,3)$, and $(3, \infty)$.

In intervals $(-\infty,-2)$ and $(3, \infty), f^{\prime}(x)$ is positive while in interval
$(-2,3), f^{\prime}(x)$ is negative.
Hence, the given function $(f)$ is strictly increasing in intervals
$(-\infty,-2)$ and $(3, \infty)$, while function $(f)$ is strictly decreasing in interval
$(-2,3)$.
6. Find the intervals in which the following functions are strictly increasing or decreasing:
(a) $x^{2}+2 x-5$
(b) $10-6 x-2 x^{2}$
(c) $-2 x^{3}-9 x^{2}-12 x+1$
(d) $6-9 x-x^{2}$
(e) $(x+1)^{3}(x-3)^{3}$
Show Answer
Solution
(a) We have,
$f(x)=x^{2}+2 x-5$
$\therefore f^{\prime}(x)=2 x+2$
Now,
$f^{\prime}(x)=0 \Rightarrow x=-1$
Point $x=-1$ divides the real line into two disjoint intervals i.e., $(-\infty,-1)$ and $(-1, \infty)$.
In interval $(-\infty,-1), f^{\prime}(x)=2 x+2<0$.
$\therefore f$ is strictly decreasing in interval $(-\infty,-1)$.
Thus, $f$ is strictly decreasing for $x<-1$.
In interval $(-1, \infty), f^{\prime}(x)=2 x+2>0$.
$\therefore f$ is strictly increasing in interval $(-1, \infty)$.
Thus, $f$ is strictly increasing for $x>-1$.
(b) We have,
$f(x)=10-6 x-2 x^{2}$
$\therefore f^{\prime}(x)=-6-4 x$
Now,
$f^{\prime}(x)=0 \Rightarrow x=-\frac{3}{2}$
The point $x=-\frac{3}{2}$ divides the real line into two disjoint intervals
i.e., $(-\infty,-\frac{3}{2})$ and $(-\frac{3}{2}, \infty)$.
In interval $(-\infty,-\frac{3}{2})$ i.e., when $x<-\frac{3}{2}, f^{\prime}(x)=-6-4 x<0$.
$\therefore f$ is strictly increasing for $x<-\frac{3}{2}$.
In interval $(-\frac{3}{2}, \infty)$ i.e., when $x>-\frac{3}{2}, f^{\prime}(x)=-6-4 x<0$.
$\therefore f$ is strictly decreasing for $x>-\frac{3}{2}$.
(c) We have,
$f(x)=-2 x^{3}-9 x^{2}-12 x+1$
$\therefore f^{\prime}(x)=-6 x^{2}-18 x-12=-6(x^{2}+3 x+2)=-6(x+1)(x+2)$
Now,
$f^{\prime}(x)=0 \Rightarrow x=-1$ and $x=-2$
Points $x=-1$ and $x=-2$ divide the real line into three disjoint intervals
i.e., $(-\infty,-2),(-2,-1)$, and $(-1, \infty)$.
In intervals $(-\infty,-2)$ and $(-1, \infty)$ i.e., when $x<-2$ and $x>-1$,
$f^{\prime}(x)=-6(x+1)(x+2)<0$. $\therefore f$ is strictly decreasing for $x<-2$ and $x>-1$.
Now, in interval $(-2,-1)$ i.e., when $-2<x<-1, f^{\prime}(x)=-6(x+1)(x+2)>0$.
$\therefore f$ is strictly increasing for $-2<x<-1$.
(d) We have,
$ \begin{aligned} & f(x)=6-9 x-x^{2} \\ & \therefore f^{\prime}(x)=-9-2 x \end{aligned} $
Now, $f^{\prime}$
$(x)=0$ gives $x=-\frac{9}{2}$
The point $x=-\frac{9}{2}$ divides the real line into two disjoint intervals i.e.,
$(-\infty,-\frac{9}{2})$ and $(-\frac{9}{2}, \infty)$.
In interval $(-\infty,-\frac{9}{2})$ i.e., for $x<-\frac{9}{2}, f^{\prime}(x)=-9-2 x>0$.
$\therefore f$ is strictly increasing for $x<-\frac{9}{2}$.
In interval $(-\frac{9}{2}, \infty)$ i.e., for $x>-\frac{9}{2}, f^{\prime}(x)=-9-2 x<0$. $\therefore f$ is strictly decreasing for $x>-\frac{9}{2}$.
(e) We have,
$ \begin{aligned} f(x)= & (x+1)^{3}(x-3)^{3} \\ f^{\prime}(x) & =3(x+1)^{2}(x-3)^{3}+3(x-3)^{2}(x+1)^{3} \\ & =3(x+1)^{2}(x-3)^{2}[x-3+x+1] \\ & =3(x+1)^{2}(x-3)^{2}(2 x-2) \\ & =6(x+1)^{2}(x-3)^{2}(x-1) \end{aligned} $
Now,
$f^{\prime}(x)=0 \Rightarrow x=-1,3,1$
The points $x=-1, x=1$, and $x=3$ divide the real line into four disjoint intervals
i.e., $(-\infty,-1),(-1,1),(1,3)$, and $(3, \infty)$.
In intervals $(-\infty,-1)$ and $(-1,1), f^{\prime}(x)=6(x+1)^{2}(x-3)^{2}(x-1)<0$.
$\therefore f$ is strictly decreasing in intervals $(-\infty,-1)$ and $(-1,1)$.
In intervals $(1,3)$ and $(3, \infty), f^{\prime}(x)=6(x+1)^{2}(x-3)^{2}(x-1)>0$.
$\therefore f$ is strictly increasing in intervals $(1,3)$ and $(3, \infty)$.
7. Show that $y=\log (1+x)-\frac{2 x}{2+x}, x>-1$, is an increasing function of $x$ throughout its domain.
Show Answer
Solution
We have,
$ \begin{aligned} & y=\log (1+x)-\frac{2 x}{2+x} \\ & \therefore \frac{d y}{d x}=\frac{1}{1+x}-\frac{(2+x)(2)-2 x(1)}{(2+x)^{2}}=\frac{1}{1+x}-\frac{4}{(2+x)^{2}}=\frac{x^{2}}{(2+x)^{2}} \end{aligned} $
Now, $\frac{d y}{d x}=0$
$\Rightarrow \frac{x^{2}}{(2+x)^{2}}=0$
$\Rightarrow x^{2}=0 \quad[(2+x) \neq 0$ as $x>-1]$
$\Rightarrow x=0$
Since $x>-1$, point $x=0$ divides the domain $(-1, \infty)$ in two disjoint intervals i.e., $-1<$ $x<0$ and $x>0$.
When $-1<x<0$, we have:
$x<0 \Rightarrow x^{2}>0$
$x>-1 \Rightarrow(2+x)>0 \Rightarrow(2+x)^{2}>0$
$\therefore y^{\prime}=\frac{x^{2}}{(2+x)^{2}}>0$
Also, when $x>0$ :
$x>0 \Rightarrow x^{2}>0,(2+x)^{2}>0$
$\therefore y^{\prime}=\frac{x^{2}}{(2+x)^{2}}>0$
Hence, function $f$ is increasing throughout this domain.
8. Find the values of $x$ for which $y=[x(x-2)]^{2}$ is an increasing function.
Show Answer
Solution
We have,
$y=[x(x-2)]^{2}=[x^{2}-2 x]^{2}$
$\therefore \frac{d y}{d x}=y^{\prime}=2(x^{2}-2 x)(2 x-2)=4 x(x-2)(x-1)$
$\therefore \frac{d y}{d x}=0 \Rightarrow x=0, x=2, x=1$.
The points $x=0, x=1$, and $x=2$ divide the real line into four disjoint intervals i.e., $(-\infty, 0),(0,1)(1,2)$, and $(2, \infty)$.
In intervals $(-\infty, 0)$ and $(1,2), \frac{d y}{d x}<0$.
$\therefore y$ is strictly decreasing in intervals $(-\infty, 0)$ and $(1,2)$.
However, in intervals $(0,1)$ and $(2, \infty), \frac{d y}{d x}>0$.
$\therefore y$ is strictly increasing in intervals $(0,1)$ and $(2, \infty)$.
$\therefore y$ is strictly increasing for $0<x<1$ and $x>2$.
9. Prove that $y=\frac{4 \sin \theta}{(2+\cos \theta)}-\theta$ is an increasing function of $\theta$ in $0, \frac{\pi}{2}$.
Show Answer
Solution
We have,
$ \begin{aligned} & y=\frac{4 \sin \theta}{(2+\cos \theta)}-\theta \\ & \begin{aligned} \therefore \frac{d y}{d x} & =\frac{(2+\cos \theta)(4 \cos \theta)-4 \sin \theta(-\sin \theta)}{(2+\cos \theta)^{2}}-1 \\ \quad & \frac{8 \cos \theta+4 \cos ^{2} \theta+4 \sin ^{2} \theta}{(2+\cos \theta)^{2}}-1 \\ \quad & \frac{8 \cos \theta+4}{(2+\cos \theta)^{2}}-1 \end{aligned} \end{aligned} $
Now, $\frac{d y}{d x}=0$.
$\Rightarrow \frac{8 \cos \theta+4}{(2+\cos \theta)^{2}}=1$
$\Rightarrow 8 \cos \theta+4=4+\cos ^{2} \theta+4 \cos \theta$
$\Rightarrow \cos ^{2} \theta-4 \cos \theta=0$
$\Rightarrow \cos \theta(\cos \theta-4)=0$
$\Rightarrow \cos \theta=0$ or $\cos \theta=4$
Since $\cos \theta \neq 4, \cos \theta=0$.
$\cos \theta=0 \Rightarrow \theta=\frac{\pi}{2}$
Now,
$\frac{d y}{d x}=\frac{8 \cos \theta+4-(4+\cos ^{2} \theta+4 \cos \theta)}{(2+\cos \theta)^{2}}=\frac{4 \cos \theta-\cos ^{2} \theta}{(2+\cos \theta)^{2}}=\frac{\cos \theta(4-\cos \theta)}{(2+\cos \theta)^{2}}$
In interval $(0, \frac{\pi}{2})$, we have $\cos \theta>0$. Also, $4>\cos \theta \Rightarrow 4-\cos \theta>0$.
$\therefore \cos \theta(4-\cos \theta)>0$ and also $(2+\cos \theta)^{2}>0$
$\Rightarrow \frac{\cos \theta(4-\cos \theta)}{(2+\cos \theta)^{2}}>0$
$\Rightarrow \frac{d y}{d x}>0$
Therefore, $y$ is strictly increasing in interval $(0, \frac{\pi}{2})$.
Also, the given function is continuous at $x=0$ and $x=\frac{\pi}{2}$.
Hence, $y$ is increasing in interval $[0, \frac{\pi}{2}]$.
10. Prove that the logarithmic function is increasing on $(0, \infty)$.
Show Answer
Solution
The given function is $f(x)=\log x$.
$\therefore f^{\prime}(x)=\frac{1}{x}$
It is clear that for $x>0, f^{\prime}(x)=\frac{1}{x}>0$.
Hence, $f(x)=\log x$ is strictly increasing in interval $(0, \infty)$.
11. Prove that the function $f$ given by $f(x)=x^{2}-x+1$ is neither strictly increasing nor decreasing on $(-1,1)$.
Show Answer
Solution
The given function is $f(x)=x^{2}-x+1$.
$\therefore f^{\prime}(x)=2 x-1$
Now, $f^{\prime}(x)=0 \Rightarrow x=\frac{1}{2}$.
The point $\frac{1}{2}$ divides the interval $(-1,1)$ into two disjoint intervals
i.e., $(-1, \frac{1}{2})$ and $(\frac{1}{2}, 1)$.
Now, in interval $(-1, \frac{1}{2}), f^{\prime}(x)=2 x-1<0$.
Therefore, $f$ is strictly decreasing in interval $(-1, \frac{1}{2})$.
However, in interval $(\frac{1}{2}, 1), f^{\prime}(x)=2 x-1>0$.
Therefore, $f$ is strictly increasing in interval $(\frac{1}{2}, 1)$.
Hence, $f$ is neither strictly increasing nor decreasing in interval $(-1,1)$.
12. Which of the following functions are decreasing on $0, \frac{\pi}{2}$ ?
(A) $\cos x$
(B) $\cos 2 x$
(C) $\cos 3 x$
(D) $\tan x$
Show Answer
Solution
(A) Let $f_1(x)=\cos x$.
$\therefore f_1^{\prime}(x)=-\sin x$
In interval $(0, \frac{\pi}{2}), f_1^{\prime}(x)=-\sin x<0$.
$\therefore f_1(x)=\cos x _{\text{is strictly decreasing in interval }}(0, \frac{\pi}{2})$.
(B) Let $f_2(x)=\cos 2 x$.
$\therefore f_2^{\prime}(x)=-2 \sin 2 x$
Now, $0<x<\frac{\pi}{2} \Rightarrow 0<2 x<\pi \Rightarrow \sin 2 x>0 \Rightarrow-2 \sin 2 x<0$
$\therefore f_2^{\prime}(x)=-2 \sin 2 x<0$ on $(0, \frac{\pi}{2})$
$\therefore f_2(x)=\cos 2 x$ is strictly decreasing in interval $(0, \frac{\pi}{2})$.
(C) Let $f_3(x)=\cos 3 x$.
$\therefore f_3^{\prime}(x)=-3 \sin 3 x$
Now, $f_3^{\prime}(x)=0$.
$\Rightarrow \sin 3 x=0 \Rightarrow 3 x=\pi$, as $x \in(0, \frac{\pi}{2})$
$\Rightarrow x=\frac{\pi}{3}$
The point $x=\frac{\pi}{3}$ divides the interval $(0, \frac{\pi}{2})$ into two disjoint intervals
i.e., $0(0, \frac{\pi}{3})$ and $(\frac{\pi}{3}, \frac{\pi}{2})$.
Now, in interval $(0, \frac{\pi}{3}), f_3(x)=-3 \sin 3 x<0[.$ as $.0<x<\frac{\pi}{3} \Rightarrow 0<3 x<\pi]$.
$\therefore f_3$ is strictly decreasing in interval $(0, \frac{\pi}{3})$.
However, in interval $(\frac{\pi}{3}, \frac{\pi}{2}), f_3(x)=-3 \sin 3 x>0[.$ as $.\frac{\pi}{3}<x<\frac{\pi}{2} \Rightarrow \pi<3 x<\frac{3 \pi}{2}]$. $\therefore f_3$ is strictly increasing in interval $(\frac{\pi}{3}, \frac{\pi}{2})$.
Hence, $f_3$ is neither increasing nor decreasing in interval $(0, \frac{\pi}{2})$.
(D) Let $f_4(x)=\tan x$.
$\therefore f_4^{\prime}(x)=\sec ^{2} x$
In interval $(0, \frac{\pi}{2}), f_4^{\prime}(x)=\sec ^{2} x>0$.
$\therefore f_4$ is strictly increasing in interval $(0, \frac{\pi}{2})$.
Therefore, functions $\cos x$ and $\cos 2 x$ are strictly decreasing in $(0, \frac{\pi}{2})$. Hence, the correct answers are A and B.
13. On which of the following intervals is the function $f$ given by $f(x)=x^{100}+\sin x-1$ decreasing?
(A) $(0,1)$
(B) $\frac{\pi}{2}, \pi$
(C) $0, \frac{\pi}{2}$
(D) None of these
Show Answer
Solution
We have, $f(x)=x^{100}+\sin x-1$
$\therefore f^{\prime}(x)=100 x^{99}+\cos x$
In interval $(0,1), \cos x>0$ and $100 x^{99}>0$.
$\therefore f^{\prime}(x)>0$.
Thus, function $f$ is strictly increasing in interval $(0,1)$.
In interval $(\frac{\pi}{2}, \pi), \cos x<0$ and $100 x^{99}>0$. Also, $100 x^{99}>\cos x$.
$\therefore f^{\prime}(x)>0$ in $(\frac{\pi}{2}, \pi)$.
Thus, function $f$ is strictly increasing in interval $(\frac{\pi}{2}, \pi)$.
In interval $(0, \frac{\pi}{2}), \cos x>0$ and $100 x^{99}>0$.
$\therefore 100 x^{99}+\cos x>0$
$\Rightarrow f^{\prime}(x)>0$ on $(0, \frac{\pi}{2})$
$\therefore f$ is strictly increasing in interval $(0, \frac{\pi}{2})$.
Hence, function $f$ is strictly decreasing in none of the intervals.
The correct answer is D.
14. For what values of $a$ the function $f$ given by $f(x)=x^{2}+a x+1$ is increasing on $[1,2]$ ?
Show Answer
Solution
We have, $f(x)=x^{2}+a x+1$
$\therefore f^{\prime}(x)=2 x+a$
Now, function $f$ will be increasing in $(1,2)$, if $f^{\prime}(x)>0$ in $(1,2)$.
$f^{\prime}(x)>0$
$\Rightarrow 2 x+a>0$
$\Rightarrow 2 x>-a$
$\Rightarrow \quad x>\frac{-a}{2}$
Therefore, we have to find the least value of $a$ such that
$x>\frac{-a}{2}$, when $x \in(1,2)$.
$\Rightarrow x>\frac{-a}{2}($ when $1<x<2)$
Thus, the least value of $a$ for $f$ to be increasing on $(1,2)$ is given by,
$\frac{-a}{2}=1$
$\frac{-a}{2}=1 \Rightarrow a=-2$
Hence, the required value of $a$ is -2 .
15. Let $I$ be any interval disjoint from $[-1,1]$. Prove that the function $f$ given by $f(x)=x+\frac{1}{x}$ is increasing on $I$.
Show Answer
Solution
We have,
$f(x)=x+\frac{1}{x}$
$\therefore f^{\prime}(x)=1-\frac{1}{x^{2}}$
Now,
$f^{\prime}(x)=0 \Rightarrow \frac{1}{x^{2}}=1 \Rightarrow x= \pm 1$
The points $x=1$ and $x=-1$ divide the real line in three disjoint intervals i.e.,
$(-\infty,-1),(-1,1)$, and $(1, \infty)$.
In interval $(-1,1)$, it is observed that:
$-1<x<1$
$\Rightarrow x^{2}<1$
$\Rightarrow 1<\frac{1}{x^{2}}, x \neq 0$
$\Rightarrow 1-\frac{1}{x^{2}}<0, x \neq 0$
$\therefore f^{\prime}(x)=1-\frac{1}{x^{2}}<0$ on $(-1,1) \sim{0}$.
$\therefore f$ is strictly decreasing on $(-1,1) \sim{0}$.
In intervals $(-\infty,-1)$ and $(1, \infty)$, it is observed that: $x<-1$ or $1<x$
$\Rightarrow x^{2}>1$
$\Rightarrow 1>\frac{1}{x^{2}}$
$\Rightarrow 1-\frac{1}{x^{2}}>0$
$\therefore f^{\prime}(x)=1-\frac{1}{x^{2}}>0$ on $(-\infty,-1)$ and $(1, \infty)$.
$\therefore f$ is strictly increasing on $(-\infty, 1)$ and $(1, \infty)$.
Hence, function $f$ is strictly increasing in interval $\mathbf{I}$ disjoint from $(-1,1)$. Hence, the given result is proved.
16. Prove that the function $f$ given by $f(x)=\log \sin x$ is increasing on $0, \frac{\pi}{2}$ and decreasing on $\frac{\pi}{2}, \pi$
Show Answer
Solution
We have,
$f(x)=\log \sin x$
$\therefore f^{\prime}(x)=\frac{1}{\sin x} \cos x=\cot x$
In interval $(0, \frac{\pi}{2}), f^{\prime}(x)=\cot x>0$.
$\therefore f$ is strictly increasing in $(0, \frac{\pi}{2})$.
In interval $(\frac{\pi}{2}, \pi), f^{\prime}(x)=\cot x<0$.
$\therefore f$ is strictly decreasing in $(\frac{\pi}{2}, \pi)$.
17. Prove that the function $f$ given by $f(x)=\log |\cos x|$ is decreasing on $(0, \frac{\pi}{2})$ and increasing on $(\frac{3 \pi}{2}, 2 \pi)$.
Show Answer
Solution
We have,
$f(x)=\log \cos x$
$\therefore f^{\prime}(x)=\frac{1}{\cos x}(-\sin x)=-\tan x$
In interval $(0, \frac{\pi}{2}), \tan x>0 \Rightarrow-\tan x<0$.
$\therefore f^{\prime}(x)<0$ on $(0, \frac{\pi}{2})$
$\therefore f$ is strictly decreasing on $(0, \frac{\pi}{2})$.
In interval $(\frac{\pi}{2}, \pi), \tan x<0 \Rightarrow-\tan x>0$.
$\therefore f^{\prime}(x)>0$ on $(\frac{\pi}{2}, \pi)$ $\therefore f$ is strictly increasing on $(\frac{\pi}{2}, \pi)$.
18. Prove that the function given by $f(x)=x^{3}-3 x^{2}+3 x-100$ is increasing in $\mathbf{R}$.
Show Answer
Solution
We have,
$f(x)=x^{3}-3 x^{2}+3 x-100$
$ \begin{aligned} f^{\prime}(x) & =3 x^{2}-6 x+3 \\ & =3(x^{2}-2 x+1) \\ & =3(x-1)^{2} \end{aligned} $
For any $x \in \mathbf{R},(x-1)^{2}>0$.
Thus, $f^{\prime}(x)$ is always positive in $\mathbf{R}$.
Hence, the given function $(f)$ is increasing in $\mathbf{R}$.
19. The interval in which $y=x^{2} e^{-x}$ is increasing is
(A) $(-\infty, \infty)$
(B) $(-2,0)$
(C) $(2, \infty)$
(D) $(0,2)$
Show Answer
Solution
We have,
$y=x^{2} e^{-x}$ $\therefore \frac{d y}{d x}=2 x e^{-x}-x^{2} e^{-x}=x e^{-x}(2-x)$
Now, $\frac{d y}{d x}=0$.
$\Rightarrow x=0$ and $x=2$
The points $x=0$ and $x=2$ divide the real line into three disjoint intervals
i.e., $(-\infty, 0),(0,2)$, and $(2, \infty)$.
In intervals $(-\infty, 0)$ and $(2, \infty), f^{\prime}(x)<0$ as $e^{-x}$ is always positive.
$\therefore f$ is decreasing on $(-\infty, 0)$ and $(2, \infty)$.
In interval $(0,2), f^{\prime}(x)>0$.
$\therefore f$ is strictly increasing on $(0,2)$.
Hence, $f$ is strictly increasing in interval $(0,2)$.
The correct answer is D.
6.4 Maxima and Minima
In this section, we will use the concept of derivatives to calculate the maximum or minimum values of various functions. In fact, we will find the ’turning points’ of the graph of a function and thus find points at which the graph reaches its highest (or lowest) locally. The knowledge of such points is very useful in sketching the graph of a given function. Further, we will also find the absolute maximum and absolute minimum of a function that are necessary for the solution of many applied problems.
Let us consider the following problems that arise in day to day life.
(i) The profit from a grove of orange trees is given by $P(x)=a x+b x^{2}$, where $a, b$ are constants and $x$ is the number of orange trees per acre. How many trees per acre will maximise the profit?
(ii) A ball, thrown into the air from a building 60 metres high, travels along a path given by $h(x)=60+x-\frac{x^{2}}{60}$, where $x$ is the horizontal distance from the building and $h(x)$ is the height of the ball . What is the maximum height the ball will reach?
(iii) An Apache helicopter of enemy is flying along the path given by the curve $f(x)=x^{2}+7$. A soldier, placed at the point $(1,2)$, wants to shoot the helicopter when it is nearest to him. What is the nearest .distance?
In each of the above problem, there is something common, i.e., we wish to find out the maximum or minimum values of the given functions. In order to tackle such problems, we first formally define maximum or minimum values of a function, points of local maxima and minima and test for determining such points.
Definition 3 Let $f$ be a function defined on an interval I. Then
(a) $f$ is said to have a maximum value in I, if there exists a point $c$ in I such that $f(c)>f(x)$, for all $x \in I$.
The number $f(c)$ is called the maximum value of $f$ in I and the point $c$ is called a point of maximum value of $f$ in $I$.
(b) $f$ is said to have a minimum value in $I$, if there exists a point $c$ in $I$ such that $f(c)<f(x)$, for all $x \in I$.
The number $f(c)$, in this case, is called the minimum value of $f$ in I and the point $c$, in this case, is called a point of minimum value of $f$ in $I$.
(c) $f$ is said to have an extreme value in $I$ if there exists a point $c$ in I such that $f(c)$ is either a maximum value or a minimum value of $f$ in $I$.
The number $f(c)$, in this case, is called an extreme value of $f$ in $I$ and the point $c$ is called an extreme point.
Remark In Fig 6.7(a), (b) and (c), we have exhibited that graphs of certain particular functions help us to find maximum value and minimum value at a point. Infact, through graphs, we can even find maximum/minimum value of a function at a point at which it is not even differentiable (Example 15 ).

Fig 6.7
Example 14 Find the maximum and the minimum values, if any, of the function $f$ given by $ f(x)=x^{2}, x \in \mathbf{R} . $
Solution From the graph of the given function (Fig 6.8), we have $f(x)=0$ if $x=0$. Also $ f(x) \geq 0 \text{, for all } x \in \mathbf{R} \text{. } $
Therefore, the minimum value of $f$ is 0 and the point of minimum value of $f$ is $x=0$. Further, it may be observed from the graph of the function that $f$ has no maximum value and hence no point of maximum value of $f$ in $\mathbf{R}$.
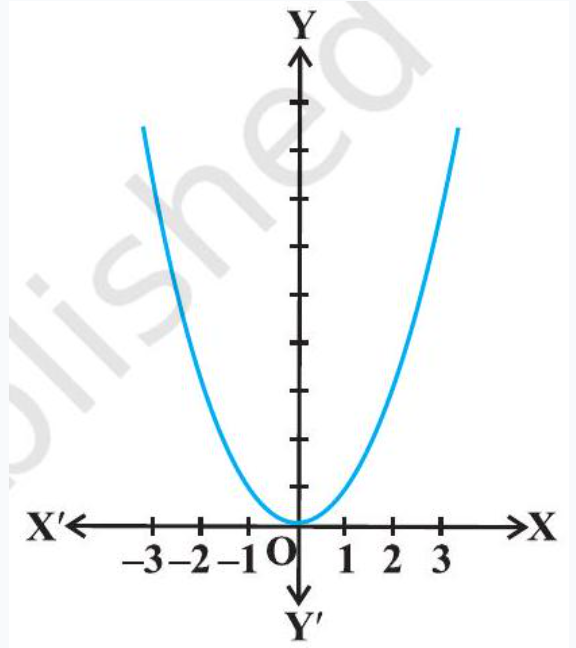
Fig 6.8
Note If we restrict the domain of $f$ to $[-2,1]$ only, then $f$ will have maximum value $(-2)^{2}=4$ at $x=-2$.
Example 15 Find the maximum and minimum values of $f$, if any, of the function given by $f(x)=|x|, x \in \mathbf{R}$.
Solution From the graph of the given function (Fig 6.9), note that
$$ f(x) \geq 0 \text{, for all } x \in \mathbf{R} \text{ and } f(x)=0 \text{ if } x=0 \text{. } $$
Therefore, the function $f$ has a minimum value 0 and the point of minimum value of $f$ is $x=0$. Also, the graph clearly shows that $f$ has no maximum value in $\mathbf{R}$ and hence no point of maximum value in $\mathbf{R}$.
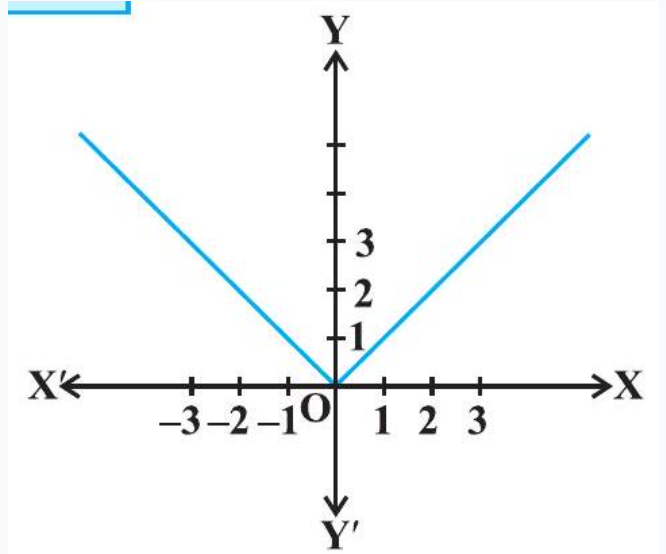
Fig 6.9
Note
(i) If we restrict the domain of $f$ to $[-2,1]$ only, then $f$ will have maximum value $|-2|=2$.
(ii) One may note that the function $f$ in Example 27 is not differentiable at $x=0$.
Example 16 Find the maximum and the minimum values, if any, of the function given by $ f(x)=x, x \in(0,1) . $
Solution The given function is an increasing (strictly) function in the given interval $(0,1)$. From the graph (Fig 6.10) of the function $f$, it seems that, it should have the minimum value at a point closest to 0 on its right and the maximum value at a point closest to 1 on its left. Are such points available? Of course, not. It is not possible to locate such points. Infact, if a point $x_0$ is closest to 0 , then we find $\frac{x_0}{2}<x_0$ for all $x_0 \in(0,1)$. Also, if $x_1$ is closest to 1 , then $\frac{x_1+1}{2}>x_1$ for all $x_1 \in(0,1)$.
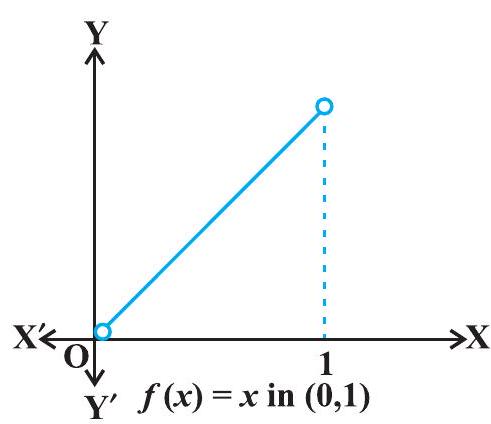
Therefore, the given function has neither the Fig 6.10 maximum value nor the minimum value in the interval $(0,1)$.
Remark The reader may observe that in Example 16 **, if we include the points 0 and 1 in the domain of $f$, i.e., if we extend the domain of $f$ to $[0,1]$, then the function $f$ has minimum value 0 at $x=0$ and maximum value 1 at $x=1$. Infact, we have the following results (The proof of these results are beyond the scope of the present text)
Every monotonic function assumes its maximum/minimum value at the end points of the domain of definition of the function.
A more general result is Every continuous function on a closed interval has a maximum and a minimum value.
Note By a monotonic function $f$ in an interval $I$, we mean that $f$ is either increasing in I or decreasing in I.
Maximum and minimum values of a function defined on a closed interval will be discussed later in this section.
Let us now examine the graph of a function as shown in Fig 6.11. Observe that at points $A, B, C$ and $D$ on the graph, the function changes its nature from decreasing to increasing or vice-versa. These points may be called turning points of the given function. Further, observe that at turning points, the graph has either a little hill or a little valley. Roughly speaking, the function has minimum value in some neighbourhood (interval) of each of the points $A$ and $C$ which are at the bottom of their respective valleys. Similarly, the function has maximum value in some neighbourhood of points B and $D$ which are at the top of their respective hills. For this reason, the points $A$ and $C$ may be regarded as points of local minimum value (or relative minimum value) and points $B$ and $D$ may be regarded as points of local maximum value (or relative maximum value) for the function. The local maximum value and local minimum value of the function are referred to as local maxima and local minima, respectively, of the function.
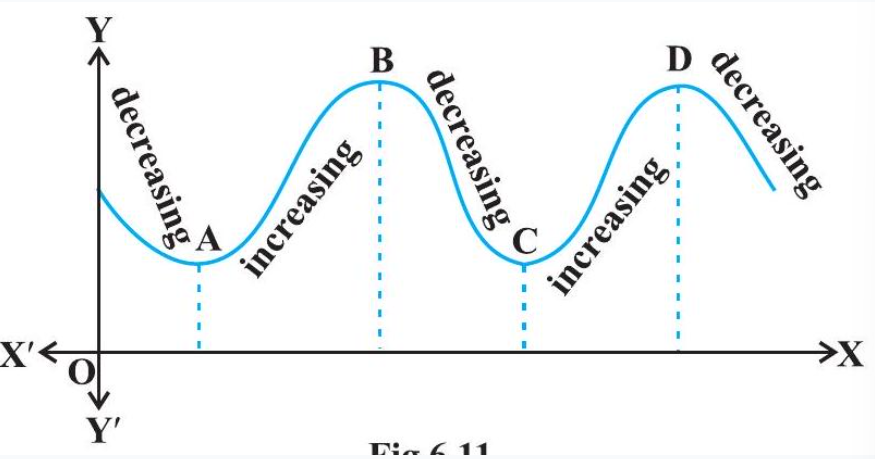
Fig 6.11
We now formally give the following definition
Definition 4 Let $f$ be a real valued function and let $c$ be an interior point in the domain of $f$. Then
(a) $c$ is called a point of local maxima if there is an $h>0$ such that
$ f(c) \geq f(x) \text{, for all } x \text{ in }(c-h, c+h), x \neq c $
The value $f(c)$ is called the local maximum value of $f$.
(b) $c$ is called a point of local minima if there is an $h>0$ such that
$ f(c) \leq f(x) \text{, for all } x \text{ in }(c-h, c+h) $
The value $f(c)$ is called the local minimum value of $f$.
Geometrically, the above definition states that if $x=c$ is a point of local maxima of $f$, then the graph of $f$ around $c$ will be as shown in Fig 6.12(a). Note that the function $f$ is increasing (i.e., $.f^{\prime}(x)>0)$ in the interval $(c-h, c)$ and decreasing (i.e., $.f^{\prime}(x)<0)$ in the interval $(c, c+h)$.
This suggests that $f^{\prime}(c)$ must be zero.
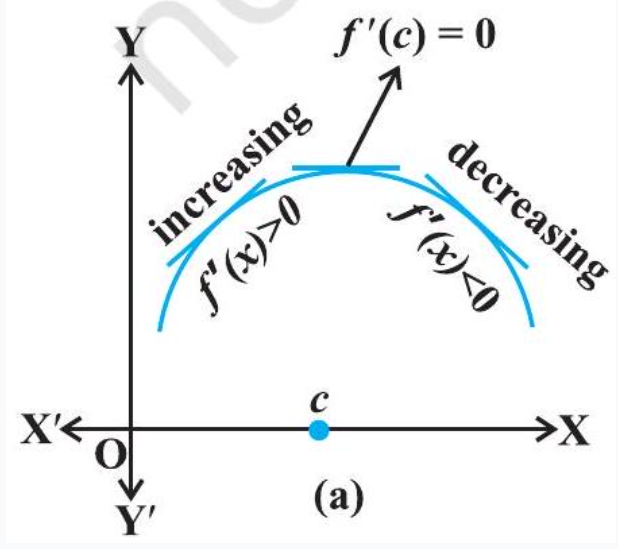
Fig 6.12
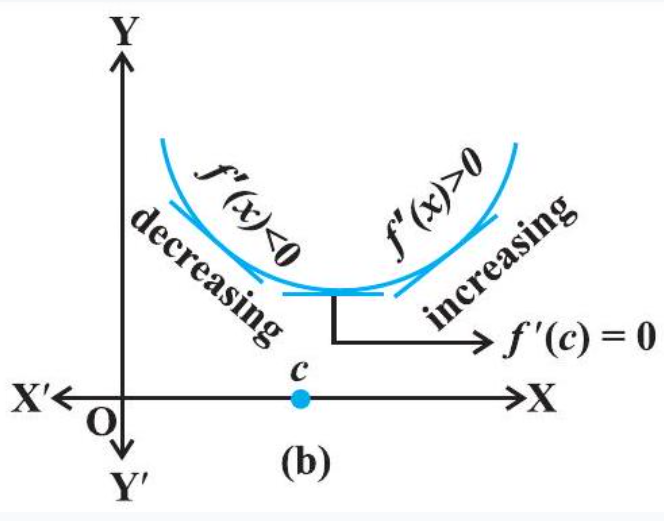
Similarly, if $c$ is a point of local minima of $f$, then the graph of $f$ around $c$ will be as shown in Fig 6.14(b). Here $f$ is decreasing (i.e., $.f^{\prime}(x)<0)$ in the interval $(c-h, c)$ and increasing (i.e., $.f^{\prime}(x)>0)$ in the interval $(c, c+h)$. This again suggest that $f^{\prime}(c)$ must be zero.
The above discussion lead us to the following theorem (without proof).
Theorem 2 Let $f$ be a function defined on an open interval I. Suppose $c \in I$ be any point. If $f$ has a local maxima or a local minima at $x=c$, then either $f^{\prime}(c)=0$ or $f$ is not differentiable at $c$.
Remark The converse of above theorem need not be true, that is, a point at which the derivative vanishes need not be a point of local maxima or local minima. For example, if $f(x)=x^{3}$, then $f^{\prime}(x)=3 x^{2}$ and so $f^{\prime}(0)=0$. But 0 is neither a point of local maxima nor a point of local minima (Fig 6.13).
Note A point $c$ in the domain of a function $f$ at which either $f^{\prime}(c)=0$ or $f$ is not differentiable is called a critical point of $f$. Note that if $f$ is continuous at $c$ and $f^{\prime}(c)=0$, then there exists an $h>0$ such that $f$ is differentiable in the interval $(c-h, c+h)$.
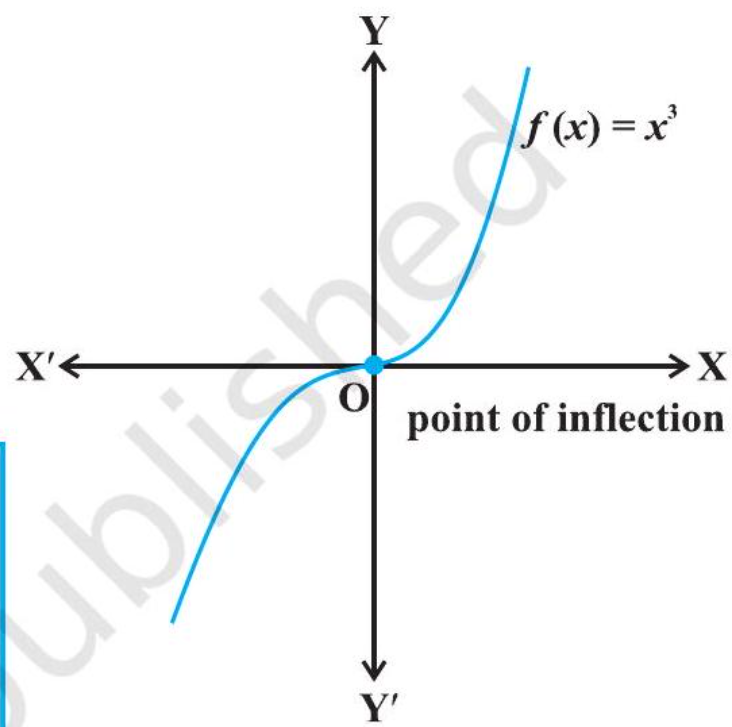
Fig 6.13
We shall now give a working rule for finding points of local maxima or points of local minima using only the first order derivatives.
Theorem 3 (First Derivative Test) Let $f$ be a function defined on an open interval I. Let $f$ be continuous at a critical point $c$ in I. Then
(i) If $f^{\prime}(x)$ changes sign from positive to negative as $x$ increases through $c$, i.e., if $f^{\prime}(x)>0$ at every point sufficiently close to and to the left of $c$, and $f^{\prime}(x)<0$ at every point sufficiently close to and to the right of $c$, then $c$ is a point of local maxima.
(ii) If $f^{\prime}(x)$ changes sign from negative to positive as $x$ increases through $c$, i.e., if $f^{\prime}(x)<0$ at every point sufficiently close to and to the left of $c$, and $f^{\prime}(x)>0$ at every point sufficiently close to and to the right of $c$, then $c$ is a point of local minima.
(iii) If $f^{\prime}(x)$ does not change sign as $x$ increases through $c$, then $c$ is neither a point of local maxima nor a point of local minima. Infact, such a point is called point of inflection (Fig 6.13).
Note If $c$ is a point of local maxima of $f$, then $f(c)$ is a local maximum value of $f$. Similarly, if $c$ is a point of local minima of $f$, then $f(c)$ is a local minimum value of $f$.
Figures 6.13 and 6.14, geometrically explain Theorem 3.
Fig 6.14
Example 17 Find all points of local maxima and local minima of the function $f$ given by $f(x)=x^{3}-3 x+3$.
Solution We have
$$ \begin{array}{lrl} & f(x) & =x^3-3 x+3 \\ \text{ or } & f^{\prime}(x) & =3 x^2-3=3(x-1)(x+1) \\ \text{ or } & f^{\prime}(x) &=0 \text{ at } x=1 \text{ and } x=-1 \end{array} $$
Thus, $x= \pm 1$ are the only critical points which could possibly be the points of local maxima and/or local minima of $f$. Let us first examine the point $x=1$.
Note that for values close to 1 and to the right of $1, f^{\prime}(x)>0$ and for values close to 1 and to the left of $1, f^{\prime}(x)<0$. Therefore, by first derivative test, $x=1$ is a point of local minima and local minimum value is $f(1)=1$. In the case of $x=-1$, note that $f^{\prime}(x)>0$, for values close to and to the left of -1 and $f^{\prime}(x)<0$, for values close to and to the right of -1 . Therefore, by first derivative test, $x=-1$ is a point of local maxima and local maximum value is $f(-1)=5$.
$ \begin{array}{|c|c|c|} \hline & \text{ Values of } x & \text{ Sign of } f^{\prime}(x)=3(x-1)(x+1) \\ \hline {\begin{array}{l} \text{ Close to 1 } \end{array}} & \text{ to the right (say 1.1 etc.)} & > 0 \\ \hline & \text{ to the left (say 0.9 etc.) } & <0 \\ \hline \text{ Close to - 1 } & \text{ to the right (say -0.9 etc.) } & <0 \\ \hline & \text{ to the left (say -1.1 etc.) } & >0 \\ \hline \end{array} $
Example 18 Find all the points of local maxima and local minima of the function $f$ given by
Solution We have
$$ \begin{array}{lrl} & f(x) & =2 x^{3}-6 x^{2}+6 x+5 \\ \text{ or }& f^{\prime}(x) & =6 x^{2}-12 x+6=6(x-1)^{2} \\ \text{ or }& f^{\prime}(x) & =0 \quad \text{ at } \quad x=1 \end{array} $$
Thus, $x=1$ is the only critical point of $f$. We shall now examine this point for local maxima and/or local minima of $f$. Observe that $f^{\prime}(x) \geq 0$, for all $x \in \mathbf{R}$ and in particular $f^{\prime}(x)>0$, for values close to 1 and to the left and to the right of 1 . Therefore, by first derivative test, the point $x=1$ is neither a point of local maxima nor a point of local minima. Hence $x=1$ is a point of inflexion.
Remark One may note that since $f^{\prime}(x)$, in Example 30 , never changes its sign on $\mathbf{R}$, graph of $f$ has no turning points and hence no point of local maxima or local minima.
We shall now give another test to examine local maxima and local minima of a given function. This test is often easier to apply than the first derivative test.
Theorem 4 (Second Derivative Test) Let $f$ be a function defined on an interval I and $c \in I$. Let $f$ be twice differentiable at $c$. Then
(i) $x=c$ is a point of local maxima if $f^{\prime}(c)=0$ and $f^{\prime \prime}(c)<0$
The value $f(c)$ is local maximum value of $f$.
(ii) $x=c$ is a point of local minima if $f^{\prime}(c)=0$ and $f^{\prime \prime}(c)>0$
In this case, $f(c)$ is local minimum value of $f$.
(iii) The test fails if $f^{\prime}(c)=0$ and $f^{\prime \prime}(c)=0$.
In this case, we go back to the first derivative test and find whether $c$ is a point of local maxima, local minima or a point of inflexion.
Note As $f$ is twice differentiable at $c$, we mean second order derivative of $f$ exists at $c$.
Example 19 Find local minimum value of the function $f$ given by $f(x)=3+|x|, x \in \mathbf{R}$.
Solution Note that the given function is not differentiable at $x=0$. So, second derivative test fails. Let us try first derivative test. Note that 0 is a critical point of $f$. Now to the left of $0, f(x)=3-x$ and so $f^{\prime}(x)=-1<0$. Also to
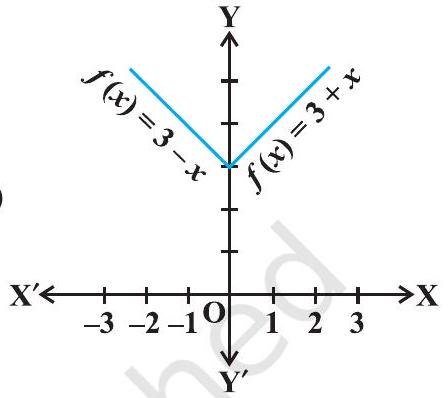
Fig 6.15 the right of $0, f(x)=3+x$ and so $f^{\prime}(x)=1>0$. Therefore, by first derivative test, $x=$ 0 is a point of local minima of $f$ and local minimum value of $f$ is $f(0)=3$.
Example 20 Find local maximum and local minimum values of the function $f$ given by
$ f(x)=3 x^{4}+4 x^{3}-12 x^{2}+12 $
Solution We have
$f(x)=3 x^{4}+4 x^{3}-12 x^{2}+12$
or & $f^{\prime}(x)=12 x^{3}+12 x^{2}-24 x=12 x(x-1)(x+2)$
or & $f^{\prime}(x)=0$ at $x=0, x=1$ and $x=-2$.
Now & $f^{\prime \prime}(x)=36 x^{2}+24 x-24=12(3 x^{2}+2 x-2)$
$$ \left\{\begin{array}{c} f^{\prime \prime}(0)=-24<0 \\ f^{\prime \prime}(1)=36>0 \\ f^{\prime \prime}(-2)=72>0 \end{array}\right. $$
Therefore, by second derivative test, $x=0$ is a point of local maxima and local maximum value of $f$ at $x=0$ is $f(0)=12$ while $x=1$ and $x=-2$ are the points of local minima and local minimum values of $f$ at $x=-1$ and -2 are $f(1)=7$ and $f(-2)=-20$, respectively.
Example 21 Find all the points of local maxima and local minima of the function $f$ given by f(x)=2 x^{3}-6 x^{2}+6 x+5
Solution We have
$$ \text{or} \qquad \left\{\begin{array}{1}& f(x)=2 x^{3}-6 x^{2}+6 x+5 \\ \end{array}\right. $$
$$ \begin{aligned} & \left\{\begin{array}{l} f^{\prime}(x)=6 x^{2}-12 x+6=6(x-1)^{2} \\ f^{\prime \prime}(x)=12(x-1) \end{array}\right. \end{aligned} $$
Now $f^{\prime}(x)=0$ gives $x=1$. Also $f^{\prime \prime}(1)=0$. Therefore, the second derivative test fails in this case. So, we shall go back to the first derivative test.
We have already seen (Example 18 ) that, using first derivative test, $x=1$ is neither a point of local maxima nor a point of local minima and so it is a point of inflexion.
Example 22 Find two positive numbers whose sum is 15 and the sum of whose squares is minimum.
Solution Let one of the numbers be $x$. Then the other number is $(15-x)$. Let $S(x)$ denote the sum of the squares of these numbers. Then or
$$ S(x)=x^{2}+(15-x)^{2}=2 x^{2}-30 x+225 $$
$$ \begin{gathered} \left\{\begin{array}{l} S^{\prime}(x)=4 x-30 \\ S^{\prime \prime}(x)=4 \end{array}\right. \end{gathered} $$
Now $S^{\prime}(x)=0$ gives $x=\frac{15}{2}$. Also $S^{\prime \prime}(\frac{15}{2})=4>0$. Therefore, by second derivative test, $x=\frac{15}{2}$ is the point of local minima of S. Hence the sum of squares of numbers is minimum when the numbers are $\frac{15}{2}$ and $15-\frac{15}{2}=\frac{15}{2}$.
Remark Proceeding as in Example 34 one may prove that the two positive numbers, whose sum is $k$ and the sum of whose squares is minimum, are $\frac{k}{2}$ and $\frac{k}{2}$.
Example 23 Find the shortest distance of the point $(0, c)$ from the parabola $y=x^{2}$, where $\frac{1}{2} \leq c \leq 5$.
Solution Let $(h, k)$ be any point on the parabola $y=x^{2}$. Let $D$ be the required distance between $(h, k)$ and $(0, c)$. Then
$$ \begin{equation*} \mathrm{D}=\sqrt{(h-0)^{2}+(k-c)^{2}}=\sqrt{h^{2}+(k-c)^{2}} \tag{1} \end{equation*} $$
Since $(h, k)$ lies on the parabola $y=x^{2}$, we have $k=h^{2}$. So (1) gives
$$ \mathrm{D} \equiv \mathrm{D}(k)=\sqrt{k+(k-c)^{2}} $$
or $\mathrm{D}^{\prime}(k)=\frac{1+2(k-c)}{\sqrt{k+(k-c)^{2}}}$
Now $\mathrm{D}^{\prime}(k)=0 \text { से } k=\frac{2 c-1}{2}$
Observe that when $k<\frac{2 c-1}{2}$, then $2(k-c)+1<0$, i.e., $D^{\prime}(k)<0$. Also when $k>\frac{2 c-1}{2}$, then $D^{\prime}(k)>0$. So, by first derivative test, $D(k)$ is minimum at $k=\frac{2 c-1}{2}$.
Hence, the required shortest distance is given by
$$ \mathrm{D}\left(\frac{2 c-1}{2}\right)=\sqrt{\frac{2 c-1}{2}+\left(\frac{2 c-1}{2}-c\right)^{2}}=\frac{\sqrt{4 c-1}}{2} \text { } $$
Note The reader may note that in Example 35 , we have used first derivative test instead of the second derivative test as the former is easy and short.
Example 24 Let $AP$ and $BQ$ be two vertical poles at points $A$ and $B$, respectively. If $AP=16 m, BQ=22 m$ and $A B=20 m$, then find the distance of a point $R$ on $A B$ from the point $A$ such that $R P^{2}+R Q^{2}$ is minimum.
Solution Let $R$ be a point on $AB$ such that $AR=x m$. Then $RB=(20-x) m($ as $AB=20 m)$. From Fig 6.16, we have
$$ \begin{aligned} & \mathrm{RP}^{2}=\mathrm{AR}^{2}+\mathrm{AP}^{2} \\ \end{aligned} $$
and $R Q^{2}=R B^{2}+B Q^{2}$

Fig 6.16
Therefore $ \mathrm{RP}^{2}+\mathrm{RQ}^{2}=\mathrm{AR}^{2}+\mathrm{AP}^{2}+\mathrm{RB}^{2}+\mathrm{BQ}^{2}$ $$ \begin{aligned} & =x^{2}+(16)^{2}+(20-x)^{2}+(22)^{2} \\ & =2 x^{2}-40 x+1140 \end{aligned} $$
Let $S \equiv S(x)=RP^{2}+RQ^{2}=2 x^{2}-40 x+1140$
Therefore $S^{\prime}(x)=4 x-40$
Now $S^{\prime}(x)=0$ gives $x=10$. Also $S^{\prime \prime}(x)=4>0$, for all $x$ and so $S^{\prime \prime}(10)>0$. Therefore, by second derivative test, $x=10$ is the point of local minima of $S$. Thus, the distance of $R$ from $A$ on $AB$ is $AR=x=10 m$.
Example 25 If length of three sides of a trapezium other than base are equal to $10 cm$, then find the area of the trapezium when it is maximum.
Solution The required trapezium is as given in Fig 6.17. Draw perpendiculars DP and $CQ$ on $AB$. Let $AP=x cm$. Note that $\triangle APD \sim \triangle BQC$. Therefore, $QB=x cm$. Also, by Pythagoras theorem, $DP=QC=\sqrt{100-x^{2}}$. Let $A$ be the area of the trapezium. Then
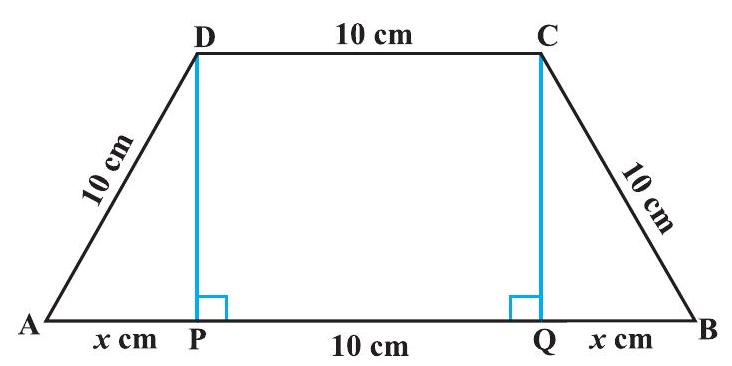
Fig 6.17
$$ \begin{aligned} \mathrm{A} & \equiv \mathrm{A}(x) \\ & =\frac{1}{2} \text { (sum of parallel sides) (height) } \\ & =\frac{1}{2}(2 x+10+10)\left(\sqrt{100-x^{2}}\right) \\ & =(x+10)\left(\sqrt{100-x^{2}}\right) \end{aligned} $$
or
$$ \begin{aligned} \mathrm{A}^{\prime}(x) & =(x+10) \frac{(-2 x)}{\sqrt{100-x^{2}}}+\left(\sqrt{100-x^{2}}\right) \\ & =\frac{-2 x^{2}-10 x+100}{\sqrt{100-x^{2}}} \end{aligned} $$
Now $\quad A^{\prime}(x)=0$ gives $2 x^{2}+10 x-100=0$, i.e., $x=5$ and $x=-10$.
Since $x$ represents distance, it can not be negative. So, $x=5$. Now
$$ \begin{aligned} A^{\prime \prime}(x) & =\frac{\sqrt{100-x^{2}}(-4 x-10)-(-2 x^{2}-10 x+100) \frac{(-2 x)}{2 \sqrt{100-x^{2}}}}{100-x^{2}} \\ & =\frac{2 x^{3}-300 x-1000}{(100-x^{2})^{\frac{3}{2}}} \text{ (on simplification) } \\ A^{\prime \prime}(5) & =\frac{2(5)^{3}-300(5)-1000}{(100-(5)^{2})^{\frac{3}{2}}}=\frac{-2250}{75 \sqrt{75}}=\frac{-30}{\sqrt{75}}<0 \end{aligned} $$
Thus, area of trapezium is maximum at $x=5$ and the area is given by
$$ A(5)=(5+10) \sqrt{100-(5)^{2}}=15 \sqrt{75}=75 \sqrt{3} cm^{2} $$
Example 26 Prove that the radius of the right circular cylinder of greatest curved surface area which can be inscribed in a given cone is half of that of the cone.
Solution Let $OC=r$ be the radius of the cone and $OA=h$ be its height. Let a cylinder with radius $OE=x$ inscribed in the given cone (Fig 6.18). The height $QE$ of the cylinder is given by
$ \begin{array}{llll} \quad & \frac{QE}{OA} & =\frac{EC}{OC} \quad(\text{ since } \Delta QEC \sim \Delta AOC) \\ \text{ or } & \frac{QE}{h} & =\frac{r-x}{r} \\ \text{ or } & QE & =\frac{h(r-x)}{r} \end{array} $
Let $S$ be the curved surface area of the given cylinder. Then
$$ \begin{aligned} & S \equiv S(x)=\frac{2 \pi x h(r-x)}{r}=\frac{2 \pi h}{r}\left(r x-x^{2}\right) \\ & \left\{\begin{array}{l} S^{\prime}(x)=\frac{2 \pi h}{r}(r-2 x) \\ S^{\prime \prime}(x)=\frac{-4 \pi h}{r} \end{array}\right. \end{aligned} $$

Now $S^{\prime}(x)=0$ gives $x=\frac{r}{2}$. Since $S^{\prime \prime}(x)<0$ for all $x, S^{\prime \prime}(\frac{r}{2})<0$. So $x=\frac{r}{2}$ is a point of maxima of S. Hence, the radius of the cylinder of greatest curved surface area which can be inscribed in a given cone is half of that of the cone.
6.4.1 Maximum and Minimum Values of a Function in a Closed Interval
Let us consider a function $f$ given by $f(x)=x+2, x \in(0,1)$
Observe that the function is continuous on $(0,1)$ and neither has a maximum value nor has a minimum value. Further, we may note that the function even has neither a local maximum value nor a local minimum value.
However, if we extend the domain of $f$ to the closed interval $[0,1]$, then $f$ still may not have a local maximum (minimum) values but it certainly does have maximum value $3=f(1)$ and minimum value $2=f(0)$. The maximum value 3 of $f$ at $x=1$ is called absolute maximum value (global maximum or greatest value) of $f$ on the interval $[0,1]$. Similarly, the minimum value 2 of $f$ at $x=0$ is called the absolute minimum value (global minimum or least value) of $f$ on $[0,1]$.
Consider the graph given in Fig 6.19 of a continuous function defined on a closed interval $[a, d]$. Observe that the function $f$ has a local minima at $x=b$ and local
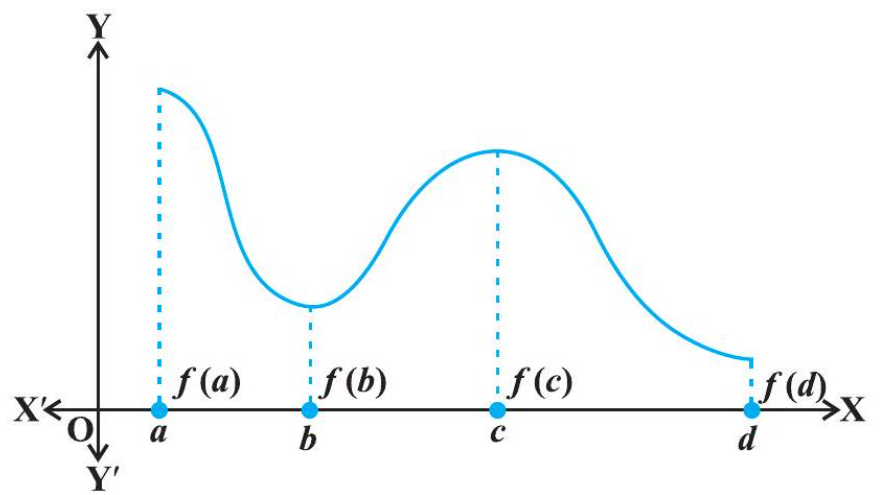 Fig 6.19
Fig 6.19
minimum value is $f(b)$. The function also has a local maxima at $x=c$ and local maximum value is $f(c)$.
Also from the graph, it is evident that $f$ has absolute maximum value $f(a)$ and absolute minimum value $f(d)$. Further note that the absolute maximum (minimum) value of $f$ is different from local maximum (minimum) value of $f$.
We will now state two results (without proof) regarding absolute maximum and absolute minimum values of a function on a closed interval I.
Theorem 5 Let $f$ be a continuous function on an interval $I=[a, b]$. Then $f$ has the absolute maximum value and $f$ attains it at least once in I. Also, $f$ has the absolute minimum value and attains it at least once in $I$.
Theorem 6 Let $f$ be a differentiable function on a closed interval I and let $c$ be any interior point of I. Then
(i) $f^{\prime}(c)=0$ if $f$ attains its absolute maximum value at $c$.
(ii) $f^{\prime}(c)=0$ if $f$ attains its absolute minimum value at $c$.
In view of the above results, we have the following working rule for finding absolute maximum and/or absolute minimum values of a function in a given closed interval $[a, b]$.
Working Rule
Step 1: Find all critical points of $f$ in the interval, i.e., find points $x$ where either $f^{\prime}(x)=0$ or $f$ is not differentiable.
Step 2: Take the end points of the interval.
Step 3: At all these points (listed in Step 1 and 2), calculate the values of $f$.
Step 4: Identify the maximum and minimum values of $f$ out of the values calculated in Step 3. This maximum value will be the absolute maximum (greatest) value of $f$ and the minimum value will be the absolute minimum (least) value of $f$.
Example 27 Find the absolute maximum and minimum values of a function $f$ given by $f(x)=2 x^{3}-15 x^{2}+36 x+1 \text{ on the interval }[1,5] .$
Solution We have
$$ \begin{aligned} f(x) & =2 x^{3}-15 x^{2}+36 x+1 \end{aligned} $$ $ \begin{aligned} or \qquad f^{\prime}(x) & =6 x^{2}-30 x+36=6(x-3)(x-2) \end{aligned} $
Note that $f^{\prime}(x)=0$ gives $x=2$ and $x=3$.
We shall now evaluate the value of $f$ at these points and at the end points of the interval $[1,5]$, i.e., at $x=1, x=2, x=3$ and at $x=5$. So
$$ \begin{aligned} & f(1)=2\left(1^{3}\right)-15\left(1^{2}\right)+36(1)+1=24 \\ & f(2)=2\left(2^{3}\right)-15\left(2^{2}\right)+36(2)+1=29 \\ & f(3)=2\left(3^{3}\right)-15\left(3^{2}\right)+36(3)+1=28 \\ & f(5)=2\left(5^{3}\right)-15\left(5^{2}\right)+36(5)+1=56 \end{aligned} $$
Thus, we conclude that absolute maximum value of $f$ on $[1,5]$ is 56 , occurring at $x=5$, and absolute minimum value of $f$ on $[1,5]$ is 24 which occurs at $x=1$.
Example 28 Find absolute maximum and minimum values of a function $f$ given by $f(x)=12 x^{\frac{4}{3}}-6 x^{\frac{1}{3}}, x \in[-1,1]$
Solution We have
$$ \begin{aligned} & f(x)=12 x^{\frac{4}{3}}-6 x^{\frac{1}{3}} \end{aligned} $$ $ \begin{aligned} or \qquad & f^{\prime}(x)=16 x^{\frac{1}{3}}-\frac{2}{x^{\frac{2}{3}}}=\frac{2(8 x-1)}{x^{\frac{2}{3}}} \end{aligned} $
Thus, $f^{\prime}(x)=0$ gives $x=\frac{1}{8}$. Further note that $f^{\prime}(x)$ is not defined at $x=0$. So the critical points are $x=0$ and $x=\frac{1}{8}$. Now evaluating the value of $f$ at critical points $x=0, \frac{1}{8}$ and at end points of the interval $x=-1$ and $x=1$, we have
$$ \begin{aligned} f(-1) & =12\left(-1^{\frac{4}{3}}\right)-6\left(-1^{\frac{1}{3}}\right)=18 \\ f(0) & =12(0)-6(0)=0 \\ f\left(\frac{1}{8}\right) & =12\left(\frac{1}{8}\right)^{\frac{4}{3}}-6\left(\frac{1}{8}\right)^{\frac{1}{3}}=\frac{-9}{4} \\ f(1) & =12\left(1^{\frac{4}{3}}\right)-6\left(1^{\frac{1}{3}}\right)=6 \end{aligned} $$
Hence, we conclude that absolute maximum value of $f$ is 18 that occurs at $x=-1$ and absolute minimum value of $f$ is $\frac{-9}{4}$ that occurs at $x=\frac{1}{8}$.
Example 29 An Apache helicopter of enemy is flying along the curve given by $y=x^{2}+7$. A soldier, placed at $(3,7)$, wants to shoot down the helicopter when it is nearest to him. Find the nearest distance.
Solution For each value of $x$, the helicopter’s position is at point $(x, x^{2}+7)$. Therefore, the distance between the helicopter and the soldier placed at $(3,7)$ is
$ \sqrt{(x-3)^{2}+(x^{2}+7-7)^{2}} \text{, i.e., } \sqrt{(x-3)^{2}+x^{4}} \text{. } $
$ Let \qquad f(x)=(x-3)^{2}+x^{4} $
$ or \qquad f^{\prime}(x)=2(x-3)+4 x^{3}=2(x-1)\left(2 x^{2}+2 x+3\right) $
Thus, $f^{\prime}(x)=0$ gives $x=1$ or $2 x^{2}+2 x+3=0$ for which there are no real roots. Also, there are no end points of the interval to be added to the set for which $f^{\prime}$ is zero, i.e., there is only one point, namely, $x=1$. The value of $f$ at this point is given by $f(1)=(1-3)^{2}+(1)^{4}=5$. Thus, the distance between the solider and the helicopter is $\sqrt{f(1)}=\sqrt{5}$.
Note that $\sqrt{5}$ is either a maximum value or a minimum value. Since
$$ \sqrt{f(0)}=\sqrt{(0-3)^{2}+(0)^{4}}=3>\sqrt{5} \text { } $$
it follows that $\sqrt{5}$ is the minimum value of $\sqrt{f(x)}$. Hence, $\sqrt{5}$ is the minimum distance between the soldier and the helicopter.
EXERCISE 6.3
1. Find the maximum and minimum values, if any, of the following functions given by
(i) $f(x)=(2 x-1)^{2}+3$
(ii) $f(x)=9 x^{2}+12 x+2$
(iii) $f(x)=-(x-1)^{2}+10$
(iv) $g(x)=x^{3}+1$
Show Answer
Solution
(i) The given function is $f(x)=(2 x-1)^{2}+3$.
It can be observed that $(2 x-1)^{2} \geq 0$ for every $x \square \mathbf{R}$.
Therefore, $f(x)=(2 x-1)^{2}+3 \geq 3$ for every $x \square \mathbf{R}$.
The minimum value of $f$ is attained when $2 x-1=0$.
$2 x-1=0$
$ x=\frac{1}{2} $
$\square$ Minimum value of $f=f(\frac{1}{2})=(2 \cdot \frac{1}{2}-1)^{2}+3=3$
Hence, function $f$ does not have a maximum value.
(ii) The given function is $f(x)=9 x^{2}+12 x+2=(3 x+2)^{2}-2$.
It can be observed that $(3 x+2)^{2} \geq 0$ for every $x \square \mathbf{R}$.
Therefore, $f(x)=(3 x+2)^{2}-2 \geq-2$ for every $x \square \mathbf{R}$.
The minimum value of $f$ is attained when $3 x+2=0$.
$3 x+2=0 \square^{x=\frac{-2}{3}}$
$\square$ Minimum value of $f=f(-\frac{2}{3})=(3(\frac{-2}{3})+2)^{2}-2=-2$
Hence, function $f$ does not have a maximum value.
(iii) The given function is $f(x)=-(x-1)^{2}+10$.
It can be observed that $(x-1)^{2} \geq 0$ for every $x \square \mathbf{R}$.
Therefore, $f(x)=-(x-1)^{2}+10 \leq 10$ for every $x \square \mathbf{R}$.
The maximum value of $f$ is attained when $(x-1)=0$.
$(x-1)=0 \square x=0$
$\square$ Maximum value of $f=f(1)=-(1-1)^{2}+10=10$
Hence, function $f$ does not have a minimum value.
(iv) The given function is $g(x)=x^{3}+1$.
Hence, function $g$ neither has a maximum value nor a minimum value.
2. Find the maximum and minimum values, if any, of the following functions given by
(i) $f(x)=|x+2|-1$
(ii) $g(x)=-|x+1|+3$
(iii) $h(x)=\sin (2 x)+5$
(iv) $f(x)=|\sin 4 x+3|$
(v) $h(x)=x+1, x \in(-1,1)$
Show Answer
Solution
(i) $f(x)=|x+2|-1$
We know that $|x+2| \geq 0$ for every $x \square \mathbf{R}$.
Therefore, $f(x)=|x+2|-1 \geq-1$ for every $x \square \mathbf{R}$.
The minimum value of $f$ is attained when $|x+2|=0$.
$|x+2|=0$
$\Rightarrow x=-2$
$\square$ Minimum value of $f=f(-2)=|-2+2|-1=-1$
Hence, function $f$ does not have a maximum value.
(ii) $g(x)=-|x+1|+3$
We know that $-|x+1| \leq 0$ for every $x \square \mathbf{R}$.
Therefore, $g(x)=-|x+1|+3 \leq 3$ for every $x \square \mathbf{R}$.
The maximum value of $g$ is attained when $|x+1|=0$.
$|x+1|=0$
$\Rightarrow x=-1$
$\square$ Maximum value of $g=g(-1)=-|-1+1|+3=3$
Hence, function $g$ does not have a minimum value. (iii) $h(x)=\sin 2 x+5$
We know that $-1 \leq \sin 2 x \leq 1$.
$\square-1+5 \leq \sin 2 x+5 \leq 1+5$
$\square 4 \leq \sin 2 x+5 \leq 6$
Hence, the maximum and minimum values of $h$ are 6 and 4 respectively.
(iv) $f(x)=|\sin 4 x+3|$
We know that $-1 \leq \sin 4 x \leq 1$.
$\square 2 \leq \sin 4 x+3 \leq 4$
$\square 2 \leq^{|\sin 4 x+3|} \leq 4$
Hence, the maximum and minimum values of $f$ are 4 and 2 respectively.
(v) $h(x)=x+1, x \square(-1,1)$
Here, if a point $x_0$ is closest to -1 , then we find $\frac{x_0}{2}+1<x_0+1$ for all $x_0 \square(-1,1)$.
Also, if $x_1$ is closest to 1 , then $x_1+1<\frac{x_1+1}{2}+1$ for all $x_1 \square(-1,1)$.
Hence, function $h(x)$ has neither maximum nor minimum value in $(-1,1)$.
3. Find the local maxima and local minima, if any, of the following functions. Find also the local maximum and the local minimum values, as the case may be:
(i) $f(x)=x^{2}$
(ii) $g(x)=x^{3}-3 x$
(iii) $h(x)=\sin x+\cos x, 0<x<\frac{\pi}{2}$
(iv) $f(x)=\sin x-\cos x, 0<x<2 \pi$
(v) $f(x)=x^{3}-6 x^{2}+9 x+15 \quad$ (vi) $g(x)=\frac{x}{2}+\frac{2}{x}, x>0$
(vii) $g(x)=\frac{1}{x^{2}+2}$
(viii) $f(x)=x \sqrt{1-x}, 0<x<1$
Show Answer
Solution
(i) $f(x)=x^{2}$
$\therefore f^{\prime}(x)=2 x$
Now,
$f^{\prime}(x)=0 \Rightarrow x=0$
Thus, $x=0$ is the only critical point which could possibly be the point of local maxima or local minima of $f$.
We have $f^{\prime \prime}(0)=2$, which is positive.
Therefore, by second derivative test, $x=0$ is a point of local minima and local minimum value of $f$ at $x=0$ is $f(0)=0$.
(ii) $g(x)=x^{3}-3 x$
$\therefore g^{\prime}(x)=3 x^{2}-3$
Now,
$g^{\prime}(x)=0 \Rightarrow 3 x^{2}=3 \Rightarrow x= \pm 1$
$g^{\prime}(x)=6 x$
$g^{\prime}(1)=6>0$
$g^{\prime}(-1)=-6<0$
By second derivative test, $x=1$ is a point of local minima and local minimum value of $g$ at $x=1$ is $g(1)=1^{3}-3=1-3=-2$. However, $x=-1$ is a point of local maxima and local maximum value of $g$ at $x=-1$ is $g(1)=(-1)^{3}-3(-1)=-1+3=2$.
(iii) $h(x)=\sin x+\cos x, 0<x<\frac{\pi}{2}$
$\therefore h^{\prime}(x)=\cos x-\sin x$
$h^{\prime}(x)=0 \Rightarrow \sin x=\cos x \Rightarrow \tan x=1 \Rightarrow x=\frac{\pi}{4} \in(0, \frac{\pi}{2})$
$h^{\prime \prime}(x)=-\sin x-\cos x=-(\sin x+\cos x)$
$h^{\prime \prime}(\frac{\pi}{4})=-(\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}})=-\frac{2}{\sqrt{2}}=-\sqrt{2}<0$
Therefore, by second derivative test, $x=\frac{\pi}{4}$ is a point of local maxima and the local
maximum value of $h$ at $x=\frac{\pi}{4}$ is $h(\frac{\pi}{4})=\sin \frac{\pi}{4}+\cos \frac{\pi}{4}=\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}=\sqrt{2}$.
(iv) $f(x)=\sin x-\cos x, 0<x<2 \pi$
$\therefore f^{\prime}(x)=\cos x+\sin x$
$f^{\prime}(x)=0 \Rightarrow \cos x=-\sin x \Rightarrow \tan x=-1 \Rightarrow x=\frac{3 \pi}{4}, \frac{7 \pi}{4} \in(0,2 \pi)$
$f^{\prime \prime}(x)=-\sin x+\cos x$
$f^{\prime \prime}(\frac{3 \pi}{4})=-\sin \frac{3 \pi}{4}+\cos \frac{3 \pi}{4}=-\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}=-\sqrt{2}>0$
$f^{\prime \prime}(\frac{7 \pi}{4})=-\sin \frac{7 \pi}{4}+\cos \frac{7 \pi}{4}=\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}=\sqrt{2}>0$
Therefore, by second derivative test, $x=\frac{3 \pi}{4}$ is a point of local maxima and the local
maximum value of $f$ at $x=\frac{3 \pi}{4}$ is
$f(\frac{3 \pi}{4})=\sin \frac{3 \pi}{4}-\cos \frac{3 \pi}{4}=\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}=\sqrt{2}$ However, $x=\frac{7 \pi}{4}$ is a point of local minima and
the local minimum value of $f$ at $x=\frac{7 \pi}{4}$ is $f(\frac{7 \pi}{4})=\sin \frac{7 \pi}{4}-\cos \frac{7 \pi}{4}=-\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}=-\sqrt{2}$.
(v) $f(x)=x^{3}-6 x^{2}+9 x+15$
$\therefore f^{\prime}(x)=3 x^{2}-12 x+9$
$f^{\prime}(x)=0 \Rightarrow 3(x^{2}-4 x+3)=0$
$\Rightarrow 3(x-1)(x-3)=0$
$\Rightarrow x=1,3$
Now, $f^{\prime \prime}$
$(x)=6 x-12=6(x-2)$
$f^{\prime \prime}(1)=6(1-2)=-6<0$
$f^{\prime \prime}(3)=6(3-2)=6>0$
Therefore, by second derivative test, $x=1$ is a point of local maxima and the local maximum value of $f$ at $x=1$ is $f(1)=1-6+9+15=19$. However, $x=3$ is a point of local minima and the local minimum value of $f$ at $x=3$ is $f(3)=27-54+27+15=$ 15.
(vi) $g(x)=\frac{x}{2}+\frac{2}{x}, x>0$
$\therefore g^{\prime}(x)=\frac{1}{2}-\frac{2}{x^{2}}$
Now,
$g^{\prime}(x)=0$ gives $\frac{2}{x^{2}}=\frac{1}{2} \Rightarrow x^{2}=4 \Rightarrow x= \pm 2$
Since $x>0$, we take $x=2$.
Now,
$g^{\prime \prime}(x)=\frac{4}{x^{3}}$
$g^{\prime \prime}(2)=\frac{4}{2^{3}}=\frac{1}{2}>0$
Therefore, by second derivative test, $x=2$ is a point of local minima and the local
minimum value of $g$ at $x=2$ is $g(2)=\frac{2}{2}+\frac{2}{2}=1+1=2$.
(vii) $g(x)=\frac{1}{x^{2}+2}$
$\therefore g^{\prime}(x)=\frac{-(2 x)}{(x^{2}+2)^{2}}$
$g^{\prime}(x)=0 \Rightarrow \frac{-2 x}{(x^{2}+2)^{2}}=0 \Rightarrow x=0$
Now, for values close to $x=0$ and to the left of $0, g^{\prime}(x)>0$. Also, for values close to $x=$ 0 and to the right of $0, g^{\prime}(x)<0$.
Therefore, by first derivative test, $x=0$ is a point of local maxima and the local maximum value of $g(0)$ is $\frac{1}{0+2}=\frac{1}{2}$.
(viii) $f(x)=x \sqrt{1-x}, x>0$
$\therefore f^{\prime}(x)=\sqrt{1-x}+x \cdot \frac{1}{2 \sqrt{1-x}}(-1)=\sqrt{1-x}-\frac{x}{2 \sqrt{1-x}}$
$=\frac{2(1-x)-x}{2 \sqrt{1-x}}=\frac{2-3 x}{2 \sqrt{1-x}}$
$f^{\prime}(x)=0 \Rightarrow \frac{2-3 x}{2 \sqrt{1-x}}=0 \Rightarrow 2-3 x=0 \Rightarrow x=\frac{2}{3}$
$f^{\prime \prime}(x)=\frac{1}{2}[\frac{\sqrt{1-x}(-3)-(2-3 x)(\frac{-1}{2 \sqrt{1-x}})}{1-x}]$
$=\frac{\sqrt{1-x}(-3)+(2-3 x)(\frac{1}{2 \sqrt{1-x}})}{2(1-x)}$
$=\frac{-6(1-x)+(2-3 x)}{4(1-x)^{\frac{3}{2}}}$
$=\frac{3 x-4}{4(1-x)^{\frac{3}{2}}}$
$f^{\prime \prime}(\frac{2}{3})=\frac{3(\frac{2}{3})-4}{4(1-\frac{2}{3})^{\frac{3}{2}}}=\frac{2-4}{4(\frac{1}{3})^{\frac{3}{2}}}=\frac{-1}{2(\frac{1}{3})^{\frac{3}{2}}}<0$
Therefore, by second derivative test, $x=\frac{2}{3}$ is a point of local maxima and the local
maximum value of $f$ at $x=\frac{2}{3}$ is $f(\frac{2}{3})=\frac{2}{3} \sqrt{1-\frac{2}{3}}=\frac{2}{3} \sqrt{\frac{1}{3}}=\frac{2}{3 \sqrt{3}}=\frac{2 \sqrt{3}}{9}$.
4. Prove that the following functions do not have maxima or minima:
(i) $f(x)=e^{x}$
(ii) $g(x)=\log x$
(iii) $h(x)=x^{3}+x^{2}+x+1$
Show Answer
Solution
i. We have,
$f(x)=e^{x}$
$\therefore f^{\prime}(x)=e^{x}$
Now, if $f^{\prime}(x)=0$, then $e^{x}=0$. But, the exponential function can never assume 0 for any value of $x$.
Therefore, there does not exist $c \square \mathbf{R}$ such that $f^{\prime}(c)=0$.
Hence, function $f$ does not have maxima or minima.
ii. We have,
$g(x)=\log x$
$\therefore g^{\prime}(x)=\frac{1}{x}$
Since $\log x$ is defined for a positive number $x, g^{\prime}(x)>0$ for any $x$.
Therefore, there does not exist $c \square \mathbf{R}$ such that $g^{\prime}(c)=0$.
Hence, function $g$ does not have maxima or minima.
iii. We have,
$h(x)=x^{3}+x^{2}+x+1$
$\therefore h^{\prime}(x)=3 x^{2}+2 x+1$
Now,
$h(x)=0 \square 3 x^{2}+2 x+1=0 \square x=\frac{-2 \pm 2 \sqrt{2} i}{6}=\frac{-1 \pm \sqrt{2} i}{3} \notin \mathbf{R}$
Therefore, there does not exist $c \square \mathbf{R}$ such that $h^{\prime}(c)=0$.
Hence, function $h$ does not have maxima or minima.
5. Find the absolute maximum value and the absolute minimum value of the following functions in the given intervals:
(i) $f(x)=x^{3}, x \in[-2,2]$
(ii) $f(x)=\sin x+\cos x, x \in[0, \pi]$
(iii) $f(x)=4 x-\frac{1}{2} x^{2}, x \in[-2, \frac{9}{2}]$
(iv) $.f(x)=(x-1)^{2}+3, x \in-3,1]$
Show Answer
Solution
(i) The given function is $f(x)=x^{3}$.
$\therefore f^{\prime}(x)=3 x^{2}$
Now,
$f^{\prime}(x)=0 \Rightarrow x=0$
Then, we evaluate the value of $f$ at critical point $x=0$ and at end points of the interval $[-2,2]$.
$f(0)=0$
$f(-2)=(-2)^{3}=-8$
$f(2)=(2)^{3}=8$
Hence, we can conclude that the absolute maximum value of $f$ on $[-2,2]$ is 8 occurring at $x=2$. Also, the absolute minimum value of $f$ on $[-2,2]$ is -8 occurring at $x=-2$.
(ii) The given function is $f(x)=\sin x+\cos x$.
$\therefore f^{\prime}(x)=\cos x-\sin x$
Now,
$f^{\prime}(x)=0 \Rightarrow \sin x=\cos x \Rightarrow \tan x=1 \Rightarrow x=\frac{\pi}{4}$
Then, we evaluate the value of $f$ at critical point $x=\frac{\pi}{4}$ and at the end points of the interval $[0, \pi]$.
6. Find the maximum profit that a company can make, if the profit function is given by
$ p(x)=41-72 x-18 x^{2} $
Show Answer
Solution
The profit function is given as $p(x)=41-24 x-18 x^{2}$.
$\therefore p^{\prime}(x)=-24-36 x$
$p^{\prime \prime}(x)=-36$
Now,
$p^{\prime}(x)=0 \Rightarrow x=\frac{-24}{36}=-\frac{2}{3}$
Also,
$p^{\prime \prime}(\frac{-2}{3})=-36<0$
By second derivative test, $x=-\frac{2}{3}$ is the point of local maxima of $p$.
$\therefore$ Maximum profit $=p(-\frac{2}{3})$
$ \begin{aligned} & =41-24(-\frac{2}{3})-18(-\frac{2}{3})^{2} \\ & =41+16-8 \\ & =49 \end{aligned} $
Hence, the maximum profit that the company can make is 49 units.
7. Find both the maximum value and the minimum value of $3 x^{4}-8 x^{3}+12 x^{2}-48 x+25$ on the interval $[0,3]$.
Show Answer
Solution
$f(x)=3 x^4-8 x^3+12 x^2-48 x+25$
$f^{\prime}(x)=0$ for finding critical points
$f^{\prime}(x)=12 x^3-24 x^2+24 x-48$
$12 x^3-24 x^2+24 x-48=0$
$12 x^2(x-2)+24(x-2)=0$
$\left(12 x^2+24\right)(x-2)=0$
Since $12 x^2+24 \neq 0$ and $x-2=0 \Longrightarrow x=2$
We need to check at $x=0,2,3$ $f(0)=25$
$f(2)=-39$
$f(3)=16$
$\therefore$ Maximum of $f(x)$ at $x=0$ is 25
Minimum of $f(x)$ at $x=2$ is -39
8. At what points in the interval $[0,2 \pi]$, does the function $\sin 2 x$ attain its maximum value?
Show Answer
Solution
Let $f(x)=\sin 2 x$.
$\therefore f^{\prime}(x)=2 \cos 2 x$
Now,
$ \begin{aligned} & f^{\prime}(x)=0 \Rightarrow \cos 2 x=0 \\ & \Rightarrow 2 x=\frac{\pi}{2}, \frac{3 \pi}{2}, \frac{5 \pi}{2}, \frac{7 \pi}{2} \\ & \Rightarrow x=\frac{\pi}{4}, \frac{3 \pi}{4}, \frac{5 \pi}{4}, \frac{7 \pi}{4} \end{aligned} $
Then, we evaluate the values of $f$ at critical points $x=\frac{\pi}{4}, \frac{3 \pi}{4}, \frac{5 \pi}{4}, \frac{7 \pi}{4}$ and at the end points of the interval $[0,2 \pi]$.
$f(\frac{\pi}{4})=\sin \frac{\pi}{2}=1, f(\frac{3 \pi}{4})=\sin \frac{3 \pi}{2}=-1$
$f(\frac{5 \pi}{4})=\sin \frac{5 \pi}{2}=1, f(\frac{7 \pi}{4})=\sin \frac{7 \pi}{2}=-1$
$f(0)=\sin 0=0, f(2 \pi)=\sin 2 \pi=0$
Hence, we can conclude that the absolute maximum value of $f$ on $[0,2 \pi]$ is occurring
at $x=\frac{\pi}{4}$ and $\quad x=\frac{5 \pi}{4}$.
9. What is the maximum value of the function $\sin x+\cos x$ ?
Show Answer
Solution
Let $f(x)=\sin x+\cos x$.
$\therefore f^{\prime}(x)=\cos x-\sin x$
$f^{\prime}(x)=0 \Rightarrow \sin x=\cos x \Rightarrow \tan x=1 \Rightarrow x=\frac{\pi}{4}, \frac{5 \pi}{4} \ldots$,
$f^{\prime \prime}(x)=-\sin x-\cos x=-(\sin x+\cos x)$
Now, $f^{\prime \prime}(x)$ will be negative when $(\sin x+\cos x)$ is positive i.e., when $\sin x$ and $\cos x$ are both positive. Also, we know that $\sin x$ and $\cos x$ both are positive in the first
quadrant. Then, $f^{\prime \prime}(x)$ will be negative when $x \in(0, \frac{\pi}{2})$.
Thus, we consider $x=\frac{\pi}{4}$.
$f^{\prime \prime}(\frac{\pi}{4})=-(\sin \frac{\pi}{4}+\cos \frac{\pi}{4})=-(\frac{2}{\sqrt{2}})=-\sqrt{2}<0$ $\square$ By second derivative test, $f$ will be the maximum at $x=\frac{\pi}{4}$ and the maximum value of $f$ is $f(\frac{\pi}{4})=\sin \frac{\pi}{4}+\cos \frac{\pi}{4}=\frac{1}{\sqrt{2}} \times \frac{1}{\sqrt{2}}=\frac{2}{\sqrt{2}}=\sqrt{2}$.
10. Find the maximum value of $2 x^{3}-24 x+107$ in the interval $[1,3]$. Find the maximum value of the same function in $[-3,-1]$.
Show Answer
Solution
Let $f(x)=2 x^{3}-24 x+107$.
$\therefore f^{\prime}(x)=6 x^{2}-24=6(x^{2}-4)$
Now,
$f^{\prime}(x)=0 \Rightarrow 6(x^{2}-4)=0 \Rightarrow x^{2}=4 \Rightarrow x= \pm 2$
We first consider the interval $[1,3]$.
Then, we evaluate the value of $f$ at the critical point $x=2 \square[1,3]$ and at the end points of the interval $[1,3]$.
$f(2)=2(8)-24(2)+107=16-48+107=75$
$f(1)=2(1)-24(1)+107=2-24+107=85$
$f(3)=2(27)-24(3)+107=54-72+107=89$
Hence, the absolute maximum value of $f(x)$ in the interval $[1,3]$ is 89 occurring at $x=$ 3.
Next, we consider the interval $[-3,-1]$.
Evaluate the value of $f$ at the critical point $x=-2 \square[-3,-1]$ and at the end points of the interval $[1,3]$.
$f(-3)=2(-27)-24(-3)+107=-54+72+107=125$
$f(-1)=2(-1)-24(-1)+107=-2+24+107=129$
$f(-2)=2(-8)-24(-2)+107=-16+48+107=139$
Hence, the absolute maximum value of $f(x)$ in the interval $[-3,-1]$ is 139 occurring at $x$ $=-2$.
11. It is given that at $x=1$, the function $x^{4}-62 x^{2}+a x+9$ attains its maximum value, on the interval $[0,2]$. Find the value of $a$.
Show Answer
Solution
Let $f(x)=x^{4}-62 x^{2}+a x+9$.
$\therefore f^{\prime}(x)=4 x^{3}-124 x+a$
It is given that function $f$ attains its maximum value on the interval $[0,2]$ at $x=1$.
$\therefore f^{\prime}(1)=0$
$\Rightarrow 4-124+a=0$
$\Rightarrow a=120$
Hence, the value of $a$ is 120 .
12. Find the maximum and minimum values of $x+\sin 2 x$ on $[0,2 \pi]$.
Show Answer
Solution
Let $f(x)=x+\sin 2 x$.
$\therefore f^{\prime}(x)=1+2 \cos 2 x$
Now, $f^{\prime}(x)=0 \Rightarrow \cos 2 x=-\frac{1}{2}=-\cos \frac{\pi}{3}=\cos (\pi-\frac{\pi}{3})=\cos \frac{2 \pi}{3}$
$2 x=2 \pi \pm \frac{2 \pi}{3} \quad n \in \mathbf{Z}$
$\Rightarrow x=n \pi \pm \frac{\pi}{3}, n \in \mathbf{Z}$
$\Rightarrow x=\frac{\pi}{3}, \frac{2 \pi}{3}, \frac{4 \pi}{3}, \frac{5 \pi}{3} \in[0,2 \pi]$
Then, we evaluate the value of $f$ at critical points $x=\frac{\pi}{3}, \frac{2 \pi}{3}, \frac{4 \pi}{3}, \frac{5 \pi}{3}$ and at the end points of the interval $[0,2 \pi]$. $f(\frac{\pi}{3})=\frac{\pi}{3}+\sin \frac{2 \pi}{3}=\frac{\pi}{3}+\frac{\sqrt{3}}{2}$
$f(\frac{2 \pi}{3})=\frac{2 \pi}{3}+\sin \frac{4 \pi}{3}=\frac{2 \pi}{3}-\frac{\sqrt{3}}{2}$
$f(\frac{4 \pi}{3})=\frac{4 \pi}{3}+\sin \frac{8 \pi}{3}=\frac{4 \pi}{3}+\frac{\sqrt{3}}{2}$
$f(\frac{5 \pi}{3})=\frac{5 \pi}{3}+\sin \frac{10 \pi}{3}=\frac{5 \pi}{3}-\frac{\sqrt{3}}{2}$
$f(0)=0+\sin 0=0$
$f(2 \pi)=2 \pi+\sin 4 \pi=2 \pi+0=2 \pi$
Hence, we can conclude that the absolute maximum value of $f(x)$ in the interval $[0,2 \pi]$ is $2 \pi$ occurring at $x=2 \pi$ and the absolute minimum value of $f(x)$ in the interval $[0,2 \pi]$ is 0 occurring at $x=0$.
13. Find two numbers whose sum is 24 and whose product is as large as possible.
Show Answer
Solution
Let one number be $x$. Then, the other number is $(24-x)$.
Let $P(x)$ denote the product of the two numbers. Thus, we have:
$ \begin{aligned} & P(x)=x(24-x)=24 x-x^{2} \\ & \therefore P^{\prime}(x)=24-2 x \\ & P^{\prime \prime}(x)=-2 \end{aligned} $
Now,
$P^{\prime}(x)=0 \Rightarrow x=12$
Also,
$P^{\prime \prime}(12)=-2<0$
$\square$ By second derivative test, $x=12$ is the point of local maxima of $P$. Hence, the product of the numbers is the maximum when the numbers are 12 and $24-12=12$.
14. Find two positive numbers $x$ and $y$ such that $x+y=60$ and $x y^{3}$ is maximum.
Show Answer
Solution
The two numbers are $x$ and $y$ such that $x+y=60$.
$\square y=60-x$
Let $f(x)=x y^{3}$.
$\Rightarrow f(x)=x(60-x)^{3}$
$\therefore f^{\prime}(x)=(60-x)^{3}-3 x(60-x)^{2}$
$=(60-x)^{2}[60-x-3 x]$
$=(60-x)^{2}(60-4 x)$
And, $f^{\prime \prime}(x)=-2(60-x)(60-4 x)-4(60-x)^{2}$
$ \begin{aligned} & =-2(60-x)[60-4 x+2(60-x)] \\ & =-2(60-x)(180-6 x) \\ & =-12(60-x)(30-x) \end{aligned} $
Now, $f^{\prime}(x)=0 \Rightarrow x=60$ or $x=15$
When $x=60, f^{\prime \prime}(x)=0$.
When $x=15, f^{\prime \prime}(x)=-12(60-15)(30-15)=-12 \times 45 \times 15<0$.
$\square$ By second derivative test, $x=15$ is a point of local maxima of $f$. Thus, function $x y^{3}$ is maximum when $x=15$ and $y=60-15=45$.
Hence, the required numbers are 15 and 45 .
15. Find two positive numbers $x$ and $y$ such that their sum is 35 and the product $x^{2} y^{5}$ is a maximum.
Show Answer
Solution
Let one number be $x$. Then, the other number is $y=(35-x)$.
Let $P(x)=x^{2} y^{5}$. Then, we have:
$ \begin{aligned} & P(x)=x^{2}(35-x)^{5} \\ & \begin{aligned} \therefore P^{\prime}(x) & =2 x(35-x)^{5}-5 x^{2}(35-x)^{4} \\ & =x(35-x)^{4}[2(35-x)-5 x] \\ & =x(35-x)^{4}(70-7 x) \\ & =7 x(35-x)^{4}(10-x) \end{aligned} \end{aligned} $
And, $P^{\prime \prime}(x)=7(35-x)^{4}(10-x)+7 x[-(35-x)^{4}-4(35-x)^{3}(10-x)]$
$ \begin{aligned} & =7(35-x)^{4}(10-x)-7 x(35-x)^{4}-28 x(35-x)^{3}(10-x) \\ & =7(35-x)^{3}[(35-x)(10-x)-x(35-x)-4 x(10-x)] \\ & =7(35-x)^{3}[350-45 x+x^{2}-35 x+x^{2}-40 x+4 x^{2}] \\ & =7(35-x)^{3}(6 x^{2}-120 x+350) \end{aligned} $
Now, $P^{\prime}(x)=0 \Rightarrow x=0, x=35, x=10$
When $x=35,{ }^{\prime}(x)=f(x)=0$ and $y=35-35=0$. This will make the product $x^{2} y^{5}$ equal to 0 .
When $x=0, y=35-0=35$ and the product $x^{2} y^{2}$ will be 0 .
$\square x=0$ and $x=35$ cannot be the possible values of $x$.
When $x=10$, we have:
$ \begin{aligned} P^{\prime \prime}(x) & =7(35-10)^{3}(6 \times 100-120 \times 10+350) \\ & =7(25)^{3}(-250)<0 \end{aligned} $
$\square$ By second derivative test, $P(x)$ will be the maximum when $x=10$ and $y=35-10=$ 25.
Hence, the required numbers are 10 and 25 .
16. Find two positive numbers whose sum is 16 and the sum of whose cubes is minimum.
Show Answer
Solution
Let one number be $x$. Then, the other number is $(16-x)$.
Let the sum of the cubes of these numbers be denoted by $S(x)$. Then,
$S(x)=x^{3}+(16-x)^{3}$
$\therefore S^{\prime}(x)=3 x^{2}-3(16-x)^{2}, S^{\prime \prime}(x)=6 x+6(16-x)$
Now, $S^{\prime}(x)=0 \Rightarrow 3 x^{2}-3(16-x)^{2}=0$
$\Rightarrow x^{2}-(16-x)^{2}=0$
$\Rightarrow x^{2}-256-x^{2}+32 x=0$
$\Rightarrow x=\frac{256}{32}=8$
Now, $S^{\prime \prime}(8)=6(8)+6(16-8)=48+48=96>0$
$\square$ By second derivative test, $x=8$ is the point of local minima of $S$.
Hence, the sum of the cubes of the numbers is the minimum when the numbers are 8 and $16-8=8$.
17. A square piece of tin of side $18 cm$ is to be made into a box without top, by cutting a square from each corner and folding up the flaps to form the box. What should be the side of the square to be cut off so that the volume of the box is the maximum possible.
Show Answer
Solution
Let the side of the square to be cut off be $x cm$. Then, the length and the breadth of the box will be $(18-2 x) cm$ each and the height of the box is $x cm$.
Therefore, the volume $V(x)$ of the box is given by,
$V(x)=x(18-2 x)^{2}$ $\therefore V^{\prime}(x)=(18-2 x)^{2}-4 x(18-2 x)$
$=(18-2 x)[18-2 x-4 x]$
$=(18-2 x)(18-6 x)$
$=6 \times 2(9-x)(3-x)$
$=12(9-x)(3-x)$
And, $V^{\prime \prime}(x)=12[-(9-x)-(3-x)]$
$=-12(9-x+3-x)$
$=-12(12-2 x)$
$=-24(6-x)$
Now, $V^{\prime}(x)=0 \Rightarrow x=9$ or $x=3$
If $x=9$, then the length and the breadth will become 0 .
$\therefore x \neq 9$.
$\Rightarrow x=3$.
Now, $V^{\prime \prime}(3)=-24(6-3)=-72<0$
$\therefore$ By second derivative test, $x=3$ is the point of maxima of $V$.
Hence, if we remove a square of side $3 cm$ from each corner of the square tin and make a box from the remaining sheet, then the volume of the box obtained is the largest possible.
18. A rectangular sheet of tin $45 cm$ by $24 cm$ is to be made into a box without top, by cutting off square from each corner and folding up the flaps. What should be the side of the square to be cut off so that the volume of the box is maximum?
Show Answer
Solution
Let the side of the square to be cut off be $x cm$. Then, the height of the box is $x$, the length is $45-2 x$, and the breadth is $24-2 x$.
Therefore, the volume $V(x)$ of the box is given by,
$ \begin{aligned} V(x) & =x(45-2 x)(24-2 x) \\ & =x(1080-90 x-48 x+4 x^{2}) \\ & =4 x^{3}-138 x^{2}+1080 x \end{aligned} $
$\therefore V^{\prime}(x)=12 x^{2}-276 x+1080$
$ =12(x^{2}-23 x+90) $
$ =12(x-18)(x-5) $
$V^{\prime \prime}(x)=24 x-276=12(2 x-23)$
Now, ${ }^{V^{\prime}}(x)=0 \Rightarrow x=18$ and $x=5$
It is not possible to cut off a square of side $18 cm$ from each corner of the rectangular sheet. Thus, $x$ cannot be equal to 18 .
$\square x=5$
Now, $V^{\prime \prime}(5)=12(10-23)=12(-13)=-156<0$
$\therefore$ By second derivative test, $x=5$ is the point of maxima.
Hence, the side of the square to be cut off to make the volume of the box maximum possible is $5 cm$.
19. Show that of all the rectangles inscribed in a given fixed circle, the square has the maximum area.
Show Answer
Solution
Let a rectangle of length / and breadth $b$ be inscribed in the given circle of radius $a$. Then, the diagonal passes through the centre and is of length $2 a cm$.
Now, by applying the Pythagoras theorem, we have:
$ \begin{aligned} & (2 a)^{2}=l^{2}+b^{2} \\ & \Rightarrow b^{2}=4 a^{2}-l^{2} \\ & \Rightarrow b=\sqrt{4 a^{2}-l^{2}} \end{aligned} $
$\square$ Area of the rectangle, $A=l \sqrt{4 a^{2}-l^{2}}$
$\therefore \frac{d A}{d l}=\sqrt{4 a^{2}-l^{2}}+l \frac{1}{2 \sqrt{4 a^{2}-l^{2}}}(-2 l)=\sqrt{4 a^{2}-l^{2}}-\frac{l^{2}}{\sqrt{4 a^{2}-l^{2}}}$
$ =\frac{4 a^{2}-2 l^{2}}{\sqrt{4 a^{2}-l^{2}}} $
$\frac{d^{2} A}{d l^{2}}=\frac{\sqrt{4 a^{2}-l^{2}}(-4 l)-(4 a^{2}-2 l^{2}) \frac{(-2 l)}{2 \sqrt{4 a^{2}-l^{2}}}}{(4 a^{2}-l^{2})}$
$ =\frac{(4 a^{2}-l^{2})(-4 l)+l(4 a^{2}-2 l^{2})}{(4 a^{2}-l^{2})^{\frac{3}{2}}} $
$ =\frac{-12 a^{2} l+2 l^{3}}{(4 a^{2}-l^{2})^{\frac{3}{2}}}=\frac{-2 l(6 a^{2}-l^{2})}{(4 a^{2}-l^{2})^{\frac{3}{2}}} $
Now, $\frac{d A}{d l}=0$ gives $4 a^{2}=2 l^{2} \Rightarrow l=\sqrt{2} a$
$ \Rightarrow b=\sqrt{4 a^{2}-2 a^{2}}=\sqrt{2 a^{2}}=\sqrt{2} a $
Now, when $l=\sqrt{2} a$,
$\frac{d^{2} A}{d l^{2}}=\frac{-2(\sqrt{2} a)(6 a^{2}-2 a^{2})}{2 \sqrt{2} a^{3}}=\frac{-8 \sqrt{2} a^{3}}{2 \sqrt{2} a^{3}}=-4<0$
$\therefore$ By the second derivative test, when $l=\sqrt{2} a$, then the area of the rectangle is the maximum.
Since $l=b=\sqrt{2} a$, the rectangle is a square.
Hence, it has been proved that of all the rectangles inscribed in the given fixed circle, the square has the maximum area.
20. Show that the right circular cylinder of given surface and maximum volume is such that its height is equal to the diameter of the base.
Show Answer
Solution
Let $r$ and $h$ be the radius and height of the cylinder respectively.
Then, the surface area $(S)$ of the cylinder is given by,
$ \begin{aligned} & S=2 \pi r^{2}+2 \pi r h \\ & \Rightarrow h=\frac{S-2 \pi r^{2}}{2 \pi r} \\ &=\frac{S}{2 \pi}(\frac{1}{r})-r \end{aligned} $
Let $V$ be the volume of the cylinder. Then,
$V=\pi r^{2} h=\pi r^{2}[\frac{S}{2 \pi}(\frac{1}{r})-r]=\frac{S r}{2}-\pi r^{3}$
Then, $\frac{d V}{d r}=\frac{S}{2}-3 \pi r^{2}, \frac{d^{2} V}{d r^{2}}=-6 \pi r$
Now, $\frac{d V}{d r}=0 \Rightarrow \frac{S}{2}=3 \pi r^{2} \Rightarrow r^{2}=\frac{S}{6 \pi}$
When $r^{2}=\frac{S}{6 \pi}$, then $\frac{d^{2} V}{d r^{2}}=-6 \pi(\sqrt{\frac{S}{6 \pi}})<0$.
By second derivative test, the volume is the maximum when $r^{2}=\frac{S}{6 \pi}$.
Now, when $r^{2}=\frac{S}{6 \pi}$, then $h=\frac{6 \pi r^{2}}{2 \pi}(\frac{1}{r})-r=3 r-r=2 r$.
Hence, the volume is the maximum when the height is twice the radius i.e., when the height is equal to the diameter.
21. Of all the closed cylindrical cans (right circular), of a given volume of 100 cubic centimetres, find the dimensions of the can which has the minimum surface area?
Show Answer
Solution
Let $r$ and $h$ be the radius and height of the cylinder respectively.
Then, volume $(V)$ of the cylinder is given by,
$V=\pi r^{2} h=100 \quad$ (given)
$\therefore h=\frac{100}{\pi r^{2}}$
Surface area $(S$ ) of the cylinder is given by,
$S=2 \pi r^{2}+2 \pi r h=2 \pi r^{2}+\frac{200}{r}$
$\therefore \frac{d S}{d r}=4 \pi r-\frac{200}{r^{2}}, \frac{d^{2} S}{d r^{2}}=4 \pi+\frac{400}{r^{3}}$
$\frac{d S}{d r}=0 \Rightarrow 4 \pi r=\frac{200}{r^{2}}$
$\Rightarrow r^{3}=\frac{200}{4 \pi}=\frac{50}{\pi}$
$\Rightarrow r=(\frac{50}{\pi})^{\frac{1}{3}}$
Now, it is observed that when $r=(\frac{50}{\pi})^{\frac{1}{3}}, \frac{d^{2} S}{d r^{2}}>0$.
$\square$ By second derivative test, the surface area is the minimum when the radius of the
cylinder is $(\frac{50}{\pi})^{\frac{1}{3}} cm$.
When $r=(\frac{50}{\pi})^{\frac{1}{3}}, h=\frac{100}{\pi(\frac{50}{\pi})^{\frac{2}{3}}}=\frac{2 \times 50}{(50)^{\frac{2}{3}}()^{1-\frac{2}{3}}}=2(\frac{50}{\pi})^{\frac{1}{3}} cm$.
Hence, the required dimensions of the can which has the minimum surface area is given
by radius $=(\frac{50}{\pi})^{\frac{1}{3}} cm$ and height $=2(\frac{50}{\pi})^{\frac{1}{3}} cm$.
22. A wire of length $28 m$ is to be cut into two pieces. One of the pieces is to be made into a square and the other into a circle. What should be the length of the two pieces so that the combined area of the square and the circle is minimum?
Show Answer
Solution
Let a piece of length / be cut from the given wire to make a square.
Then, the other piece of wire to be made into a circle is of length $(28-I) m$.
Now, side of square $=\frac{l}{4}$.
Let $r$ be the radius of the circle. Then,
$ 2 \pi r=28-l \Rightarrow r=\frac{1}{2 \pi}(28-l) \text{. } $
The combined areas of the square and the circle $(A)$ is given by,
$A=(\text{ side of the square })^{2}+r^{2}$
$=\frac{l^{2}}{16}+\pi[\frac{1}{2 \pi}(28-l)]^{2}$
$=\frac{l^{2}}{16}+\frac{1}{4 \pi}(28-l)^{2}$
$\therefore \frac{d A}{d l}=\frac{2 l}{16}+\frac{2}{4 \pi}(28-l)(-1)=\frac{l}{8}-\frac{1}{2 \pi}(28-l)$
$\frac{d^{2} A}{d l^{2}}=\frac{1}{8}+\frac{1}{2 \pi}>0$
Now, $\frac{d A}{d l}=0 \Rightarrow \frac{l}{8}-\frac{1}{2 \pi}(28-l)=0$
$\Rightarrow \frac{\pi l-4(28-l)}{8 \pi}=0$
$\Rightarrow(\pi+4) l-112=0$
$\Rightarrow l=\frac{112}{\pi+4}$
Thus, when $l=\frac{112}{\pi+4}, \frac{d^{2} A}{d l^{2}}>0$.
$\therefore$ By second derivative test, the area $(A)$ is the minimum when $l=\frac{112}{\pi+4}$.
Hence, the combined area is the minimum when the length of the wire in making the
square is $\frac{112}{\pi+4} cm$ while the length of the wire in making the circle
is $28-\frac{112}{\pi+4}=\frac{28 \pi}{\pi+4} cm$.
23. Prove that the volume of the largest cone that can be inscribed in a sphere of radius $R$ is $\frac{8}{27}$ of the volume of the sphere.
Show Answer
Solution
Let $r$ and $h$ be the radius and height of the cone respectively inscribed in a sphere of radius $R$.
Let $V$ be the volume of the cone.
Then, $V=\frac{1}{3} \pi r^{2} h$
Height of the cone is given by,
$h=R+AB=R+\sqrt{R^{2}-r^{2}} \quad$ [ $ABC$ is a right triangle]
$ \begin{aligned} & \therefore V=\frac{1}{3} \pi r^{2}(R+\sqrt{R^{2}-r^{2}}) \\ & =\frac{1}{3} \pi r^{2} R+\frac{1}{3} \pi r^{2} \sqrt{R^{2}-r^{2}} \\ & \therefore \frac{d V}{d r}=\frac{2}{3} \pi r R+\frac{2}{3} \pi r \sqrt{R^{2}-r^{2}}+\frac{1}{3} \pi r^{2} \cdot \frac{(-2 r)}{2 \sqrt{R^{2}-r^{2}}} \\ & =\frac{2}{3} \pi r R+\frac{2}{3} \pi r \sqrt{R^{2}-r^{2}}-\frac{1}{3} \pi \frac{r^{3}}{\sqrt{R^{2}-r^{2}}} \\ & =\frac{2}{3} \pi r R+\frac{2 \pi r(R^{2}-r^{2})-\pi r^{3}}{3 \sqrt{R^{2}-r^{2}}} \\ & =\frac{2}{3} \pi r R+\frac{2 \pi r R^{2}-3 \pi r^{3}}{3 \sqrt{R^{2}-r^{2}}} \\ & \frac{d^{2} V}{d r^{2}}=\frac{2 \pi R}{3}+\frac{3 \sqrt{R^{2}-r^{2}}(2 \pi R^{2}-9 \pi r^{2})-(2 \pi r R^{2}-3 \pi r^{3}) \cdot \frac{(-2 r)}{6 \sqrt{R^{2}-r^{2}}}}{9(R^{2}-r^{2})} \\ & =\frac{2}{3} \pi R+\frac{9(R^{2}-r^{2})(2 \pi R^{2}-9 \pi r^{2})+2 \pi r^{2} R^{2}+3 \pi r^{4}}{27(R^{2}-r^{2})^{\frac{3}{2}}} \end{aligned} $
Now, $\frac{d V}{d r}=0 \Rightarrow \frac{2}{3} \quad r R=\frac{3 \pi r^{3}-2 \pi r R^{2}}{3 \sqrt{R^{2}-r^{2}}}$
$\Rightarrow 2 R=\frac{3 r^{2}-2 R^{2}}{\sqrt{R^{2}-r^{2}}} \Rightarrow 2 R \sqrt{R^{2}-r^{2}}=3 r^{2}-2 R^{2}$
$\Rightarrow 4 R^{2}(R^{2}-r^{2})=(3 r^{2}-2 R^{2})^{2}$
$\Rightarrow 4 R^{4}-4 R^{2} r^{2}=9 r^{4}+4 R^{4}-12 r^{2} R^{2}$
$\Rightarrow 9 r^{4}=8 R^{2} r^{2}$
$\Rightarrow r^{2}=\frac{8}{9} R^{2}$
When $r^{2}=\frac{8}{9} R^{2}$, then $\frac{d^{2} V}{d r^{2}}<0$.
By second derivative test, the volume of the cone is the maximum when $r^{2}=\frac{8}{9} R^{2}$.
When $r^{2}=\frac{8}{9} R^{2}, h=R+\sqrt{R^{2}-\frac{8}{9} R^{2}}=R+\sqrt{\frac{1}{9} R^{2}}=R+\frac{R}{3}=\frac{4}{3} R$.
Therefore,
$=\frac{1}{3} \pi(\frac{8}{9} R^{2})(\frac{4}{3} R)$
$=\frac{8}{27}(\frac{4}{3} \pi R^{3})$
$=\frac{8}{27} \times($ Volume of the sphere $)$
Hence, the volume of the largest cone that can be inscribed in the sphere is $\frac{8}{27}$ the volume of the sphere.
24. Show that the right circular cone of least curved surface and given volume has an altitude equal to $\sqrt{2}$ time the radius of the base.
Show Answer
Solution
Let $r$ and $h$ be the radius and the height (altitude) of the cone respectively.
Then, the volume $(V)$ of the cone is given as:
$V=\frac{1}{3} \pi r^{2} h \Rightarrow h=\frac{3 V}{r^{2}}$
The surface area $(S)$ of the cone is given by,
$S=\pi r l$ (where $/$ is the slant height)
$=\pi r \sqrt{r^{2}+h^{2}}$
$=\pi r \sqrt{r^{2}+\frac{9 \hbar^{2}}{\pi^{2} r^{4}}} \stackrel{\pi}{=} \frac{r \sqrt{9^{2} r^{6}+V^{2}}}{\pi r^{2}}$
$=\frac{1}{r} \sqrt{\pi^{2} r^{6}+9 V^{2}}$
$ \begin{aligned} \therefore \frac{d S}{d r} & =\frac{r \cdot \frac{6 \pi^{2} r^{5}}{2 \sqrt{{ }^{2} r^{6} 9 V^{2}}}-\sqrt{\pi^{2} r^{6}+9 V^{2}}}{r^{2}} \\ & =\frac{3 \pi^{2} r^{6}-\pi^{2} r^{6}-9 V^{2}}{r^{2} \sqrt{\pi^{2} r^{6}+9 V^{2}}} \\ & =\frac{2 \pi^{2} r^{6}-9 V^{2}}{r^{2} \sqrt{\pi^{2} r^{6}+9 V^{2}}} \\ & =\frac{2 \pi^{2} r^{6}-9 V^{2}}{r^{2} \sqrt{\pi^{2} r^{6}+9 V^{2}}} \end{aligned} $
Now, $\frac{d S}{d r}=0 \Rightarrow 2 \pi^{2} r^{6}=9 V^{2} \Rightarrow r^{6}=\frac{9 V^{2}}{2 \pi^{2}}$
Thus, it can be easily verified that when $r^{6}=\frac{9 V^{2}}{2 \pi^{2}}, \frac{d^{2} S}{d r^{2}}>0$.
$\square$ By second derivative test, the surface area of the cone is the least when $r^{6}=\frac{9 V^{2}}{2 \pi^{2}}$.
When $r^{6}=\frac{9 V^{2}}{2 \pi^{2}}, h=\frac{3 V}{\pi r^{2}}=\frac{3}{\pi r^{2}}(\frac{2 \pi^{2} r^{6}}{9})^{\frac{1}{2}}=\frac{3}{\pi r^{2}} \cdot \frac{\sqrt{2} \pi r^{3}}{3}=\sqrt{2} r$.
Hence, for a given volume, the right circular cone of the least curved surface has an altitude equal to $\sqrt{2}$ times the radius of the base.
25. Show that the semi-vertical angle of the cone of the maximum volume and of given slant height is $\tan ^{-1} \sqrt{2}$.
Show Answer
Solution
Let $\theta$ be the semi-vertical angle of the cone.
It is clear that
$ \theta \in[0, \frac{\pi}{2}] $
Let $r, h$, and $/$ be the radius, height, and the slant height of the cone respectively. The slant height of the cone is given as constant.
Now, $r=I \sin \theta$ and $h=I \cos \theta$
The volume $(V)$ of the cone is given by,
$ \begin{aligned} & V=\frac{1}{3} \pi r^{2} h \\ & =\frac{1}{3} \pi(l^{2} \sin ^{2} \theta)(l \cos \theta) \\ & =\frac{1}{3} \pi l^{3} \sin ^{2} \theta \cos \theta \\ & \begin{aligned} \therefore \frac{d V}{d \theta} & =\frac{l^{3} \pi}{3}[\sin ^{2} \theta(-\sin \theta)+\cos \theta(2 \sin \theta \cos \theta)] \\ & =\frac{l^{3} \pi}{3}[-\sin ^{3}+2 \sin \theta \cos ^{2} \theta] \end{aligned} \\ & \begin{aligned} \frac{d^{2} V}{d \theta^{2}} & =\frac{l^{3} \pi}{3}[-3 \sin ^{2} \theta \cos \theta+2 \cos ^{3} \theta-4 \sin ^{2} \theta \cos \theta] \\ & =\frac{l^{3} \pi}{3}[2 \cos ^{3} \theta-7 \sin ^{2} \theta \cos \theta] \end{aligned} \end{aligned} $
Now, $\frac{d V}{d \theta}=0$
$\Rightarrow \sin ^{3} \theta=2 \sin \theta \cos ^{2} \theta$
$\Rightarrow \tan ^{2} \theta=2$
$\Rightarrow \tan \theta=\sqrt{2}$
$\Rightarrow \theta=\tan ^{-1} \sqrt{2}$
Now, when $\theta=\tan ^{-1} \sqrt{2}$, then $\tan ^{2} \theta=2$ or $\sin ^{2} \theta=2 \cos ^{2} \theta$.
Then, we have:
$\frac{d^{2} V}{d \theta^{2}}=\frac{l^{3} \pi}{3}[2 \cos ^{3} \theta-14 \cos ^{3} \theta]=-4 \pi l^{3} \cos ^{3} \theta<0$ for $\theta \in[0, \frac{\pi}{2}]$
$\square$ By second derivative test, the volume $(V)$ is the maximum when $\theta=\tan ^{-1} \sqrt{2}$.
Hence, for a given slant height, the semi-vertical angle of the cone of the maximum volume is $\tan ^{-1} \sqrt{2}$.
26. Show that semi-vertical angle of right circular cone of given surface area and maximum volume is $\sin ^{-1} \frac{1}{3}$.
Show Answer
Solution
Let $\mathrm{r}, \mathrm{h}, \mathrm{l}$ be the radius, height and slant height of the right circular cone respectively. Let $S$ be the given surface area of the cone. We have, $l^2=\mathrm{r}^2+\mathrm{h}^2 \ldots .(1)$
$\begin{aligned} & S=\pi r l+\pi r^2 \ & S-\pi r^2=\pi r l \ & \Rightarrow l=\frac{S-\pi r^2}{\pi r} \ldots . . \end{aligned}$
$\begin{aligned} & \mathrm{V}=\frac{1}{3} \pi \mathrm{r}^2 \mathrm{~h} \\ & \Rightarrow \mathrm{V}=\frac{1}{3} \pi \mathrm{r}^2 \sqrt{1^2-\mathrm{r}^2}(\mathrm{by}(1)) \\ & \Rightarrow \mathrm{V}^2=\frac{1}{9} \pi^2 \mathrm{r}^4\left(\mathrm{l}^2-\mathrm{r}^2\right) \end{aligned}$
$\begin{aligned} & \Rightarrow \mathrm{V}^2=\frac{1}{9} \pi^2 \mathrm{r}^4\left[\left(\frac{\mathrm{S}-\pi \mathrm{r}^2}{\pi \mathrm{r}}\right)^2-\mathrm{r}^2\right] \\ & \Rightarrow \mathrm{V}^2=\frac{1}{9} \pi^2 \mathrm{r}^4\left[\frac{\left(\mathrm{S}-\pi \mathrm{r}^2\right)^2-\pi^2 \mathrm{r}^4}{\pi^2 \mathrm{r}^2}\right] \\ & \Rightarrow \mathrm{V}^2=\frac{1}{9} \mathrm{r}^2\left[\left(\mathrm{~S}-\pi \mathrm{r}^2\right)^2-\pi^2 \mathrm{r}^4\right] \\ & \Rightarrow \mathrm{V}^2=\frac{1}{9} \mathrm{r}^2\left[\mathrm{~S}^2-2 \pi \mathrm{Sr}^2+\pi^2 \mathrm{r}^4-\pi^2 \mathrm{r}^4\right] \\ & \Rightarrow \mathrm{V}^2=\frac{1}{9}\left(\mathrm{r}^2 \mathrm{~S}^2-2 \pi \mathrm{Sr}^4\right) \\ \end{aligned}$
$2 \mathrm{~V} \frac{\mathrm{dV}}{\mathrm{dr}}=\frac{\mathrm{S}^2}{9} 2 \mathrm{r}-\frac{2 \pi \mathrm{S}}{9} 4 \mathrm{r}^3$
$2 \mathrm{~V} \frac{\mathrm{dV}}{\mathrm{dr}}=\frac{2 \mathrm{rS}}{9}\left(\mathrm{~S}-4 \pi \mathrm{r}^2\right)$
For maximum volume, $\frac{\mathrm{dV}}{\mathrm{dr}}=0$
$\begin{aligned} & \Rightarrow \frac{2 \mathrm{rS}}{9}\left(\mathrm{~S}-4 \pi \mathrm{r}^2\right)=0 \\ & \Rightarrow \mathrm{r}=\mathrm{o} \text { or } \mathrm{S}-4 \pi \mathrm{r}^2=0 \end{aligned}$
Since, $r$ cannot be $o$
$\begin{aligned} & \Rightarrow \mathrm{S}=4 \pi \mathrm{r}^2 \ & \Rightarrow \mathrm{r}^2=\frac{\mathrm{S}}{4 \pi} \ & \Rightarrow \mathrm{r}^2=\frac{\pi \mathrm{rl}+\pi \mathrm{r}^2}{4 \pi} \end{aligned}$
$\begin{aligned} & \Rightarrow 4 \pi r^2=\pi r l+\pi r^2 \ & \Rightarrow 3 \pi r^2=\pi r l \ & \Rightarrow l=3 r \end{aligned}$
Let $\alpha$ be the semi-vertical angle.
$\begin{aligned} & \sin \alpha=\frac{r}{l} \\ & \sin \alpha=\frac{r}{3 r} \\ & \Rightarrow \alpha=\sin ^{-1}\left(\frac{1}{3}\right) \end{aligned}$
Choose the correct answer in Questions 27 and 29.
27. The point on the curve $x^{2}=2 y$ which is nearest to the point $(0,5)$ is
(A) $(2 \sqrt{2}, 4)$
(B) $(2 \sqrt{2}, 0)$
(C) $(0,0)$
(D) $(2,2)$
Show Answer
Solution
The given curve is $x^{2}=2 y$.
For each value of $x$, the position of the point will be $(x, \frac{x^{2}}{2})$.
The distance $d(x)$ between the points $(x, \frac{x^{2}}{2})$ and $(0,5)$ is given by,
$d(x)=\sqrt{(x-0)^{2}+(\frac{x^{2}}{2}-5)^{2}}=\sqrt{x^{2}+\frac{x^{4}}{4}+25-5 x^{2}}=\sqrt{\frac{x^{4}}{4}-4 x^{2}+25}$
$\therefore d^{\prime}(x)=\frac{(x^{3}-8 x)}{2 \sqrt{\frac{x^{4}}{4}-4 x^{2}+25}}=\frac{(x^{3}-8 x)}{\sqrt{x^{4}-16 x^{2}+100}}$
Now, $d^{\prime}(x)=0 \Rightarrow x^{3}-8 x=0$
$\Rightarrow x(x^{2}-8)=0$
$\Rightarrow x=0, \pm 2 \sqrt{2}$
$ \begin{aligned} & \begin{aligned} & \text{ And, } d^{\prime \prime}(x)= \frac{\sqrt{x^{4}-16 x^{2}+100}(3 x^{2}-8)-(x^{3}-8 x) \cdot \frac{4 x^{3}-32 x}{2 \sqrt{x^{4}-16 x^{2}+100}}}{(x^{4}-16 x^{2}+100)} \\ &=\frac{(x^{4}-16 x^{2}+100)(3 x^{2}-8)-2(x^{3}-8 x)(x^{3}-8 x)}{(x^{4}-16 x^{2}+100)^{\frac{3}{2}}} \\ &=\frac{(x^{4}-16 x^{2}+100)(3 x^{2}-8)-2(x^{3}-8 x)^{2}}{(x^{4}-16 x^{2}+100)^{\frac{3}{2}}} \\ & \text{ When, } x=0 \text{, then } d^{\prime \prime}(x)=\frac{36(-8)}{6^{3}}<0 . \end{aligned} \\ & \text{ When, } x= \pm 2 \sqrt{2}, d^{\prime \prime}(x)>0 . \end{aligned} $
$\square$ By second derivative test, $d(x)$ is the minimum at $x= \pm 2 \sqrt{2}$.
When $x= \pm 2 \sqrt{2}, y=\frac{(2 \sqrt{2})^{2}}{2}=4$.
Hence, the point on the curve $x^{2}=2 y$ which is nearest to the point $(0,5)$ is $( \pm 2 \sqrt{2}, 4)$. The correct answer is $A$.
28. For all real values of $x$, the minimum value of $\frac{1-x+x^{2}}{1+x+x^{2}}$ is
(A) 0
(B) 1
(C) 3
(D) $\frac{1}{3}$
Show Answer
Solution
Let $f(x)=\frac{1-x+x^{2}}{1+x+x^{2}}$.
$ \begin{aligned} \therefore f^{\prime}(x) & =\frac{(1+x+x^{2})(-1+2 x)-(1-x+x^{2})(1+2 x)}{(1+x+x^{2})^{2}} \\ & =\frac{-1+2 x-x+2 x^{2}-x^{2}+2 x^{3}-1-2 x+x+2 x^{2}-x^{2}-2 x^{3}}{(1+x+x^{2})^{2}} \\ & =\frac{2 x^{2}-2}{(1+x+x^{2})^{2}}=\frac{2(x^{2}-1)}{(1+x+x^{2})^{2}} \end{aligned} $
$\therefore f^{\prime}(x)=0 \Rightarrow x^{2}=1 \Rightarrow x= \pm 1$
Now, $f^{\prime \prime}(x)=\frac{2[(1+x+x^{2})^{2}(2 x)-(x^{2}-1)(2)(1+x+x^{2})(1+2 x)]}{(1+x+x^{2})^{4}}$
$ =\frac{4(1+x+x^{2})[(1+x+x^{2}) x-(x^{2}-1)(1+2 x)]}{(1+x+x^{2})^{4}} $
$ \begin{aligned} & =\frac{4[x+x^{2}+x^{3}-x^{2}-2 x^{3}+1+2 x]}{(1+x+x^{2})^{3}} \\ & =\frac{4(1+3 x-x^{3})}{(1+x+x^{2})^{3}} \end{aligned} $
And, $f^{\prime \prime}(1)=\frac{4(1+3-1)}{(1+1+1)^{3}}=\frac{4(3)}{(3)^{3}}=\frac{4}{9}>0$
Also, $f^{\prime \prime}(-1)=\frac{4(1-3+1)}{(1-1+1)^{3}}=4(-1)=-4<0$
$\square$ By second derivative test, $f$ is the minimum at $x=1$ and the minimum value is given
by $f(1)=\frac{1-1+1}{1+1+1}=\frac{1}{3}$.
The correct answer is $D$.
29. The maximum value of $[x(x-1)+1]^{\frac{1}{3}}, 0 \leq x \leq 1$ is
(A) $\frac{1}{3} \frac{1}{3}$
(B) $\frac{1}{2}$
(C) 1
(D) 0
Show Answer
Solution
Let $f(x)=[x(x-1)+1]^{\frac{1}{3}}$.
$\therefore f^{\prime}(x)=\frac{2 x-1}{3[x(x-1)+1]^{\frac{2}{3}}}$
Now, $f^{\prime}(x)=0 \Rightarrow x=\frac{1}{2}$
Then, we evaluate the value of $f$ at critical point $x=\frac{1}{2}$ and at the end points of the interval $[0,1]$ {i.e., at $x=0$ and $x=1}$.
$f(0)=[0(0-1)+1]^{\frac{1}{3}}=1$
$f(1)=[1(1-1)+1]^{\frac{1}{3}}=1$
$f(\frac{1}{2})=[\frac{1}{2}(\frac{-1}{2})+1]^{\frac{1}{3}}=(\frac{3}{4})^{\frac{1}{3}}$
Hence, we can conclude that the maximum value of $f$ in the interval $[0,1]$ is 1 .
The correct answer is $C$.
Miscellaneous Examples
Example 30 A car starts from a point $P$ at time $t=0$ seconds and stops at point $Q$. The distance $x$, in metres, covered by it, in $t$ seconds is given by
$$ x=t^{2}\left(2-\frac{t}{3}\right) \text { } $$
Find the time taken by it to reach $Q$ and also find distance between $P$ and $Q$.
Solution Let $v$ be the velocity of the car at $t$ seconds.
Now $ \begin{aligned} & x=t^{2}\left(2-\frac{t}{3}\right) \end{aligned} $
Therefore $ \begin{aligned} & v=\frac{d x}{d t}=4 t-t^{2}=t(4-t) \end{aligned} $
Thus, $v=0$ gives $t=0$ and/or $t=4$. Now $v=0$ at $P$ as well as at $Q$ and at $P, t=0$. So, at $Q, t=4$. Thus, the car will reach the point $Q$ after 4 seconds. Also the distance travelled in 4 seconds is given by
$$ x] _{t=4}=4^{2}(2-\frac{4}{3})=16(\frac{2}{3})=\frac{32}{3} m $$
Example 32 A man of height 2 metres walks at a uniform speed of $5 km / h$ away from a lamp post which is 6 metres high. Find the rate at which the length of his shadow increases.
Solution In Fig 6.21, Let AB be the lamp-post, the lamp being at the position $B$ and let $MN$ be the man at a particular time $t$ and let $AM=l$ metres. Then, MS is the shadow of the man. Let MS $=s$ metres.
Note that $\triangle \mathrm{ASB} \sim \triangle \mathrm{MSN}$
or $\frac{\mathrm{MS}}{\mathrm{AS}}=\frac{\mathrm{MN}}{\mathrm{AB}}$
or $\mathrm{AS}=3 s$
Fig 6.21
$\mathrm{MN}=2 \mathrm{~m}$ और $\mathrm{AB}=6 \mathrm{~m}$ (given)
$\mathrm{AM}=3 s-s=2 s$ है। परन्तु $\mathrm{AM}=l$
so $\quad l=2 s$
Since $\frac{d l}{d t}=5 km / h$.
Hence, the length of the shadow increases at the rate $\frac{5}{2} km / h$.
Example 33 Find intervals in which the function given by
$$ f(x)=\frac{3}{10} x^{4}-\frac{4}{5} x^{3}-3 x^{2}+\frac{36}{5} x+11 $$
is (a) increasing (b) decreasing.
Solution We have
$$ f(x)=\frac{3}{10} x^{4}-\frac{4}{5} x^{3}-3 x^{2}+\frac{36}{5} x+11 $$ $$ \begin{aligned} f^{\prime}(x) & =\frac{3}{10}(4 x^{3})-\frac{4}{5}(3 x^{2})-3(2 x)+\frac{36}{5} \\ & =\frac{6}{5}(x-1)(x+2)(x-3) \quad \text{ (on simplification) } \end{aligned} $$
Now $f^{\prime}(x)=0$ gives $x=1, x=-2$, or $x=3$. The points $x=1,-2$, and 3 divide the real line into four disjoint intervals namely, $(-\infty,-2),(-2,1),(1,3)$ and $(3, \infty)$ (Fig 6.22).
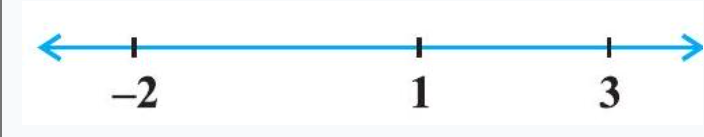
Fig 6.22
Consider the interval $(-\infty,-2)$, i.e., when $-\infty<x<-2$.
In this case, we have $x-1<0, x+2<0$ and $x-3<0$.
(In particular, observe that for $x=-3, f^{\prime}(x)=(x-1)(x+2)(x-3)=(-4)(-1)$ $(-6)<0)$
Therefore, $\quad f^{\prime}(x)<0$ when $-\infty<x<-2$.
Thus, the function $f$ is decreasing in $(-\infty,-2)$.
Consider the interval $(-2,1)$, i.e., when $-2<x<1$.
In this case, we have $x-1<0, x+2>0$ and $x-3<0$
(In particular, observe that for $x=0, f^{\prime}(x)=(x-1)(x+2)(x-3)=(-1)(2)(-3)$ $=6>0)$
So $ f^{\prime}(x)>0 \text{ when }-2<x<1 \text{. } $
Thus, $ f \text{ is increasing in }(-2,1) \text{. } $
Now consider the interval $(1,3)$, i.e., when $1<x<3$. In this case, we have $x-1>0, x+2>0$ and $x-3<0$.
$ So, \qquad f^{\prime}(x)<0 \text{ when } 1<x<3 \text{. } $
Thus, $f$ is decreasing in $(1,3)$.
Finally, consider the interval $(3, \infty)$, i.e., when $x>3$. In this case, we have $x-1>0$, $x+2>0$ and $x-3>0$. So $f^{\prime}(x)>0$ when $x>3$.
Thus, $f$ is increasing in the interval $(3, \infty)$.
Example 34 Show that the function $f$ given by
$ f(x)=\tan ^{-1}(\sin x+\cos x), x>0 $
is always an increasing function in $0, \frac{\pi}{4}$.
Solution We have
Therefore $ \begin{aligned} f(x) & =\tan ^{-1}(\sin x+\cos x), x>0 \\ f^{\prime}(x) & =\frac{1}{1+(\sin x+\cos x)^{2}}(\cos x-\sin x) \\ & =\frac{\cos x-\sin x}{2+\sin 2 x} \end{aligned} $
Note that $2+\sin 2 x>0$ for all $x$ in $0, \frac{\pi}{4}$.
Therefore $\qquad f^{\prime}(x)>0 \text{ if } \cos x-\sin x>0$
or $\qquad f^{\prime}(x)>0 \text{ if } \cos x>\sin x \text{ or } \cot x>1$
Now $\qquad \cot x>1 \text{ if } \tan x<1 \text{, i.e., if } 0<x<\frac{\pi}{4}$
Thus $\qquad f^{\prime}(x)>0 \text{ in } 0, \frac{\pi}{4}$
Hence $f$ is increasing function in $(0, \frac{\pi}{4})$.
Example 35 A circular disc of radius $3 cm$ is being heated. Due to expansion, its radius increases at the rate of $0.05 cm / s$. Find the rate at which its area is increasing when radius is $3.2 cm$.
Solution Let $r$ be the radius of the given disc and $A$ be its area. Then
Then $\qquad \mathrm{A}=\pi r^{2}$
or $\qquad \frac{d A}{d t}=2 \pi r \frac{d r}{d t}$
Now approximate rate of increase of radius $=d r=\frac{d r}{d t} \Delta t=0.05 cm / s$.
Therefore, the approximate rate of increase in area is given by
$ \begin{aligned} d A & =\frac{d A}{d t}(\Delta t)=2 \pi r(\frac{d r}{d t} \Delta t) \\ & =2 \pi(3.2)(0.05)=0.320 \pi cm^{2} / s \quad(r=3.2 cm) \end{aligned} $
Example 36 An open topped box is to be constructed by removing equal squares from each corner of a 3 metre by 8 metre rectangular sheet of aluminium and folding up the sides. Find the volume of the largest such box.
Solution Let $x$ metre be the length of a side of the removed squares. Then, the height of the box is $x$, length is $8-2 x$ and breadth is $3-2 x$ (Fig 6.23). If $V(x)$ is the volume of the box, then
 Fig 6.23
Fig 6.23
$ \begin{aligned} V(x) & =x(3-2 x)(8-2 x) \\ & =4 x^{3}-22 x^{2}+24 x \end{aligned} $
Therefore $\qquad \begin{cases} V^{\prime}(x)=12 x^{2}-44 x+24=4(x-3)(3 x-2) \\ V^{\prime \prime}(x)=24 x-44 \end{cases}$
Now $\qquad V^{\prime}(x)=0 \text{ gives } x=3, \frac{2}{3} \text{. But } x \neq 3 \text{ (Why?) }$
Thus, we have $x=\frac{2}{3}$. Now $V^{\prime \prime}(\frac{2}{3})=24(\frac{2}{3})-44=-28<0$.
Therefore, $x=\frac{2}{3}$ is the point of maxima, i.e., if we remove a square of side $\frac{2}{3}$ metre from each corner of the sheet and make a box from the remaining sheet, then the volume of the box such obtained will be the largest and it is given by
$\mathrm{V}\left(\frac{2}{3}\right)=4\left(\frac{2}{3}\right)^{3}-22\left(\frac{2}{3}\right)^{2}+24\left(\frac{2}{3}\right)=\frac{200}{27} \mathrm{~m}^{3}$
Example 37 Manufacturer can sell $x$ items at a price of rupees $5-\frac{x}{100}$ each. The cost price of $x$ items is $Rs \frac{x}{5}+500$. Find the number of items he should sell to earn maximum profit.
Solution Let $S(x)$ be the selling price of $x$ items and let $C(x)$ be the cost price of $x$ items. Then, we have
$$ S(x)=\left(5-\frac{x}{100}\right) x=5 x-\frac{x^{2}}{100} $$
and $\qquad \mathrm{C}(x)=\frac{x}{5}+500$
Thus, the profit function $P(x)$ is given by
i.e. $\qquad \mathrm{P}(x)=\mathrm{S}(x)-\mathrm{C}(x)=5 x-\frac{x^{2}}{100}-\frac{x}{5}-500$
$$ \mathrm{P}(x)=\frac{24}{5} x-\frac{x^{2}}{100}-500 $$
Now $\qquad P^{\prime}(x)=0$ gives $x=240$. Also $P^{\prime \prime}(x)=\frac{-1}{50}$. So $\qquad P^{\prime \prime}(240)=\frac{-1}{50}<0$
Thus, $x=240$ is a point of maxima. Hence, the manufacturer can earn maximum profit, if he sells 240 items.
Miscellaneous Exercise on Chapter 6
1. Show that the function given by $f(x)=\frac{\log x}{x}$ has maximum at $x=e$.
Show Answer
Solution
The given function is $f(x)=\frac{\log x}{x}$.
$ f^{\prime}(x)=\frac{x(\frac{1}{x})-\log x}{x^{2}}=\frac{1-\log x}{x^{2}} $
Now, $f^{\prime}(x)=0$
$\Rightarrow 1-\log x=0$ $\Rightarrow \log x=1$
$\Rightarrow \log x=\log e$
$\Rightarrow x=e$
Now, $f^{\prime \prime}(x)=\frac{x^{2}(-\frac{1}{x})-(1-\log x)(2 x)}{x^{4}}$
$ \begin{aligned} & =\frac{-x-2 x(1-\log x)}{x^{4}} \\ & =\frac{-3+2 \log x}{x^{3}} \end{aligned} $
Now, $f^{\prime \prime}(e)=\frac{-3+2 \log e}{e^{3}}=\frac{-3+2}{e^{3}}=\frac{-1}{e^{3}}<0$
Therefore, by second derivative test, $f$ is the maximum at $x=e$.
2. The two equal sides of an isosceles triangle with fixed base $b$ are decreasing at the rate of $3 cm$ per second. How fast is the area decreasing when the two equal sides are equal to the base?
Show Answer
Solution
Let $\triangle A B C$ be isosceles where $B C$ is the base of fixed length $b$.
Let the length of the two equal sides of $\triangle A B C$ be $a$.
Draw AD $\square BC$.
Now, in $\triangle A D C$, by applying the Pythagoras theorem, we have:
$AD=\sqrt{a^{2}-\frac{b^{2}}{4}}$
Area of triangle
$ (A)=\frac{1}{2} b \sqrt{a^{2}-\frac{b^{2}}{4}} $
The rate of change of the area with respect to time $(t)$ is given by,
$\frac{d A}{d t}=\frac{1}{2} b \cdot \frac{2 a}{2 \sqrt{a^{2}-\frac{b^{2}}{4}}} \frac{d a}{d t}=\frac{a b}{\sqrt{4 a^{2}-b^{2}}} \frac{d a}{d t}$
It is given that the two equal sides of the triangle are decreasing at the rate of $3 cm$ per second.
$ \begin{aligned} & \\ & \square \frac{d a}{d t}=-3 cm / s \\ & \therefore \frac{d A}{d t}=\frac{-3 a b}{\sqrt{4 a^{2}-b^{2}}} \end{aligned} $
Then, when $a=b$, we have:
$\frac{d A}{d t}=\frac{-3 b^{2}}{\sqrt{4 b^{2}-b^{2}}}=\frac{-3 b^{2}}{\sqrt{3 b^{2}}}=-\sqrt{3} b$
Hence, if the two equal sides are equal to the base, then the area of the triangle is decreasing at the rate of $\sqrt{3} bm cm^{2} / s$.
3. Find the intervals in which the function $f$ given by
$$ f(x)=\frac{4 \sin x-2 x-x \cos x}{2+\cos x} $$
is (i) increasing (ii) decreasing.
Show Answer
Solution
$f(x)=\frac{4 \sin x-2 x-x \cos x}{2+\cos x}$
$\therefore f^{\prime}(x)=\frac{(2+\cos x)(4 \cos x-2-\cos x+x \sin x)-(4 \sin x-2 x-x \cos x)(-\sin x)}{(2+\cos x)^{2}}$
$=\frac{(2+\cos x)(3 \cos x-2+x \sin x)+\sin x(4 \sin x-2 x-x \cos x)}{(2+\cos x)^{2}}$
$=\frac{6 \cos x-4+2 x \sin x+3 \cos ^{2} x-2 \cos x+x \sin x \cos x+4 \sin ^{2} x-2 x \sin x-x \sin x \cos x}{(2+\cos x)^{2}}$
$=\frac{4 \cos x-4+3 \cos ^{2} x+4 \sin ^{2} x}{(2+\cos x)^{2}}$
$=\frac{4 \cos x-4+3 \cos ^{2} x+4-4 \cos ^{2} x}{(2+\cos x)^{2}}$
$=\frac{4 \cos x-\cos ^{2} x}{(2+\cos x)^{2}}=\frac{\cos x(4-\cos x)}{(2+\cos x)^{2}}$
Now, $f^{\prime}(x)=0$
$\Rightarrow \cos x=0$ or $\cos x=4$
But, $\cos x \neq 4$
$\square \cos x=0$
$\Rightarrow x=\frac{\pi}{2}, \frac{3 \pi}{2}$
Now, $x=\frac{\pi}{2}$ and $x=\frac{3 \pi}{2}$ divides $(0,2 \pi)$ into three disjoint intervals i.e.,
$(0, \frac{\pi}{2}),(\frac{\pi}{2}, \frac{3 \pi}{2})$, and $(\frac{3 \pi}{2}, 2 \pi)$.
In intervals $(0, \frac{\pi}{2})$ and $(\frac{3 \pi}{2}, 2 \pi), f^{\prime}(x)>0$.
Thus, $f(x)$ is increasing for $0<x<\frac{x}{2}$ and $\frac{3 \pi}{2}<x<2 \pi$.
In the interval $(\frac{\pi}{2}, \frac{3 \pi}{2}), f^{\prime}(x)<0$.
Thus, $f(x)$ is decreasing for $\frac{\pi}{2}<x<\frac{3 \pi}{2}$.
4. Find the intervals in which the function $f$ given by $f(x)=x^{3}+\frac{1}{x^{3}}, x \neq 0$ is
(i) increasing
(ii) decreasing.
Show Answer
Solution
$f(x)=x^{3}+\frac{1}{x^{3}}$
$\therefore f^{\prime}(x)=3 x^{2}-\frac{3}{x^{4}}=\frac{3 x^{6}-3}{x^{4}}$
Then, $f^{\prime}(x)=0 \Rightarrow 3 x^{6}-3=0 \Rightarrow x^{6}=1 \Rightarrow x= \pm 1$
Now, the points $x=1$ and $x=-1$ divide the real line into three disjoint intervals
i.e., $(-\infty,-1),(-1,1)$, and $(1, \infty)$.
In intervals $(-\infty,-1)$ and $(1, \infty)$ i.e., when $x<-1$ and $x>1, f^{\prime}(x)>0$.
Thus, when $x<-1$ and $x>1, f$ is increasing.
In interval $(-1,1)$ i.e., when $-1<x<1, f^{\prime}(x)<0$.
Thus, when $-1<x<1, f$ is decreasing.
5. Find the maximum area of an isosceles triangle inscribed in the ellipse $\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$ with its vertex at one end of the major axis.
Show Answer
Solution

The given ellipse is $\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$.
Let the major axis be along the $x$-axis.
Let $A B C$ be the triangle inscribed in the ellipse where vertex $C$ is at $(a, 0)$.
Since the ellipse is symmetrical with respect to the $x$-axis and $y$-axis, we can assume the coordinates of $A$ to be $(-x_1, y_1)$ and the coordinates of $B$ to be $(-x_1,-y_1)$.
Now, we have $y_1= \pm \frac{b}{a} \sqrt{a^{2}-x_1^{2}}$.
$\square$ Coordinates of $A$ are $(-x_1, \frac{b}{a} \sqrt{a^{2}-x_1^{2}})$ and the coordinates of $B$ are $(x_1,-\frac{b}{a} \sqrt{a^{2}-x_1^{2}})$.
As the point $(x_1, y_1)$ lies on the ellipse, the area of triangle $ABC(A)$ is given by,
$ \begin{aligned} & A=\frac{1}{2}|a(\frac{2 b}{a} \sqrt{a^{2}-x_1^{2}})+(-x_1)(-\frac{b}{a} \sqrt{a^{2}-x_1^{2}})+(-x_1)(-\frac{b}{a} \sqrt{a^{2}-x_1^{2}})| \\ & \Rightarrow A=b \sqrt{a^{2}-x_1^{2}}+x_1 \frac{b}{a} \sqrt{a^{2}-x_1^{2}} \\ & \therefore \frac{d A}{d x_1}=\frac{-2 x_1 b}{2 \sqrt{a^{2}-x_1^{2}}}+\frac{b}{a} \sqrt{a^{2}-x_1^{2}}-\frac{2 b x_1^{2}}{a 2 \sqrt{a^{2}-x_1^{2}}} \\ & =\frac{b}{a \sqrt{a^{2}-x_1^{2}}}[-x_1 a+(a^{2}-x_1^{2})-x_1^{2}] \\ & =\frac{b(-2 x_1^{2}-x_1 a+a^{2})}{a \sqrt{a^{2}-x_1^{2}}} \end{aligned} $
Now, $\frac{d A}{d x_1}=0$
$\Rightarrow-2 x_1^{2}-x_1 a+a^{2}=0$
$\Rightarrow x_1=\frac{a \pm \sqrt{a^{2}-4(-2)(a^{2})}}{2(-2)}$
$=\frac{a \pm \sqrt{9 a^{2}}}{-4}$
$=\frac{a \pm 3 a}{-4}$
$\Rightarrow x_1=-a, \frac{a}{2}$
But, $x_1$ cannot be equal to $a$. $\therefore x_1=\frac{a}{2} \Rightarrow y_1=\frac{b}{a} \sqrt{a^{2}-\frac{a^{2}}{4}}=\frac{b a}{2 a} \sqrt{3}=\frac{\sqrt{3} b}{2}$
Now, $\frac{d^{2} A}{d x_1^{2}}=\frac{b}{a}{\frac{\sqrt{a^{2}-x_1^{2}}(-4 x_1-a)-(-2 x_1^{2}-x_1 a+a^{2}) \frac{(-2 x_1)}{2 \sqrt{a^{2}-x_1^{2}}}}{a^{2}-x_1^{2}}}$
$ =\frac{b}{a}{\frac{(a^{2}-x_1^{2})(-4 x_1-a)+x_1(-2 x_1^{2}-x_1 a+a^{2})}{(a^{2}-x_1^{2})^{\frac{3}{2}}}} $
$ =\frac{b}{a}{\frac{2 x^{3}-3 a^{2} x-a^{3}}{(a^{2}-x_1^{2})^{\frac{3}{2}}}} $
Also, when $x_1=\frac{a}{2}$, then
$ \begin{aligned} \frac{d^{2} A}{d x_1^{2}} & =\frac{b}{a}{\frac{2 \frac{a^{3}}{8}-3 \frac{a^{3}}{2}-a^{3}}{(\frac{3 a^{2}}{4})^{\frac{3}{2}}}}=\frac{b}{a}{\frac{\frac{a^{3}}{4}-\frac{3}{2} a^{3}-a^{3}}{(\frac{3 a^{2}}{4})^{\frac{3}{2}}}} \\ & =-\frac{b}{a}{\frac{\frac{9}{4} a^{3}}{(\frac{3 a^{2}}{4})^{\frac{3}{2}}}}<0 \end{aligned} $
Thus, the area is the maximum when $x_1=\frac{a}{2}$.
$\square$ Maximum area of the triangle is given by,
$ \begin{aligned} A & =b \sqrt{a^{2}-\frac{a^{2}}{4}}+(\frac{a}{2}) \frac{b}{a} \sqrt{a^{2}-\frac{a^{2}}{4}} \\ & =a b \frac{\sqrt{3}}{2}+(\frac{a}{2}) \frac{b}{a} \times \frac{a \sqrt{3}}{2} \\ & =\frac{a b \sqrt{3}}{2}+\frac{a b \sqrt{3}}{4}=\frac{3 \sqrt{3}}{4} a b \end{aligned} $
6. A tank with rectangular base and rectangular sides, open at the top is to be constructed so that its depth is $2 m$ and volume is $8 m^{3}$. If building of tank costs Rs 70 per sq metres for the base and Rs 45 per square metre for sides. What is the cost of least expensive tank?
Show Answer
Solution
Let $l, b$, and $h$ represent the length, breadth, and height of the tank respectively.
Then, we have height $(h)=2 m$
Volume of the tank $=8 m^{3}$
Volume of the tank $=l \times b \times h$
$\square 8=l \times b \times 2$
$\Rightarrow l b=4 \Rightarrow b=\frac{4}{l}$
Now, area of the base $=I b=4$
Area of the 4 walls $(A)=2 h(I+b)$
$\therefore A=4(l+\frac{4}{l})$
$\Rightarrow \frac{d A}{d l}=4(1-\frac{4}{l^{2}})$
Now, $\frac{d A}{d l}=0$
$\Rightarrow 1-\frac{4}{l^{2}}=0$
$\Rightarrow l^{2}=4$
$\Rightarrow l= \pm 2$
However, the length cannot be negative.
Therefore, we have $I=4$.
$\therefore b=\frac{4}{l}=\frac{4}{2}=2$
Now, $\frac{d^{2} A}{d l^{2}}=\frac{32}{l^{3}}$
When $l=2, \frac{d^{2} A}{d l^{2}}=\frac{32}{8}=4>0$.
Thus, by second derivative test, the area is the minimum when $I=2$.
We have $I=b=h=2$.
$\square$ Cost of building the base $=Rs 70 \times(I b)=Rs 70(4)=Rs 280$
Cost of building the walls $=Rs 2 h(I+b) \times 45=Rs 90(2)(2+2)$
$=Rs 8(90)=Rs 720$
Required total cost $=Rs(280+720)=Rs 1000$
Hence, the total cost of the tank will be Rs 1000.
7. The sum of the perimeter of a circle and square is $k$, where $k$ is some constant. Prove that the sum of their areas is least when the side of square is double the radius of the circle.
Show Answer
Solution
Let $r$ be the radius of the circle and $a$ be the side of the square.
Then, we have:
$2 \pi r+4 a=k$ (where $k$ is constant)
$\Rightarrow a=\frac{k-2 \pi r}{4}$
The sum of the areas of the circle and the square $(A)$ is given by,
$A=\pi r^{2}+a^{2}=\pi r^{2}+\frac{(k-2 \pi r)^{2}}{16}$
$\therefore \frac{d A}{d r}=2 \pi r+\frac{2(k-2 \pi r)(-2 \pi)}{16}=2 \pi r-\frac{\pi(k-2 \pi r)}{4}$
Now, $\frac{d A}{d r}=0$
$\Rightarrow 2 \pi r=\frac{\pi(k-2 \pi r)}{4}$
$8 r=k-2 \pi r$
$\Rightarrow(8+2 \pi) r=k$
$\Rightarrow r=\frac{k}{8+2 \pi}=\frac{k}{2(4+\pi)}$
Now, $\frac{d^{2} A}{d r^{2}}=2 \pi+\frac{\pi^{2}}{2}>0$
$\therefore$ When $r=\frac{k}{2(4 \pi)}, \frac{d^{2} A}{d r^{2}}>0$.
When $r=\frac{k}{2(4 \pi)}, a=\frac{k-2 \pi[\frac{k}{2(4 \pi)}]}{4}=\frac{k(4 \pi) \pi k}{4(\pi)}=\frac{4 k}{4(\pi)}=\frac{k}{\pi}=2 r$.
Hence, it has been proved that the sum of their areas is least when the side of the square is double the radius of the circle.
8. A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is $10 m$. Find the dimensions of the window to admit maximum light through the whole opening.
Show Answer
Solution
Let $x$ and $y$ be the length and breadth of the rectangular window.
Radius of the semicircular opening $=\frac{x}{2}$

It is given that the perimeter of the window is $10 m$.
$\therefore x+2 y+\frac{\pi x}{2}=10$
$\Rightarrow x(1+\frac{\pi}{2})+2 y=10$
$\Rightarrow 2 y=10-x(1+\frac{\pi}{2})$
$\Rightarrow y=5-x(\frac{1 \pi}{2}+\frac{-}{4})$
$\square$ Area of the window $(A)$ is given by,
$ \begin{aligned} A & =x y+\frac{\pi}{2}(\frac{x}{2})^{2} \\ & =x[5-x(\frac{1 \pi}{2}+\frac{\pi}{4})]+\frac{x^{2}}{8} \\ & =5 x-x^{2}(\frac{1 \pi}{2}+\frac{\pi}{4})+\frac{\pi}{8} x^{2} \\ \therefore & \frac{d A}{d x}=5-2 x(\frac{1 \pi}{2}+\frac{\pi}{4})+\frac{\pi}{4} x \\ & =5-x(1+\frac{\pi}{2})+\frac{\pi}{4} x \\ \therefore & \frac{d^{2} A}{d x^{2}}=-(1+\frac{\pi}{2})+\frac{\pi}{4}=-1-\frac{\pi}{4} \end{aligned} $
Now, $\frac{d A}{d x}=0$
$\Rightarrow 5-x(1+\frac{\pi}{2})+\frac{\pi}{4} x=0$
$\Rightarrow 5-x-\frac{\pi}{4} x=0$
$\Rightarrow x(1+\frac{\pi}{4})=5$
$\Rightarrow x=\frac{5}{(1+\frac{\pi}{4})}=\frac{20}{\pi+4}$
Thus, when $x=\frac{20}{\pi+4}$ then $\frac{d^{2} A}{d x^{2}}<0$.
Therefore, by second derivative test, the area is the maximum when length $x=\frac{20}{\pi+4} m$. Now,
$y=5-\frac{20}{\pi+4}(\frac{2+\pi}{4})=5-\frac{5(2+\pi)}{\pi+4}=\frac{10}{\pi+4} m$
Hence, the required dimensions of the window to admit maximum light is given
by length $=\frac{20}{\pi+4} m$ and breadth $=\frac{10}{\pi+4} m$.
9. A point on the hypotenuse of a triangle is at distance $a$ and $b$ from the sides of the triangle.Show that the minimum length of the hypotenuse is
$\left(a^{\frac{2}{3}}+b^{\frac{2}{3}}\right)^{\frac{3}{2}}$.
Show Answer
Solution
Let $\triangle A B C$ be right-angled at $B$. Let $A B=x$ and $B C=y$.
Let $P$ be a point on the hypotenuse of the triangle such that $P$ is at a distance of $a$ and $b$ from the sides $A B$ and $B C$ respectively.
Let $\square C=\theta$.
We have,
$AC=\sqrt{x^{2}+y^{2}}$
Now,
$PC=b cosec \theta$
And, $AP=a \sec \theta$
$\square AC=AP+PC$
$\square AC=b cosec \theta+a \sec \theta \ldots$ (1)
$\therefore \frac{d(AC)}{d \theta}=-b cosec \theta \cot \theta+a \sec \theta \tan \theta$
$\therefore \frac{d(AC)}{d \theta}=0$
$\Rightarrow a \sec \theta \tan \theta=b cosec \theta \cot \theta$
$\Rightarrow \frac{a}{\cos \theta} \cdot \frac{\sin \theta}{\cos \theta}=\frac{b}{\sin \theta} \frac{\cos \theta}{\sin \theta}$
$\Rightarrow a \sin ^{3} \theta=b \cos ^{3} \theta$
$\Rightarrow(a)^{\frac{1}{3}} \sin \theta=(b)^{\frac{1}{3}} \cos \theta$
$\Rightarrow \tan \theta=(\frac{b}{a})^{\frac{1}{3}}$
$\therefore \sin \theta=\frac{(b)^{\frac{1}{3}}}{\sqrt{a^{\frac{2}{3}}+b^{\frac{2}{3}}}}$ and $\cos \theta=\frac{(a)^{\frac{1}{3}}}{\sqrt{a^{\frac{2}{3}}+b^{\frac{2}{3}}}}$
It can be clearly shown that $\frac{d^{2}(AC)}{d \theta^{2}}<0$ when $\tan \theta=(\frac{b}{a})^{\frac{1}{3}}$.
Therefore, by second derivative test, the length of the hypotenuse is the maximum when
$\tan \theta=(\frac{b}{a})^{\frac{1}{3}}$.
Now, when $\tan \theta=(\frac{b}{a})^{\frac{1}{3}}$, we have:
$ \begin{aligned} AC & =\frac{b \sqrt{a^{\frac{2}{3}}+b^{\frac{2}{3}}}}{b^{\frac{1}{3}}}+\frac{a \sqrt{a^{\frac{2}{3}}+b^{\frac{2}{3}}}}{a^{\frac{1}{3}}} \\ & =\sqrt{a^{\frac{2}{3}}+b^{\frac{2}{3}}}(b^{\frac{2}{3}}+a^{\frac{2}{3}}) \\ & =(a^{\frac{2}{3}}+b^{\frac{2}{3}})^{\frac{3}{2}} \end{aligned} $
Hence, the maximum length of the hypotenuses is $(a^{\frac{2}{3}}+b^{\frac{2}{3}})^{\frac{3}{2}}$.
10. Find the points at which the function $f$ given by $f(x)=(x-2)^{4}(x+1)^{3}$ has
(i) local maxima
(ii) local minima
(iii) point of inflexion
Show Answer
Solution
The given function is $f(x)=(x-2)^{4}(x+1)^{3}$.
$ \begin{aligned} \therefore f^{\prime}(x) & =4(x-2)^{3}(x+1)^{3}+3(x+1)^{2}(x-2)^{4} \\ & =(x-2)^{3}(x+1)^{2}[4(x+1)+3(x-2)] \\ & =(x-2)^{3}(x+1)^{2}(7 x-2) \end{aligned} $
Now, $f^{\prime}(x)=0 \Rightarrow x=-1$ and $x=\frac{2}{7}$ or $x=2$
Now, for values of $x$ close to $\frac{2}{7}$ and to the left of $\frac{2}{7}, f^{\prime}(x)>0$. Also, for values of $x$ close to $\frac{2}{7}$ and to the right of $\frac{2}{7}, f^{\prime}(x)<0$.
Thus, $x=\frac{2}{7}$ is the point of local maxima.
Now, for values of $x$ close to 2 and to the left of $2, f^{\prime}(x)<0$. Also, for values of $x$ close to 2 and to the right of $2, f^{\prime}(x)>0$.
Thus, $x=2$ is the point of local minima.
Now, as the value of $x$ varies through $-1, f^{\prime}(x)$ does not changes its sign.
Thus, $x=-1$ is the point of inflexion.
11. Find the absolute maximum and minimum values of the function $f$ given by
$ f(x)=\cos ^{2} x+\sin x, x \in[0, \pi] $
Show Answer
Solution
$ \begin{aligned} f(x) & =\cos ^{2} x+\sin x \\ f^{\prime}(x) & =2 \cos x(-\sin x)+\cos x \\ & =-2 \sin x \cos x+\cos x \end{aligned} $
Now, $f^{\prime}(x)=0$
$\Rightarrow 2 \sin x \cos x=\cos x \Rightarrow \cos x(2 \sin x-1)=0$
$\Rightarrow \sin x=\frac{1}{2}$ or $\cos x=0$
$\Rightarrow x=\frac{\pi}{6}$, or $\frac{\pi}{2}$ as $x \in[0, \pi]$
Now, evaluating the value of $f$ at critical points $x=\frac{\pi}{2}$ and $x=\frac{\pi}{6}$ and at the end points of
the interval $[0, \pi]$ (i.e., at $x=0$ and $x=\pi$ ), we have: $f(\frac{\pi}{6})=\cos ^{2} \frac{\pi}{6}+\sin \frac{\pi}{6}=(\frac{\sqrt{3}}{2})^{2}+\frac{1}{2}=\frac{5}{4}$
$f(0)=\cos ^{2} 0+\sin 0=1+0=1$
$f(\pi)=\cos ^{2} \pi+\sin \pi=(-1)^{2}+0=1$
$f(\frac{\pi}{2})=\cos ^{2} \frac{\pi}{2}+\sin \frac{\pi}{2}=0+1=1$
Hence, the absolute maximum value of $f$ is $\frac{5}{4}$ occurring at $x=\frac{\pi}{6}$ and the absolute minimum value of $f$ is 1 occurring at $x=0, \frac{\pi}{2}$, and $\pi$.
12. Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius $r$ is $\frac{4 r}{3}$.
Show Answer
Solution
A sphere of fixed radius $(r)$ is given.
Let $R$ and $h$ be the radius and the height of the cone respectively.
The volume $(V)$ of the cone is given by,
$V=\frac{1}{3} \pi R^{2} h$
Now, from the right triangle $B C D$, we have:
$BC=\sqrt{r^{2}-R^{2}}$
$\square h=r+\sqrt{r^{2}-R^{2}}$
$ \begin{aligned} & \therefore V=\frac{1}{3} \pi R^{2}(r+\sqrt{r^{2}-R^{2}})=\frac{1}{3} \pi R^{2} r+\frac{1}{3} \pi R^{2} \sqrt{r^{2}-R^{2}} \\ & \begin{aligned} \therefore \frac{d V}{d R} & =\frac{2}{3} \pi R r+\frac{2 \pi}{3} \pi R \sqrt{r^{2}-R^{2}}+\frac{R^{2}}{3} \cdot \frac{(-2 R)}{2 \sqrt{r^{2}-R^{2}}} \\ & =\frac{2}{3} \pi R r+\frac{2 \pi}{3} \pi R \sqrt{r^{2}-R^{2}}-\frac{R^{3}}{3 \sqrt{r^{2}-R^{2}}} \\ & =\frac{2}{3} \pi R r+\frac{2 \pi R(r^{2}-R^{2})-\pi R^{3}}{3 \sqrt{r^{2}-R^{2}}} \\ & =\frac{2}{3} \pi R r+\frac{2 \pi R r^{2}-3 \pi R^{3}}{3 \sqrt{r^{2}-R^{2}}} \end{aligned} \end{aligned} $
Now, $\frac{d V}{d R^{2}}=0$
$\Rightarrow \frac{2 \pi r R}{3}=\frac{3 \pi R^{3}-2 \pi R r^{2}}{3 \sqrt{r^{2}-R^{2}}}$
$\Rightarrow 2 r \sqrt{r^{2}-R^{2}}=3 R^{2}-2 r^{2}$
$\Rightarrow 4 r^{2}(r^{2}-R^{2})=(3 R^{2}-2 r^{2})^{2}$
$\Rightarrow 4 r^{4}-4 r^{2} R^{2}=9 R^{4}+4 r^{4}-12 R^{2} r^{2}$
$\Rightarrow 9 R^{4}-8 r^{2} R^{2}=0$
$\Rightarrow 9 R^{2}=8 r^{2}$
$\Rightarrow R^{2}=\frac{8 r^{2}}{9}$
Now, $\frac{d^{2} V}{d R^{2}}=\frac{2 \pi r}{3}+\frac{3 \sqrt{r^{2}-R^{2}}(2 \pi r^{2}-9 \pi R^{2})-(2 \pi R r^{2}-3 \pi R^{3})(-6 R) \frac{1}{2 \sqrt{r^{2}-R^{2}}}}{9(r^{2}-R^{2})}$
$ =\frac{2 \pi r}{3}+\frac{3 \sqrt{r^{2}-R^{2}}(2 \pi r^{2}-9 \pi R^{2})+(2 \pi R r^{2}-3 \pi R^{3})(3 R) \frac{1}{2 \sqrt{r^{2}-R^{2}}}}{9(r^{2}-R^{2})} $
Now, when $R^{2}=\frac{8 r^{2}}{9}$, it can be shown that $\frac{d^{2} V}{d R^{2}}<0$.
$\square$ The volume is the maximum when
$ R^{2}=\frac{8 r^{2}}{9} $
When $R^{2}=\frac{8 r^{2}}{9}$, height of the cone $=r+\sqrt{r^{2}-\frac{8 r^{2}}{9}}=r+\sqrt{\frac{r^{2}}{9}}=r+\frac{r}{3}=\frac{4 r}{3}$.
Hence, it can be seen that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius $r$ is $\frac{4 r}{3}$.
13. Let $f$ be a function defined on $[a, b]$ such that $f^{\prime}(x)>0$, for all $x \in(a, b)$. Then prove that $f$ is an increasing function on $(a, b)$.
Show Answer
Solution
Let us take any 2 points $\mathrm{c}_1$ and $\mathrm{c}_2$ such that
${\mathrm{c} _1, \mathrm{c} _2} \in(\mathrm{a}, \mathrm{b})$ and
$\mathrm{c} _2=\mathrm{c} _1+\mathrm{h}$,
where $\mathrm{h} \rightarrow \mathrm{O}$
Now, $f^{\prime}\left(c _1\right)=\lim _{h \rightarrow 0} \frac{f\left(c _1+h\right)-f\left(c _1\right)}{h}=\frac{f\left(c _2\right)-f\left(c _1\right)}{c _2-c _1}$
Now, it is given that $\mathrm{f}^{\prime}(\mathrm{x})>0 \quad \forall \mathrm{x} \in(\mathrm{a}, \mathrm{b})$. Therefore, $\mathrm{f}^{\prime}\left(\mathrm{c}_1\right)>0$
$\Rightarrow \frac{\mathrm{f}\left(\mathrm{c}_2\right)-\mathrm{f}\left(\mathrm{c}_1\right)}{\mathrm{c}_2-\mathrm{c}_1}>\mathrm{O}$
From the above fraction, we can conclude that:
1. For $\mathrm{c}_2>\mathrm{c}_1, \mathrm{f}\left(\mathrm{c}_2\right)>\mathrm{f}\left(\mathrm{c}_1\right)$
2. For $c_1>c_2, f\left(c_1\right)>f\left(c_2\right)$
Hence, $\mathrm{f}(\mathrm{x})$ is an increasing function in $(\mathrm{a}, \mathrm{b})$.
14. Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius $R$ is $\frac{2 R}{\sqrt{3}}$. Also find the maximum volume.
Show Answer
Solution
A sphere of fixed radius $(R)$ is given.
Let $r$ and $h$ be the radius and the height of the cylinder respectively.
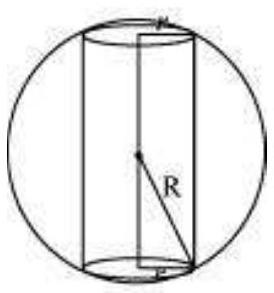
From the given figure, we have $h=2 \sqrt{R^{2}-r^{2}}$.
The volume $(V)$ of the cylinder is given by,
$ \begin{aligned} & V=\pi r^{2} h=2 \pi r^{2} \sqrt{R^{2}-r^{2}} \\ & \begin{aligned} \therefore \frac{d V}{d r} & =4 \pi r \sqrt{R^{2}-r^{2}}+\frac{2 \pi r^{2}(-2 r)}{2 \sqrt{R^{2}-r^{2}}} \\ & =4 \pi r \sqrt{R^{2}-r^{2}}-\frac{2 \pi r^{3}}{\sqrt{R^{2}-r^{2}}} \\ & =\frac{4 \pi r(R^{2}-r^{2})-2 \pi r^{3}}{\sqrt{R^{2}-r^{2}}} \\ & =\frac{4 \pi r R^{2}-6 \pi r^{3}}{\sqrt{R^{2}-r^{2}}} \end{aligned} \end{aligned} $
Now, $\frac{d V}{d r}=0 \Rightarrow 4 \pi r R^{2}-6 \pi r^{3}=0$
$\Rightarrow r^{2}=\frac{2 R^{2}}{3}$
Now, $\frac{d^{2} V}{d r^{2}}=\frac{\sqrt{R^{2}-r^{2}}(4 \pi R^{2}-18 \pi r^{2})-(4 \pi r R^{2}-6 \pi r^{3}) \frac{(-2 r)}{2 \sqrt{R^{2}-r^{2}}}}{(R^{2}-r^{2})}$
$ \begin{aligned} & =\frac{(R^{2}-r^{2})(4 \pi R^{2}-18 \pi r^{2})+r(4 \pi r R^{2}-6 \pi r^{3})}{(R^{2}-r^{2})^{\frac{3}{2}}} \\ & =\frac{4 \pi R^{4}-22 \pi r^{2} R^{2}+12 \pi r^{4}+4 \pi r^{2} R^{2}}{(R^{2}-r^{2})^{\frac{3}{2}}} \end{aligned} $
Now, it can be observed that at $r^{2}=\frac{2 R^{2}}{3}, \frac{d^{2} V}{d r^{2}}<0$.
$\square$ The volume is the maximum when $r^{2}=\frac{2 R^{2}}{3}$.
When $r^{2}=\frac{2 R^{2}}{3}$, the height of the cylinder is $2 \sqrt{R^{2}-\frac{2 R^{2}}{3}}=2 \sqrt{\frac{R^{2}}{3}}=\frac{2 R}{\sqrt{3}}$.
Hence, the volume of the cylinder is the maximum when the height of the cylinder is $\frac{2 R}{\sqrt{3}}$.
15. Show that height of the cylinder of greatest volume which can be inscribed in a right circular cone of height $h$ and semi vertical angle $\alpha$ is one-third that of the cone and the greatest volume of cylinder is $\frac{4}{27} \pi h^{3} \tan ^{2} \alpha$.
Show Answer
Solution
The given right circular cone of fixed height $(h)$ and semi-vertical angle ( $a$ ) can be drawn as:
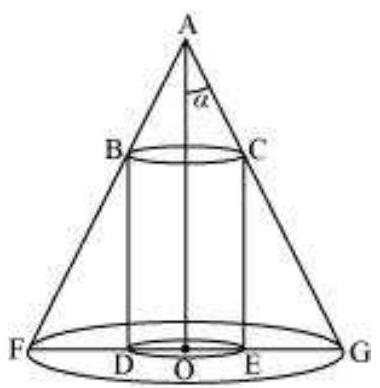
Here, a cylinder of radius $R$ and height $H$ is inscribed in the cone.
Then, $\square GAO=a, OG=r, OA=h, OE=R$, and $CE=H$.
We have,
$r=h \tan a$
Now, since $\triangle AOG$ is similar to $\triangle CEG$, we have:
$\frac{AO}{OG}=\frac{CE}{EG}$
$\Rightarrow \frac{h}{r}=\frac{H}{r-R} \quad[EG=OG-OE]$
$\Rightarrow H=\frac{h}{r}(r-R)=\frac{h}{h \tan \alpha}(h \tan \alpha-R)=\frac{1}{\tan \alpha}(h \tan \alpha-R)$
Now, the volume $(V)$ of the cylinder is given by,
$V=\pi R^{2} H=\frac{\pi R^{2}}{\tan \alpha}(h \tan \alpha-R)=\pi R^{2} h-\frac{\pi R^{3}}{\tan \alpha}$
$\therefore \frac{d V}{d R}=2 \pi R h-\frac{3 \pi R^{2}}{\tan \alpha}$
Now, $\frac{d V}{d R}=0$
$\Rightarrow 2 \pi R h=\frac{3 \pi R^{2}}{\tan \alpha}$
$\Rightarrow 2 h \tan \alpha=3 R$
$\Rightarrow R=\frac{2 h}{3} \tan \alpha$
Now, $\frac{d^{2} V}{d R^{2}}=2 \pi h-\frac{6 \pi R}{\tan \alpha}$
And, for $R=\frac{2 h}{3} \tan \alpha$, we have:
$\frac{d^{2} V}{d R^{2}}=2 \pi h-\frac{6 \pi}{\tan \alpha}(\frac{2 h}{3} \tan \alpha)=2 \pi h-4 \pi h=-2 \pi h<0$
$\square$ By second derivative test, the volume of the cylinder is the greatest when
$R=\frac{2 h}{3} \tan \alpha$.
When $R=\frac{2 h}{3} \tan \alpha, H=\frac{1}{\tan \alpha}(h \tan \alpha-\frac{2 h}{3} \tan \alpha)=\frac{1}{\tan \alpha}(\frac{h \tan \alpha}{3})=\frac{h}{3}$.
Thus, the height of the cylinder is one-third the height of the cone when the volume of the cylinder is the greatest.
Now, the maximum volume of the cylinder can be obtained as:
$\pi(\frac{2 h}{3} \tan \alpha)^{2}(\frac{h}{3})=\pi(\frac{4 h^{2}}{9} \tan ^{2} \alpha)(\frac{h}{3})=\frac{4}{27} \pi h^{3} \tan ^{2} \alpha$
Hence, the given result is proved.
16. A cylindrical tank of radius $10 m$ is being filled with wheat at the rate of 314 cubic metre per hour. Then the depth of the wheat is increasing at the rate of
(A) $1 m / h$
(B) $0.1 m / h$
(C) $1.1 m / h$
(D) $0.5 m / h$
Show Answer
Solution
Let $r$ be the radius of the cylinder.
Then, volume $(V)$ of the cylinder is given by,
$V=\pi(\text{ radius })^{2} \times$ height
$ \begin{matrix} =\pi(10)^{2} h & (\text{ radius }=10 m) \\ =100 \pi h \end{matrix} $
Differentiating with respect to time $t$, we have:
$\frac{d V}{d t}=100 \pi \frac{d h}{d t}$
The tank is being filled with wheat at the rate of 314 cubic metres per hour.
$ \square \frac{d V}{d t}=314 m^{3} / h $
Thus, we have:
$ \begin{aligned} & 314=100 \pi \frac{d h}{d t} \\ & \Rightarrow \frac{d h}{d t}=\frac{314}{100(3.14)}=\frac{314}{314}=1 \end{aligned} $
Hence, the depth of wheat is increasing at the rate of $1 m / h$.
The correct answer is $A$.
Summary
If a quantity y varies with another quantity x, satisfying some rule y= f(x),then $ \frac{dy}{dx}or (f’(x)) $ represents the rate of change of y with respect to x and $ \frac{dy}dx _{x=x0} or(f’(x_0)) $ $\text{represents the rate of change of y with respect to x at}$ $ x=x+0 $ If two variables $x$ and $y$ are varying with respect to another variable $t$, i.e., if $x=f(t)$ and $y=g(t)$, then by Chain Rule
$$ \frac{d y}{d x}=\frac{d y}{d t} / \frac{d x}{d t}, \text { यदि } \frac{d x}{d t} \neq 0 $$
A function $f$ is said to be
(a) increasing on an interval $(a, b)$ if $x_1<x_2$ in
$(a, b) \Rightarrow f(x_1)<f(x_2)$ for all $x_1, x_2 \in(a, b)$.
Alternatively, if $f^{\prime}(x) \geq 0$ for each $x$ in $(a, b)$
(b) decreasing on $(a, b)$ if
$ x_1<x_2 \text{ in }(a, b) \Rightarrow f(x_1)>f(x_2) \text{ for all } x_1, x_2 \in(a, b) \text{. } $
(c) constant in $(a, b)$, if $f(x)=c$ for all $x \in(a, b)$, where $c$ is a constant.
- A point $c$ in the domain of a function $f$ at which either $f^{\prime}(c)=0$ or $f$ is not differentiable is called a critical point of $f$.
- First Derivative Test Let $f$ be a function defined on an open interval I. Let $f$ be continuous at a critical point $c$ in $I$. Then
(i) If $f^{\prime}(x)$ changes sign from positive to negative as $x$ increases through $c$, i.e., if $f^{\prime}(x)>0$ at every point sufficiently close to and to the left of $c$, and $f^{\prime}(x)<0$ at every point sufficiently close to and to the right of $c$, then $c$ is a point of local maxima.
(ii) If $f^{\prime}(x)$ changes sign from negative to positive as $x$ increases through $c$, i.e., if $f^{\prime}(x)<0$ at every point sufficiently close to and to the left of $c$, and $f^{\prime}(x)>0$ at every point sufficiently close to and to the right of $c$, then $c$ is a point of local minima.
(iii) If $f^{\prime}(x)$ does not change sign as $x$ increases through $c$, then $c$ is neither a point of local maxima nor a point of local minima. Infact, such a point is called point of inflexion.
- Second Derivative Test Let $f$ be a function defined on an interval I and $c \in I$. Let $f$ be twice differentiable at $c$. Then
(i) $x=c$ is a point of local maxima if $f^{\prime}(c)=0$ and $f^{\prime \prime}(c)<0$ The values $f(c)$ is local maximum value of $f$.
(ii) $x=c$ is a point of local minima if $f^{\prime}(c)=0$ and $f^{\prime \prime}(c)>0$ In this case, $f(c)$ is local minimum value of $f$.
(iii) The test fails if $f^{\prime}(c)=0$ and $f^{\prime \prime}(c)=0$.
In this case, we go back to the first derivative test and find whether $c$ is a point of maxima, minima or a point of inflexion.
- Working rule for finding absolute maxima and/or absolute minima
Step 1: Find all critical points of $f$ in the interval, i.e., find points $x$ where either $f^{\prime}(x)=0$ or $f$ is not differentiable.
Step 2:Take the end points of the interval.
Step 3: At all these points (listed in Step 1 and 2), calculate the values of $f$.
Step 4: Identify the maximum and minimum values of $f$ out of the values calculated in Step 3. This maximum value will be the absolute maximum value of $f$ and the minimum value will be the absolute minimum value of $f$.
With the Calculus as a key, Mathematics can be successfully applied to the explanation of the course of Nature - WHITEHEAD
6.1 भूमिका (Introduction)
अध्याय 5 में हमने संयुक्त फलनों, प्रतिलोम त्रिकोणमितीय फलनों, अस्पष्ट फलनों, चरघातांकीय फलनों और लघुघातांकीय फलनों का अवकलज ज्ञात करना सीखा है। प्रस्तुत अध्याय में, हम गणित की विभिन्न शाखाओं में अवकलज के अनुप्रयोग का अध्ययन करेंगे यथा इंजिनियरिंग, विज्ञान, सामाजिक विज्ञान और कई दूसरे क्षेत्र। उदाहरण के लिए हम सीखेंगे कि किस प्रकार अवकलज का उपयोग (i) राशियों के परिवर्तन की दर ज्ञात करने में, (ii) किसी बिंदु पर स्पर्श रेखा तथा अभिलंब की समीकरण ज्ञात करने में, (iii) एक फलन के आलेख पर वर्तन बिंदु ज्ञात करने में, जो हमें उन बिंदुओं को ज्ञात करने में सहायक होता है जिन पर फलन का अधिकतम या न्यूनतम मान होता है। हम उन अंतरालों को ज्ञात करने में भी अवकलज का उपयोग करेंगे, जिनमें एक फलन वर्धमान या ह्रासमान होता है। अंततः हम कुछ राशियों के सन्निकट मान प्राप्त करने में अवकलज प्रयुक्त करेंगे।
6.2 राशियों के परिवर्तन की दर (Rate of Change of Quantities)
पुन: स्मरण कीजिए कि अवकलज $\frac{d s}{d t}$ से हमारा तात्पर्य समय अंतराल $t$ के सापेक्ष दूरी $s$ के परिवर्तन की दर से है। इसी प्रकार, यदि एक राशि $y$ एक दूसरी राशि $x$ के सापेक्ष किसी नियम $y=f(x)$ को संतुष्ट करते हुए परिवर्तित होती है तो $\frac{d y}{d x}\left(\right.$ या $\left.f^{\prime}(x)\right), x$ के सापेक्ष $y$ के परिवर्तन की दर को प्रदर्शित करता है और $\left.\frac{d y}{d x}\right] _{x=x _{0}}$ (या $\left. f^{\prime}\left(x _{0}\right)\right) x = x _{0}$ पर) $x$ के सापेक्ष $y$ की परिवर्तन की दर को प्रदर्शित करता है।
इसके अतिरिक्त, यदि दो राशियाँ $x$ और $y, t$ के सापेक्ष परिवर्तित हो रही हों अर्थात् $x=f(t)$ और $y=g(t)$ है तब शृंखला नियम से
$$ \frac{d y}{d x}=\frac{d y}{d t} / \frac{d x}{d t}, \text { यदि } \frac{d x}{d t} \neq 0 \text { प्राप्त होता है। } $$
इस प्रकार, $x$ के सापेक्ष $y$ के परिवर्तन की दर का परिकलन $t$ के सापेक्ष $y$ और $x$ के परिवर्तन की दर का प्रयोग करके किया जा सकता है ।
आइए हम कुछ उदाहरणों पर विचार करें।
उदाहरण 1 वृत्त के क्षेत्रफल के परिवर्तन की दर इसकी त्रिज्या $r$ के सापेक्ष ज्ञात कीजिए जब $r=5 \mathrm{~cm}$ है।
हल त्रिज्या $r$ वाले वृत्त का क्षेत्रफल $\mathrm{A}=\pi r^{2}$ से दिया जाता है। इसलिए, $r$ के सापेक्ष $\mathrm{A}$ के परिवर्तन की दर $\frac{d \mathrm{~A}}{d r}=\frac{d}{d r}\left(\pi r^{2}\right)=2 \pi r$ से प्राप्त है। जब $r=5 \mathrm{~cm}$ तो $\frac{d \mathrm{~A}}{d r}=10 \pi$ है। अतः वृत्त का क्षेत्रफल $10 \pi \mathrm{cm}^{2} / \mathrm{cm}$ की दर से बदल रहा है
उदाहरण 2 एक घन का आयतन $9 \mathrm{~cm}^{3} / \mathrm{s}$ की दर से बढ़ रहा है। यदि इसके कोर की लंबायीं $10 \mathrm{~cm}$ है तो इसके पृष्ठ का क्षेत्रफल किस दर से बढ़ रहा है।
हल मान लीजिए कि घन की एक कोर की लंबायों $x \mathrm{~cm}$ है। घन का आयतन $\mathrm{V}$ तथा घन के पृष्ठ का क्षेत्रफल $\mathrm{S}$ है। तब, $\mathrm{V}=x^{3}$ और $\mathrm{S}=6 x^{2}$, जहाँ $x$ समय $t$ का फलन है।
अब $ \qquad \frac{d \mathrm{~V}}{d t}=9 \mathrm{~cm}^{3} / \mathrm{s} \text { (दिया है) }$
इसलिए $9= \qquad \frac{d \mathrm{~V}}{d t}=\frac{d}{d t}\left(x^{3}\right)=\frac{d}{d x}\left(x^{3}\right) \cdot \frac{d x}{d t} \text { (शृंखला नियम से) }$
$ या \qquad =3 x^{2} \cdot \frac{d x}{d t} $
$ अब \qquad \frac{d x}{d t}=\frac{3}{x^{2}} \tag{1} $
$$ \begin{array}{rlr} \frac{d S}{d t} & =\frac{d}{d t}\left(6 x^{2}\right)=\frac{d}{d x}\left(6 x^{2}\right) \cdot \frac{d x}{d t} & \text { (शृंखला नियम से) } \\ & =12 x \cdot\left(\frac{3}{x^{2}}\right)=\frac{36}{x} & \text { ((1) के प्रयोग से) } \end{array} $$
$ अत:, जब \qquad x=10 \mathrm{~cm}, \frac{d S}{d t}=3.6 \mathrm{~cm}^{2} / \mathrm{s} $
उदाहरण 3 एक स्थिर झील में एक पत्थर डाला जाता है और तरंगें वृत्तों में $4 \mathrm{~cm} / \mathrm{s}$ की गति से चलती हैं। जब वृत्ताकार तरंग की त्रिज्या $10 \mathrm{~cm}$ है, तो उस क्षण, घिरा हुआ क्षेत्रफल कितनी तेजी से बढ़ रहा है?
हल त्रिज्या $r$ वाले वृत्त का क्षेत्रफल $\mathrm{A}=\pi r^{2}$ से दिया जाता है। इसलिए समय $t$ के सापेक्ष क्षेत्रफल $\mathrm{A}$ के परिवर्तन की दर है
$$ \frac{d \mathrm{~A}}{d t}=\frac{d}{d t}\left(\pi r^{2}\right)=\frac{d}{d r}\left(\pi r^{2}\right) \cdot \frac{d r}{d t}=2 \pi r \frac{d r}{d t} $$
$ यह दिया गया है कि \qquad \frac{d r}{d t}=4 \mathrm{~cm} $
$ इसलिए जब \qquad r=10 \mathrm{~cm} $ $ \frac{d \mathrm{~A}}{d t}=2 \pi(10)(4)=80 \pi $
अतः जब $r=10 \mathrm{~cm}$ तब वृत्त से घिरे क्षेत्र का क्षेत्रफल $80 \pi \mathrm{cm}^{2} / \mathrm{s}$ की दर से बढ़ रहा है।
टिप्पणी $x$ का मान बढ़ने से यदि $y$ का मान बढ़ता है तो $\frac{d y}{d x}$ धनात्मक होता है और $x$ का मान बढ़ने से यदि $y$ का मान घटता है, तो $\frac{d y}{d x}$ ॠणात्मक होता है।
उदाहरण 4 किसी आयत की लंबायों $x, 3 \mathrm{~cm} / \mathrm{min}$ की दर से घट रही है और चौड़ाई $y, 2 \mathrm{~cm} / \mathrm{min}$ की दर से बढ़ रही है। जब $x=10 \mathrm{~cm}$ और $y=6 \mathrm{~cm}$ है तब आयत के (a) परिमाप और (b) क्षेत्रफल में परिवर्तन की दर ज्ञात कीजिए।
हल क्योंकि समय के सापेक्ष लंबायों $x$ घट रही है और चौड़ाई $y$ बढ़ रही है तो हम पाते हैं कि
$$ \frac{d x}{d t}=-3 \mathrm{~cm} / \mathrm{min} \text { और } \frac{d y}{d t}=2 \mathrm{~cm} / \mathrm{min} $$
(a) आयत का परिमाप $\mathrm{P}$ से प्रदत्त है, अर्थात्
$$ \mathrm{P}=2(x+y) $$
$ इसलिए \qquad \frac{d \mathrm{P}}{d t}=2\left(\frac{d x}{d t}+\frac{d y}{d t}\right)=2(-3+2)=-2 \mathrm{~cm} / \mathrm{min} $
(b) आयत का क्षेत्रफल $\mathrm{A}$ से प्रदत्त है यथा
$ \mathrm{A}=x \cdot y $
इसलिए $ \begin{aligned} \frac{d \mathrm{~A}}{d t} & =\frac{d x}{d t} \cdot y+x \cdot \frac{d y}{d t} \\ & =-3(6)+10(2)(\text { क्योंकि } x=10 \mathrm{~cm} \text { और } y=6 \mathrm{~cm}) \\ & =2 \mathrm{~cm}^{2} / \mathrm{min} \end{aligned} $
उदाहरण 5 किसी वस्तु की $x$ इकाइयों के उत्पादन में कुल लागत $\mathrm{C}(x)$ रुपये में
$$ C(x)=0.005 x^{3}-0.02 x^{2}+30 x+5000 $$
से प्रदत्त है। सीमांत लागत ज्ञात कीजिए जब 3 इकाई उत्पादित की जाती है। जहाँ सीमांत लागत (marginal cost या MC) से हमारा अभिप्राय किसी स्तर पर उत्पादन के संपूर्ण लागत में तात्कालिक परिवर्तन की दर से है।
हल क्योंकि सीमांत लागत उत्पादन के किसी स्तर पर $x$ इकाई के सापेक्ष संपूर्ण लागत के परिवर्तन की दर है। हम पाते हैं कि
$$ \begin{array}{lll} \text { सीमांत लागत } & \mathrm{MC} & =\frac{d \mathrm{C}}{d x}=0.005\left(3 x^{2}\right)-0.02(2 x)+30 \\ \text { जब } x=3 \text{ है तब} & \mathrm{MC} & =0.015\left(3^{2}\right)-0.04(3)+30 \\ & & =0.135-0.12+30=30.015 \end{array} $$
अतः अभीष्ट सीमांत लागत अर्थात लागत प्रति इकाई Rs 30.02 (लगभग) है।
उदाहरण 6 किसी उत्पाद की $x$ इकाइयों के विक्रय से प्राप्त कुल आय रुपये में $\mathrm{R}(x)=3 x^{2}+36 x$ +5 से प्रदत्त है। जब $x=5$ हो तो सीमांत आय ज्ञात कीजिए। जहाँ सीमांत आय (marginal revenue or MR) से हमारा अभिप्राय किसी क्षण विक्रय की गई वस्तुओं के सापेक्ष संपूर्ण आय के परिवर्तन की दर से है।
हल क्योंकि सीमांत आय किसी क्षण विक्रय की गई वस्तुओं के सापेक्ष आय परिवर्तन की दर होती है। हम जानते हैं कि
$ \begin{aligned} सीमांत आय \qquad \mathrm{MR}=\frac{d \mathrm{R}}{d x}=6 x+36 \end{aligned} $ $ \begin{aligned} जब \qquad x=5 है तब \mathrm{MR}=6(5)+36=66 \end{aligned} $
अतः अभीष्ट सीमांत आय अर्थात आय प्रति इकाई Rs 66 है।
प्रश्नावली 6.1
1. वृत्त के क्षेत्रफल के परिवर्तन की दर इसकी त्रिज्या $r$ के सापेक्ष ज्ञात कीजिए जबकि
(a) $r=3 \mathrm{~cm}$ है। (b) $r=4 \mathrm{~cm}$ है।
Show Answer
#missing2. एक घन का आयतन $8 \mathrm{~cm}^{3} / \mathrm{s}$ की दर से बढ़ रहा है। पृष्ठ क्षेत्रफल किस दर से बढ़ रहा है जबकि इसके किनारे की लंबायीं $12 \mathrm{~cm}$ है।
Show Answer
#missing3. एक वृत्त की त्रिज्या समान रूप से $3 \mathrm{~cm} / \mathrm{s}$ की दर से बढ़ रही है। ज्ञात कीजिए कि वृत्त का क्षेत्रफल किस दर से बढ़ रहा है जब त्रिज्या $10 \mathrm{~cm}$ है।
Show Answer
#missing4. एक परिवर्तनशील घन का किनारा $3 \mathrm{~cm} / \mathrm{s}$ की दर से बढ़ रहा है। घन का आयतन किस दर से बढ़ रहा है जबकि किनारा $10 \mathrm{~cm}$ लंबा है?
Show Answer
#missing5. एक स्थिर झील में एक पत्थर डाला जाता है ओर तरंगें वृत्तों में $5 \mathrm{~cm} / \mathrm{s}$ की गति से चलती हैं। जब वृत्ताकार तरंग की त्रिज्या $8 \mathrm{~cm}$ है तो उस क्षण, घिरा हुआ क्षेत्रफल किस दर से बढ़ रहा है?
Show Answer
#missing6. एक वृत्त की त्रिज्या $0.7 \mathrm{~cm} / \mathrm{s}$ की दर से बढ़ रही है। इसकी परिधि की वृद्धि की दर क्या है जब $r=4.9 \mathrm{~cm}$ है?
Show Answer
#missing7. एक आयत की लंबायीं $x, 5 \mathrm{~cm} / \mathrm{min}$ की दर से घट रही है और चौड़ाई $y, 4 \mathrm{~cm} / \mathrm{min}$ की दर से बढ़ रही है। जब $x=8 \mathrm{~cm}$ और $y=6 \mathrm{~cm}$ हैं तब आयत के (a) परिमाप (b) क्षेत्रफल के परिवर्तन की दर ज्ञात कीजिए।
Show Answer
#missing8. एक गुब्बारा जो सदैव गोलाकार रहता है, एक पंप द्वारा $900 \mathrm{~cm}^{3}$ गैस प्रति सेकंड भर कर फुलाया जाता है। गुब्बारे की त्रिज्या के परिवर्तन की दर ज्ञात कीजिए जब त्रिज्या $15 \mathrm{~cm}$ है।
Show Answer
#missing9. एक गुब्बारा जो सदैव गोलाकार रहता है, की त्रिज्या परिवर्तनशील है। त्रिज्या के सापेक्ष आयतन के परिवर्तन की दर ज्ञात कीजिए जब त्रिज्या $10 \mathrm{~cm}$ है।
Show Answer
#missing10. एक $5 \mathrm{~m}$ लंबी सीढ़ी दीवार के सहारे झुकी है। सीढ़ी का नीचे का सिरा, जमीन के अनुदिश, दीवार से दूर $2 \mathrm{~cm} / \mathrm{s}$ की दर से खींचा जाता है। दीवार पर इसकी ऊँचाई किस दर से घट रही है जबकि सीढ़ी के नीचे का सिरा दीवार से $4 \mathrm{~m}$ दूर है?
Show Answer
#missing11. एक कण वक्र $6 y=x^{3}+2$ के अनुगत गति कर रहा हैं। वक्र पर उन बिंदुओं को ज्ञात कीजिए जबकि $x$-निर्देशांक की तुलना में $y$-निर्देशांक 8 गुना तीव्रता से बदल रहा है।
Show Answer
#missing12. हवा के एक बुलबुले की त्रिज्या $\frac{1}{2} \mathrm{~cm} / \mathrm{s}$ की दर से बढ़ रही है। बुलबुले का आयतन किस दर से बढ़ रहा है जबकि त्रिज्या $1 \mathrm{~cm}$ है?
Show Answer
#missing13. एक गुब्बारा, जो सदैव गोलाकार रहता है, का परिवर्तनशील व्यास $\frac{3}{2}(2 x+1)$ है। $x$ के सापेक्ष आयतन के परिवर्तन की दर ज्ञात कीजिए।
Show Answer
#missing14. एक पाइप से रेत $12 \mathrm{~cm}^{3} / \mathrm{s}$ की दर से गिर रही है। गिरती रेत जमीन पर एक ऐसा शंकु बनाती है जिसकी ऊँचाई सदैव आधार की त्रिज्या का छठा भाग है। रेत से बने के शंकु की ऊँचाई किस दर से बढ़ रही है जबकि ऊँचाई $4 \mathrm{~cm}$ है?
Show Answer
#missing15. एक वस्तु की $x$ इकाइयों के उत्पादन से संबंध कुल लागत $\mathrm{C}(x)$ (रुपये में)
$$ C(x)=0.007 x^{3}-0.003 x^{2}+15 x+4000 $$
से प्रदत्त है। सीमांत लागत ज्ञात कीजिए जबकि 17 इकाइयों का उत्पादन किया गया है।
Show Answer
#missing16. किसी उत्पाद की $x$ इकाइयों के विक्रय से प्राप्त कुल आय $\mathrm{R}(x)$ रुपयों में
$$ R(x)=13 x^{2}+26 x+15 $$
से प्रदत्त है। सीमांत आय ज्ञात कीजिए जब $x=7$ है।
Show Answer
#missingप्रश्न 17 तथा 18 में सही उत्तर का चयन कीजिए:
17. एक वृत्त की त्रिज्या $r=6 \mathrm{~cm}$ पर $r$ के सापेक्ष क्षेत्रफल में परिवर्तन की दर है:
(A) $10 \pi$
(B) $12 \pi$
(C) $8 \pi$
(D) $11 \pi$
Show Answer
#missing18. एक उत्पाद की $x$ इकाइयों के विक्रय से प्राप्त कुल आय रुपयों में
$\mathrm{R}(x)=3 x^{2}+36 x+5$ से प्रदत्त है। जब $x=15$ है तो सीमांत आय है:
(A) 116
(B) 96
(C) 90
(D) 126
Show Answer
#missing6.3 वर्धमान (Increasing) और ह्रासमान (Decreasing ) फलन
इस अनुच्छेद में हम अवकलन का प्रयोग करके यह ज्ञात करेंगे कि फलन वर्धमान है या ह्रासमान या इनमें से कोई नहीं है।
$f(x)=x^{2}, x \in \mathbf{R}$ द्वारा प्रदत्त फलन $f$ पर विचार कीजिए। इस फलन का आलेख आकृति 6.1 में दिया गया है।
मूल बिंदु के बायों ओर का मान
| $x$ | $f(x)=x^{2}$ |
|---|---|
| -2 | 4 |
| $-\frac{3}{2}$ | $\frac{9}{4}$ |
| -1 | 1 |
| $-\frac{1}{2}$ | $\frac{1}{4}$ |
| 0 | 0 |
जैसे जैसे हम बाँए से दाँए ओर बढ़ते जाते हैं तो आलेख की ऊँचाई घटती जाती है। मूल बिंदु के दायीं ओर का मान

जैसे जैसे हम बाँए से दाँए ओर बढ़ते जाते है तो आलेख की ऊँचाई बढ़ती जाती है।
| $x$ | $f(x)=x^{2}$ |
|---|---|
| 0 | 0 |
| $\frac{1}{2}$ | $\frac{1}{4}$ |
| 1 | 1 |
| $\frac{3}{2}$ | $\frac{9}{4}$ |
| 2 | 4 |
सर्वप्रथम मूल बिंदु के दायीं ओर के आलेख (आकृति 6.1) पर विचार करते हैं। यह देखिए कि आलेख के अनुदिश जैसे जैसे बाएँ से दाएँ ओर जाते हैं, आलेख की ऊँचाई लगातार बढ़ती जाती है। इसी कारण वास्तविक संख्याओं $x>0$ के लिए फलन वर्धमान कहलाता है।
अब मूल बिंदु के बायों ओर के आलेख पर विचार करते हैं। यहाँ हम देखते हैं कि जैसे जैसे आलेख के अनुदिश बाएँ से दाएँ की ओर जाते हैं, आलेख की ऊँचाई लगातार घटती जाती है। फलस्वरूप वास्तविक संख्याओं $x<0$ के लिए फलन ह्रासमान कहलाता है।
हम अब एक अंतराल में वर्धमान या ह्रासमान फलनों की निम्नलिखित विश्लेषणात्मक परिभाषा देंगे।
परिभाषा 1 मान लीजिए वास्तविक मान फलन $f$ के प्रांत में $\mathrm{I}$ एक अंतराल है। तब $f$
(i) अंतराल I में वर्धमान है, यदि I में $x _{1}<x _{2} \Rightarrow f\left(x _{1}\right)<f\left(x _{2}\right)$ सभी $x _{1}, x _{2} \in$ I के लिए
(ii) अंतराल I में ह्रासमान है, यदि I में $x _{1}<x _{2} \Rightarrow f\left(x _{1}\right)>f\left(x _{2}\right)$ सभी $x _{1}, x _{2} \in \mathrm{I}$ के लिए
(iii) अंतराल I में अचर है, यदि $f(x)=\mathrm{c}, x \in \mathrm{I}$ जहाँ $\mathrm{c}$ एक अचर है।
(iv) I में ह्रासमान है, यदि I में $x_1<x_2$ $ \Rightarrow f(x_1) \geq f(x_2)$ सभी $x_1, x_2 \in I$.
(v) सख्ती से I में ह्रासमान है, यदि I में $x_1<x_2$ $ \Rightarrow f(x_1)>f(x_2)$ सभी $x_1, x_2 \in I$.
इस प्रकार के फलनों का आलेखीय निरूपण आकृति 6.2 में देखिए।

निरंतर ह्रासमान फलन अब हम एक बिंदु पर वर्धमान या ह्रासमान फलन को परिभाषित करेंगे।
परिभाषा 2 मान लीजिए कि वास्तविक मानों के परिभाषित फलन $f$ के प्रांत में एक बिंदु $x _{0}$ है तब $x _{0}$ पर $f$ वर्धमान और ह्रासमान कहलाता है यदि $x _{0}$ को अंतर्विष्ट करने वाले एक ऐसे विवृत्त अंतराल I का अस्तित्व इस प्रकार है कि I में, $f$ क्रमशः
वर्धमान और ह्रासमान है आइए इस परिभाषा को वर्धमान फलन के लिए स्पष्ट करते हैं।
उदाहरण 7 दिखाइए कि प्रदत्त फलन $f(x)=7 x-3, \mathbf{R}$ पर एक वर्धमान फलन है।
हल मान लीजिए $\mathbf{R}$ में $x _{1}$ और $x _{2}$ कोई दो संख्याएँ हैं, तब
$$ \begin{aligned} x _{1}<x _{2} & \Rightarrow 7 x _{1}<7 x _{2} \\ & \Rightarrow 7 x _{1}-3<7 x _{2}-3 \\ & \Rightarrow f\left(x _{1}\right)<f\left(x _{2}\right) \end{aligned} $$
इस प्रकार, परिभाषा 1 से परिणाम निकलता है कि $\mathbf{R}$ पर $f$ एक वर्धमान फलन है।
अब हम वर्धमान और ह्रासमान फलनों के लिए प्रथम अवकलज परीक्षण प्रस्तुत करेंगे। इस परीक्षण की उपपत्ति में अध्याय 5 में अध्ययन की गई मध्यमान प्रमेय का प्रयोग करते हैं।
प्रमेय 1 मान लीजिए कि $f$ अंतराल $[a, b]$ पर संतत और विवृत्त अंतराल $(a, b)$ पर अवकलनीय है। तब
(a) $[a, b]$ में $f$ वर्धमान है यदि प्रत्येक $x \in(a, b)$ के लिए $f^{\prime}(x)>0$ है।
(b) $[a, b]$ में $f$ ह्रासमान है यदि प्रत्येक $x \in(a, b)$ के लिए $f^{\prime}(x)<0$ है।
(c) $[a, b]$ में $f$ एक अचर फलन है यदि प्रत्येक $x \in(a, b)$ के लिए $f^{\prime}(x)=0$ है।
उपपत्ति (a) मान लीजिए $x _{1}, x _{2} \in[a, b]$ इस प्रकार हैं कि $x _{1}<x _{2}$
तब मध्य मान प्रमेय से $x _{1}$ और $x _{2}$ के मध्य एक बिंदु $c$ का अस्तित्व इस प्रकार है कि
$$ f\left(x _{2}\right)-f\left(x _{1}\right)=f^{\prime}(c)\left(x _{2}-x _{1}\right) $$
$ \begin{array}{ll} अर्थात् \qquad f\left(x _{2}\right)-f\left(x _{1}\right)>0 & \left(\text { क्योंकि } f^{\prime}(c)>0\right) \\ \end{array} $
$ अर्थात् \qquad f(x_2)>f(x_1) $
$ इस प्रकार, हम देखते हैं, कि \qquad [a, b] \text { के सभी } x _{1}, x _{2} \text { के लिए } x _{1}<x _{2} \quad f\left(x _{1}\right) \quad f\left(x _{2}\right) $
अतः $[a, b]$ में $f$ एक वर्धमान फलन है।
भाग (b) और (c) की उपपत्ति इसी प्रकार है। पाठकों के लिए इसे अभ्यास हेतु छोड़ा जाता है।
टिप्पणी
इस सदंर्भ में एक अन्य सामान्य प्रमेय के अनुसार यदि किसी अंतराल के अंत्य बिंदुओं के अतिरिक्त $f^{\prime}(x)>0$ जहाँ $x$, अंतराल में कोई अवयव है और $f$ उस अंतराल में संतत है तब $f$ को वर्धमान कहते हैं। इसी प्रकार यदि किसी अंतराल के अंत्य बिंदुओं के सिवाय $f^{1}(x)$ $<0$ जहाँ $x$ अंतराल का कोई अवयव है और $f$ उस अंतराल में संतत है तब $f$ को ह्रासमान कहते हैं।
उदाहरण 8 दिखाइए कि प्रदत्त फलन $f$,
$\mathbf{R}$ पर वर्धमान फलन है।
$$ f(x)=x^{3}-3 x^{2}+4 x, x \in \mathbf{R} $$
हल ध्यान दीजिए कि
$$ \begin{aligned} f^{\prime}(x) & =3 x^{2}-6 x+4 \\ & =3\left(x^{2}-2 x+1\right)+1 \\ & =3(x-1)^{2}+1>0, \text { सभी } x \in \mathbf{R} \text { के लिए } \end{aligned} $$
इसलिए फलन $f, \mathbf{R}$ पर वर्धमान है।
उदाहरण 9 सिद्ध कीजिए कि प्रदत्त फलन $f(x)=\cos x$
(a) $(0, \pi)$ में ह्रासमान है
(b) $(\pi, 2 \pi)$, में वर्धमान है
(c) $(0,2 \pi)$ में न तो वर्धमान और न ही ह्रासमान है।
हल ध्यान दीजिए कि $f^{\prime}(x)=-\sin x$
(a) चूँकि प्रत्येक $x \in(0, \pi)$ के लिए $\sin x>0$, हम पाते हैं कि $f^{\prime}(x)<0$ और इसलिए $(0, \pi)$ में $f$ ह्रसमान है।
(b) चूँकि प्रत्येक $x \in(\pi, 2 \pi)$ के लिए $\sin x<0$, हम पाते हैं कि $f^{\prime}(x)>0$ और इसलिए $(\pi, 2 \pi)$ में $f$ वर्धमान है।
(c) उपरोक्त (a) और (b) से स्पष्ट है कि $(0,2 \pi)$ में $f$ न तो वर्धमान है और न ही ह्रासमान है।
उदाहरण 10 अंतराल ज्ञात कीजिए जिनमें $f(x)=x^{2}-4 x+6$ से प्रदत्त फलन $f$ (a) वर्धमान है (b) ह्रासमान है
हल यहाँ
$$ f(x)=x^{2}-4 x+6 $$ $ या \qquad f^{\prime}(x)=2 x-4 $
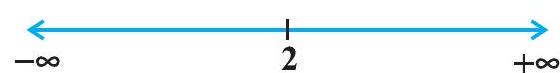
इसलिए, $f^{\prime}(x)=0$ से $x=2$ प्राप्त होता है। अब बिंदु $x=2$ वास्तविक रेखा को दो असंयुक्त अंतरालों, नामतः $(-\infty, 2)$ और $(2, \infty)$ (आकृति 6.3) में विभक्त
आकृति 6.3 करता है। अंतराल $(-\infty, 2)$ में $f^{\prime}(x)=2 x-4<0$ है। इसलिए, इस अंतराल में, $f$ ह्रासमान है। अंतराल $(2, \infty)$, में $f^{\prime}(x)>0$ है, इसलिए इस अंतराल में फलन $f$ वर्धमान है।
उदाहरण 11 वे अंतराल ज्ञात कीजिए जिनमें $f(x)=4 x^{3}-6 x^{2}-72 x+30$ द्वारा प्रदत्त फलन $f$, (a) वर्धमान (b) ह्रासमान है।
हल यहाँ
$$ \begin{aligned} f(x) & =4 x^{3}-6 x^{2}-72 x+30 \\ f^{\prime}(x) & =12 x^{2}-12 x-72 \\ & =12\left(x^{2}-x-6\right) \\ & =12(x-3)(x+2) \end{aligned} $$
इसलिए $f^{\prime}(x)=0$ से $x=-2,3$ प्राप्त होते हैं। $x=-2$ और $x=3$ वास्तविक रेखा को तीन असंयुक्त अंतरालों, नामतः $(-\infty,-2),(-2,3)$ और $(3, \infty)$ में विभक्त करता है (आकृति 6.4)।

आकृति 6.4
अंतरालों $(-\infty,-2)$ और $(3, \infty)$ में $f^{\prime}(x)$ धनात्मक है जबकि अंतराल $(-2,3)$ में $f^{\prime}(x)$ ॠणात्मक है। फलस्वरूप फलन $f$ अंतरालों $(-\infty,-2)$ और $(3, \infty)$ में वर्धमान है जबकि अंतराल $(-2,3)$ में फलन ह्रासमान है। तथापि $f, \mathbf{R}$ पर न तो वर्धमान है और न ही ह्रासमान है।
| अंतराल | $\boldsymbol{f}^{\prime}(\boldsymbol{x})$ का चिह्न | फलन $f$ की प्रकृति |
|---|---|---|
| $(-\infty,-2)$ | $(-)(-)>0$ | $f$ वर्धमान है |
| $(-2,3)$ | $(-)(+)<0$ | $f$ ह्रासमान है |
| $(3, \infty)$ | $(+)(+)>0$ | $f$ वर्धमान है |
उदाहरण 12 अंतराल ज्ञात कीजिए जिनमें प्रदत्त फलन $f(x)=\sin 3 x, x \in\left[0, \frac{\pi}{2}\right]$ में (a) वर्धमान है। (b) ह्रासमान है।
हल ज्ञात है कि
$f(x) =\sin 3 x $
या $\quad f(x) =3 \cos 3 x$
इसलिए, $f^{\prime}(x)=0$ से मिलता है $\cos 3 x=0$ जिससे $3 x=\frac{\pi}{2}, \frac{3 \pi}{2}$ (क्योंकि $x \in\left[0, \frac{\pi}{2}\right]$ $\Rightarrow 3 x \in\left[0, \frac{3 \pi}{2}\right]$ ) प्राप्त होता है। इसलिए, $x=\frac{\pi}{6}$ और $\frac{\pi}{2}$ है। अब बिंदु $x=\frac{\pi}{6}$, अंतराल $\left[0, \frac{\pi}{2}\right]$ को दो असंयुक्त अंतरालों $\left[0, \frac{\pi}{6}\right)$ और $\left(\frac{\pi}{6}, \frac{\pi}{2}\right]$ में विभाजित करता है।

आकृति 6.5
पुन: सभी $x \in\left[0, \frac{\pi}{6}\right)$ के लिए $f^{\prime}(x)>0$ क्योंकि $0 \leq x<\frac{\pi}{6} \Rightarrow 0 \leq 3 x<\frac{\pi}{2}$ और सभी $x \in\left(\frac{\pi}{6}, \frac{\pi}{2}\right)$ के लिए $f^{\prime}(x)<0$ क्योंकि $\frac{\pi}{6}<x \leq \frac{\pi}{2} \Rightarrow \frac{\pi}{2}<3 x \leq \frac{3 \pi}{2}$
इसलिए, अंतराल $\left[0, \frac{\pi}{6}\right)$ में $f$ वर्धमान है और अंतराल $\left(\frac{\pi}{6}, \frac{\pi}{2}\right)$ में ह्रासमान है।
इसके अतिरिक्त दिया गया फलन $x=0$ तथा $x=\frac{\pi}{6}$ पर संतत भी है। इसलिए प्रमेय 1 के द्वारा, $f,\left[0, \frac{\pi}{6}\right]$ में वर्धमान और $\left[\frac{\pi}{6}, \frac{\pi}{2}\right]$ में ह्रासमान है।
उदाहरण 13 अंतराल ज्ञात कीजिए जिनमें
$f(x)=\sin x+\cos x, 0 \leq x \leq 2 \pi$ द्वारा प्रदत्त फलन $f$,
वर्धमान या ह्रससमान है।
हल ज्ञात है कि
$$ \begin{array}{lrlr} & f(x) & =\sin x+\cos x, \quad 0 \leq x \leq 2 \pi \\ \text{या }&f^{\prime}(x) & =\cos x-\sin x & \end{array} $$
अब $f^{\prime}(x)=0$ से $\sin x=\cos x$ जिससे हमें $x=\frac{\pi}{4}, \frac{5 \pi}{4}$ प्राप्त होते हैं। क्योंकि $0 \leq x \leq 2 \pi$,
बिंदु $x=\frac{\pi}{4}$ और $x=\frac{5 \pi}{4}$ अंतराल $[0,2 \pi]$ को तीन असंयुक्त अंतरालों,
नामतः $\left[0, \frac{\pi}{4}\right)$, $\left(\frac{\pi}{4}, \frac{5 \pi}{4}\right)$ और $\left(\frac{5 \pi}{4}, 2 \pi\right]$ में विभक्त करते हैं।

आकृति 6.6
ध्यान दीजिए कि $f^{\prime}(x)>0$ यदि $x \in\left(0, \frac{\pi}{4}\right) \cup\left(\frac{5 \pi}{4}, 2 \pi\right]$
अतः अंतरालों $\left(0, \frac{\pi}{4}\right)$ और $\left(\frac{5 \pi}{4}, 2 \pi\right]$ में फलन $f$ वर्धमान है।
और $ f^{\prime}(x)<0 \text {, यदि } x \in\left(\frac{\pi}{4}, \frac{5 \pi}{4}\right) $
अतः $f$ अंतराल $\left(\frac{\pi}{4}, \frac{5 \pi}{4}\right)$ में ह्रासमान है।
| अंतराल | $f^{\prime}(x)$ का चिह्न | फलन की प्रकृति |
|---|---|---|
| $\left[0, \frac{\pi}{4}\right)$ | $>0$ | $f$ वर्धमान है |
| $\left(\frac{\pi}{4}, \frac{5 \pi}{4}\right)$ | $<0$ | $f$ ह्रासमान है |
| $\left(\frac{5 \pi}{4}, 2 \pi\right]$ | $>0$ | $f$ वर्धमान है |
प्रश्नावली 6.2
1. सिद्ध कीजिए $\mathbf{R}$ पर $f(x)=3 x+17$ से प्रदत्त फलन वर्धमान है।
Show Answer
#missing2. सिद्ध कीजिए कि $\mathbf{R}$ पर $f(x)=e^{2 x}$ से प्रदत्त फलन वर्धमान है।
Show Answer
#missing3. सिद्ध कीजिए $f(x)=\sin x$ से प्रदत्त फलन
(a) $\left(0, \frac{\pi}{2}\right)$ में वर्धमान है
(b) $\left(\frac{\pi}{2}, \pi\right)$ में ह्रासमान है
(c) $(0, \pi)$ में न तो वर्धमान है और न ही ह्रासमान है।
Show Answer
#missing4. अंतराल ज्ञात कीजिए जिनमें $f(x)=2 x^{2}-3 x$ से प्रदत्त फलन $f$
(a) वर्धमान
(b) ह्रासमान
Show Answer
#missing5. अंतराल ज्ञात कीजिए जिनमें $f(x)=2 x^{3}-3 x^{2}-36 x+7$ से प्रदत्त फलन $f$
(a) वर्धमान
(b) ह्रासमान
Show Answer
#missing6. अंतराल ज्ञात कीजिए जिनमें निम्नलिखित फलन $f$ वर्धमान या ह्रासमान है:
(a) $f(x) x^{2}+2 x+5$
(b) $f(x) 10-6 x-2 x^{2}$
(c) $f(x)-2 x^{3}-9 x^{2}-12 x+1$
(d) $f(x) 6-9 x-x^{2}$
(e) $f(x)(x+1)^{3}(x-3)^{3}$
Show Answer
#missing7. सिद्ध कीजिए कि $y=\log (1+x)-\frac{2 x}{2+x}, x>-1$, अपने संपूर्ण प्रांत में एक वर्धमान फलन है।
Show Answer
#missing8. $x$ के उन मानों को ज्ञात कीजिए जिनके लिए $y=[x(x-2)]^{2}$ एक वर्धमान फलन है।
Show Answer
#missing9. सिद्ध कीजिए कि $\left[0, \frac{\pi}{2}\right]$ में $y=\frac{4 \sin \theta}{(2+\cos \theta)}-\theta, \theta$ का एक वर्धमान फलन है।
Show Answer
#missing10. सिद्ध कीजिए कि लघुगणकीय फलन $(0, \infty)$ में वर्धमान फलन है।
Show Answer
#missing11. सिद्ध कीजिए कि $(-1,1)$ में $f(x)=x^{2}-x+1$ से प्रदत्त फलन न तो वर्धमान है और न ही ह्रासमान है।
Show Answer
#missing12. निम्नलिखित में कौन से फलन $\left(0, \frac{\pi}{2}\right)$ में ह्रासमान है ?
(A) $\cos x$
(B) $\cos 2 x$
(C) $\cos 3 x$
(D) $\tan x$
Show Answer
#missing13. निम्नलिखित अंतरालों में से किस अंतराल में $f(x)=x^{100}+\sin x-1$ द्वारा प्रदत्त फलन $f$ ह्रासमान है?
(A) $(0,1)$
(B) $\left(\frac{\pi}{2}, \pi\right)$
(C) $\left(0, \frac{\pi}{2}\right)$
(D) इनमें से कोई नही
Show Answer
#missing14. $a$ का वह न्यूनतम मान ज्ञात कीजिए जिसके लिए अंतराल $[1,2]$ में $f(x)=x^{2}+a x+1$ से प्रदत्त फलन वर्धमान है।
Show Answer
#missing15. मान लीजिए $[-1,1]$ से असंयुक्त एक अंतराल $\mathrm{I}$ हो तो सिद्ध कीजिए कि $\mathrm{I}$ में $f(x)=x+\frac{1}{x}$ से प्रदत्त फलन $f$, वर्धमान है।
Show Answer
#missing16. सिद्ध कीजिए कि फलन $f(x)=\log \sin x,\left(0, \frac{\pi}{2}\right)$ में वर्धमान और $\left(\frac{\pi}{2}, \pi\right)$ में ह्रासमान है।
Show Answer
#missing17. सिद्ध कीजिए कि फलन $f(x)=\log |\cos x|\left(0, \frac{\pi}{2}\right)$ में वर्धमान और $\left(\frac{3 \pi}{2}, 2 \pi\right)$ में ह्रासमान है।
Show Answer
#missing18. सिद्ध कीजिए कि $\mathbf{R}$ में दिया गया फलन $f(x)=x^{3}-3 x^{2}+3 x-100$ वर्धमान है।
Show Answer
#missing19. निम्नलिखित में से किस अंतराल में $y=x^{2} e^{-x}$ वर्धमान है?
(A) $(-\infty, \infty)$
(B) $(-2,0)$
(C) $(2, \infty)$
(D) $(0,2)$
Show Answer
#missing6.4 उच्चतम और निम्नतम (Maxima and Minima)
इस अनुच्छेद में, हम विभिन्न फलनों के उच्चतम और निम्नतम मानों की गणना करने में अवकलज की संकल्पना का प्रयोग करेंगे। वास्तव में हम एक फलन के आलेख के वर्तन बिंदुओं (Turning points) को ज्ञात करेंगे और इस प्रकार उन बिंदुओं को ज्ञात करेंगे जिन पर आलेख स्थानीय अधिकतम (या न्यूनतम) पर पहुँचता है। इस प्रकार के बिंदुओं का ज्ञान एक फलन का आलेख खींचने में बहुत उपयोगी होता है। इसके अतिरिक्त हम एक फलन का निरपेक्ष उच्चतम मान (Absolute maximum value) ओर निरपेक्ष न्यूनतम मान (Absolute minimum value) भी ज्ञात करेंगे जो कई अनुप्रयुक्त समस्याओं के हल के लिए आवश्यक हैं।
आइए हम दैनिक जीवन की निम्नलिखित समस्याओं पर विचार करें
(i) संतरों के वृक्षों के एक बाग से होने वाला लाभ फलन $\mathrm{P}(x)=a x+b x^{2}$ द्वारा प्रदत्त है जहाँ $a, b$ अचर हैं और $x$ प्रति एकड़ में संतरे के वृक्षों की संख्या है। प्रति एकड़ कितने वृक्ष अधिकतम लाभ देगें?
(ii) एक $60 \mathrm{~m}$ ऊँचे भवन से हवा में फेंकी गई एक गेंद $h(x)=60+x-\frac{x^{2}}{60}$ के द्वारा निर्धारित पथ के अनुदिश चलती है, जहाँ $x$ भवन से गेंद की क्षैतिज दूरी और $h(x)$ उसकी ऊँचाई है। गेंद कितनी अधिकतम ऊँचाई तक पहुँचेगी?
(iii) शत्रु का एक अपाचे हेलिकॉप्टर वक्र $f(x)=x^{2}+7$ द्वारा प्रदत्त पथ के अनुदिश उड़ रहा है। बिंदु $(1,2)$ पर स्थित एक सैनिक उस हेलिकॉप्टर को गोली मारना चाहता है जब हेलिकॉप्टर उसके निकटतम हो। यह निकटतम दूरी कितनी है?
उपर्युक्त समस्याओं में कुछ सर्वसामान्य है अर्थात् हम प्रदत्त फलनों के उच्चतम अथवा निम्नतम मान ज्ञात करना चाहते हैं। इन समस्याओं को सुलझाने के लिए हम विधिवत एक फलन का अधिकतम मान या न्यूनतम मान व स्थानीय उच्चतम व स्थानीय निम्नतम के बिंदुओं और इन बिंदुओं को निर्धारित करने के परीक्षण को परिभाषित करेंगे।
परिभाषा 3 मान लीजिए एक अंतराल I में एक फलन $f$ परिभाषित है, तब
(a) $f$ का उच्चतम मान $\mathrm{I}$ में होता है, यदि $\mathrm{I}$ में एक बिंदु $c$ का अस्तित्व इस प्रकार है कि $f(c) \geq f(x), \forall x \in \mathrm{I}$
संख्या $f(c)$ को $\mathrm{I}$ में $f$ का उच्चतम मान कहते हैं और बिंदु $c$ को $\mathrm{I}$ में $f$ के उच्चतम मान वाला बिंदु कहा जाता है।
(b) $f$ का निम्नतम मान $\mathrm{I}$ में होता है यदि $\mathrm{I}$ में एक बिंदु $c$ का अस्तित्व है इस प्रकार कि $f(c) \leq f(x), \forall x \in \mathrm{I}$
संख्या $f(c)$ को $\mathrm{I}$ में $f$ का निम्नतम मान कहते हैं और बिंदु $c$ को $\mathrm{I}$ में $f$ के निम्नतम मान वाला बिंदु कहा जाता है।
(c) I में $f$ एक चरम मान (extreme value) रखने वाला फलन कहलाता है यदि $\mathrm{I}$ में एक ऐसे बिंदु $c$ का अस्तित्व इस प्रकार है कि $f(c), f$ का उच्चतम मान अथवा निम्नतम मान है।
इस स्थिति में $f(c), \mathrm{I}$ में $f$ का चरम मान कहलाता है और बिंदु $c$ एक चरम बिंदु कहलाता है। आकृति 6.7
टिप्पणी आकृति 6.7 (a), (b) और (c) में हमने कुछ विशिष्ट फलनों के आलेख प्रदर्शित किए हैं जिनसे हमें एक बिंदु पर उच्चतम मान और निम्नतम मान ज्ञात करने में सहायता मिलती है। वास्तव में आलेखों से हम उन फलनों के जो अवकलित नहीं होते हैं। उच्चतम / निम्नतम मान भी ज्ञात कर सकते हैं, (उदाहरण 27)।

आकृति 6.7
उदाहरण $14 f(x)=x^{2}, x \in \mathbf{R}$ से प्रदत्त फलन $f$ के उच्चतम और निम्नतम मान, यदि कोई हों तो, ज्ञात कीजिए।
हल दिए गए फलन के आलेख (आकृति 6.8) से हम कह सकते हैं कि $f(x)=0$ यदि $x=0$ है और $f(x) \geq 0$, सभी $x \in \mathbf{R}$ के लिए।
इसलिए, $f$ का निम्नतम मान 0 है और $f$ के निम्नतम मान का बिंदु $x=0$ है। इसके अतिरिक्त आलेख से यह भी देखा जा सकता है कि फलन $f$ का कोई उच्चतम मान नहीं है, अत: $\mathbf{R}$ में $f$ के उच्चतम मान का बिंदु नहीं है।

आकृति 6.8
टिप्पणी यदि हम फलन के प्रांत को केवल $[-2,1]$ तक सीमित करें तब $x=-2$ पर $f$ का उच्चतम मान $(-2)^{2}=4$ है।
उदाहरण 15 $f(x)=|x|, x \in \mathbf{R}$ द्वारा प्रदत्त फलन $f$ के उच्चतम और निम्नतम मान, यदि कोई हो तो, ज्ञात कीजिए।
हल दिए गए फलन के आलेख (आकृति 6.9) से
$$ f(x) \geq 0 \text {, सभी } x \in \mathbf{R} \text { और } f(x)=0 \text { यदि } x=0 \text { है। } $$
इसलिए, $f$ का निम्नतम मान 0 है और $f$ के निम्नतम मान का बिंदु $x=0$ है। और आलेख से यह भी स्पष्ट है $\mathbf{R}$ में $f$ का कोई उच्चतम मान नहीं है। अतः $\mathbf{R}$ में कोई उच्चतम मान का बिंदु नहीं है।

आकृति 6.9
टिप्पणी
(i) यदि हम फलन के प्रांत को केवल $[-2,1]$ तक सीमित करें, तो $f$ का उच्चतम मान $|-2|=2$ होगा।
(ii) उदाहरण 27 में ध्यान दें कि फलन $f, x=0$ पर अवकलनीय नहीं है।
उदाहरण 16 $f(x)=x, x \in(0,1)$ द्वारा प्रदत्त फलन के उच्चतम और निम्नतम मान, यदि कोई हो तो, ज्ञात कीजिए।
हल दिए अंतराल $(0,1)$ में दिया फलन एक निरंतर वर्धमान फलन है। फलन $f$ के आलेख (आकृति 6.10) से ऐसा प्रतीत होता है कि फलन का निम्नतम मान 0 के दायीं ओर के निकटतम बिंदु और उच्चतम मान 1 के बायीं ओर के निकटतम बिंदु पर होना चाहिए। क्या ऐसे बिंदु उपलब्ध हैं? ऐसे बिंदुओं को अंकित करना संभव नहीं है। वास्तव में, यदि 0 का निकटतम बिंदु $x _{0}$ हो तो $\frac{x _{0}}{2}<x _{0}$ सभी $x _{0} \in(0,1)$ के लिए और यदि 1 का निकटतम बिंदु $x _{1}$ हो तो सभी $x _{1} \in(0,1)$ के लिए $\frac{x _{1}+1}{2}>x _{1}$ है। इसलिए दिए गए फलन का अंतराल $(0,1)$ में न तो कोई उच्चतम मान है और न ही कोई निम्नतम मान है।

आकृति 6.10
टिप्पणी पाठक देख सकते हैं कि उदाहरण 28 में यदि $f$ के प्रांत में 0 और 1 को सम्मिलित कर लिया जाए अर्थात $f$ के प्रांत को बढ़ाकर $[0,1]$ कर दिया जाए तो फलन का निम्नतम मान $x=0$ पर 0 और उच्चतम मान $x=1$ पर 1 है। वास्तव में हम निम्नलिखित परिणाम पाते हैं (इन परिणामों की उपपत्ति इस पुस्तक के क्षेत्र से बाहर है)।
प्रत्येक एकदिष्ट (monotonic) फलन अपने परिभाषित प्रांत के अंत्य बिंदुओं पर उच्चतम/निम्नतम ग्रहण करता है।
इस परिणाम का अधिक व्यापक रूप यह है कि संवृत्त अंतराल पर प्रत्येक संतत फलन के उच्चतम और निम्नष्ठ मान होते हैं।
टिप्पणी किसी अंतराल I में एकदिष्ट फलन से हमारा अभिप्राय है कि I में फलन या तो वर्धमान है या ह्रासमान है।
इस अनुच्छेद में एक संवृत्त अंतराल पर परिभाषित फलन के उच्चतम और निम्नतम मानों के बारे में बाद में विचार करेंगे।
आइए अब आकृति 6.11 में दर्शाए गए किसी फलन के आलेख का अध्ययन करें। देखिए कि फलन का आलेख बिंदुओं $\mathrm{A}, \mathrm{B}, \mathrm{C}$ तथा $\mathrm{D}$ पर वर्धमान से ह्रासमान या विलोमतः ह्रासमान से वर्धमान होता है। इन बिंदुओं को फलन के वर्तन बिंदु कहते हैं। पुनः ध्यान दीजिए कि वर्तन बिंदुओं पर आलेख में एक छोटी पहाड़ी या छोटी घाटी बनती है। मोटे तौर पर बिंदुओं $\mathrm{A}$ तथा $\mathrm{C}$ में से प्रत्येक के सामीप्य (Neighbourhood) में फलन का निम्नतम मान है, जो उनकी अपनी-अपनी घाटियों के अधोभागों (Bottom) पर है। इसी प्रकार बिंदुओं $\mathrm{B}$ तथा $\mathrm{D}$ में से प्रत्येक के सामीप्य में फलन का उच्चतम मान है, जो उनकी अपनी-अपनी पहाड़ियों के शीर्षों पर है। इस कारण से बिंदुओं $\mathrm{A}$ तथा $\mathrm{C}$ को स्थानीय निम्नतम मान (या सापेक्ष निम्नतम मान) का बिंदु तथा $\mathrm{B}$ और $\mathrm{D}$ को स्थानीय उच्चतम मान (या सापेक्ष उच्चतम मान) के बिंदु समझा जा सकता है। फलन के स्थानीय उच्चतम मान और स्थानीय निम्नतम मानों को क्रमशः फलन का स्थानीय उच्चतम और स्थानीय निम्नतम कहा जाता है।
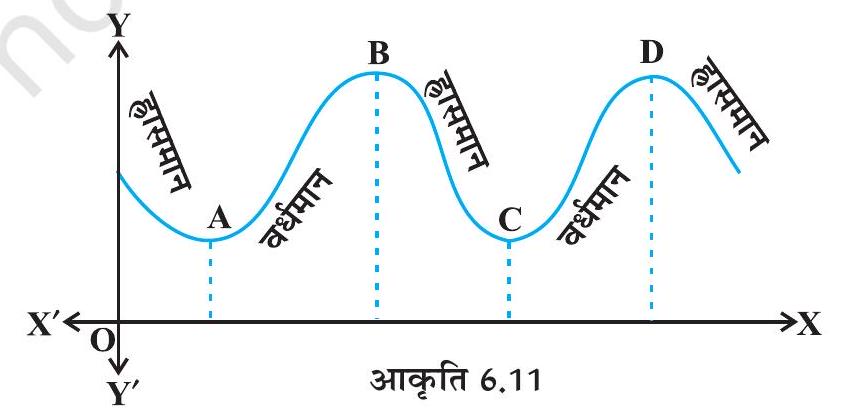
आकृति 6.11
अब हम औपचारिक रूप से निम्नलिखित परिभाषा देते हैं।
परिभाषा 4 मान लीजिए $f$ एक वास्तविक मानीय फलन है और $c$ फलन $f$ के प्रांत में एक आंतरिक बिंदु है। तब
(a) $c$ को स्थानीय उच्चतम का बिंदु कहा जाता है यदि एक ऐसा $h>0$ है
कि $(c-h, c+h)$ में सभी $x$ के लिए $f(c) \geq f(x)$ हो।
तब $f(c)$, फलन $f$ का स्थानीय उच्चतम मान कहलाता है।
(b) $c$ को स्थानीय निम्नतम का बिंदु कहा जाता है यदि एक ऐसा $h>0$ है कि $(c-h, c+h)$ में सभी
$x$ के लिए $f(c)<f(x)$ हो। तब $f(c)$,
फलन $f$ का स्थानीय निम्नतम मान कहलाता है।
ज्यामितीय दृष्टिकोण से, उपर्युक्त परिभाषा का अर्थ है कि यदि $x=c$, फलन $f$ का स्थानीय उच्चतम का बिंदु है, तो $c$ के आसपास का आलेख आकृति 6.12(a) के अनुसार होगा। ध्यान दीजिए कि अंतराल $(c-h, c)$ में फलन $f$ वर्धमान (अर्थात् $f^{\prime}(x)>0$ ) और अंतराल $(c, c+h)$ में फलन ह्रासमान (अर्थात् $f^{\prime}(x)<0$ ) है।
इससे यह निष्कर्ष निकलता है कि $f^{\prime}(c)$ अवश्य ही शून्य होना चाहिए।
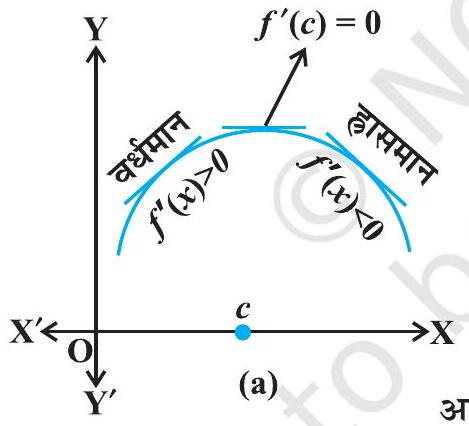
आकृति 6.12
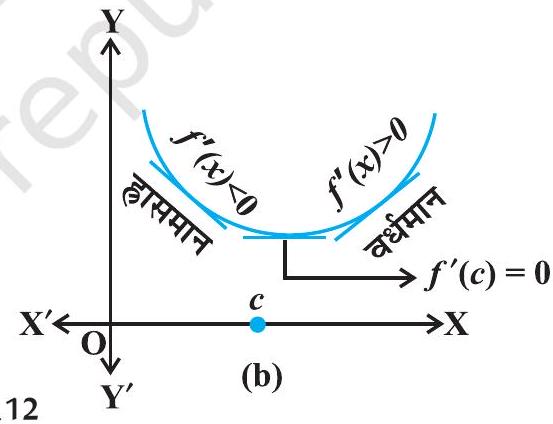
इसी प्रकार, यदि $c$, फलन $f$ का स्थानीय निम्नतम बिंदु है तो $c$ के आसपास का आलेख आकृति 6.14(b) के अनुसार होगा। यहाँ अंतराल $(c-h, c)$ में $f$ ह्रासमान (अर्थात् $\left.f^{\prime}(x)<0\right)$ है और अंतराल $(c, c+h)$ में $f$ वर्धमान (अर्थात, $f^{\prime}(x)>0$ ) है। यह पुनः सुझाव देता है कि $f^{\prime}(c)$ अवश्य ही शून्य होना चाहिए।
उपर्युक्त परिचर्चा से हमें निम्नलिखित परिभाषा प्राप्त होती है (बिना उपपत्ति)।
प्रमेय 2 मान लीजिए एक विवृत्त अंतराल $\mathrm{I}$ में $f$ एक परिभाषित फलन है। मान लीजिए $c \in \mathrm{I}$ कोई बिंदु है। यदि $f$ का $x=c$ पर एक स्थानीय उच्चतम या एक स्थानीय निम्नतम का बिंदु है तो $f^{\prime}(c)$ $=0$ है या $f$ बिंदु $c$ पर अवकलनीय नहीं है।
टिप्पणी उपरोक्त प्रमेय का विलोम आवश्यक नहीं है कि सत्य हो जैसे कि एक बिंदु जिस पर अवकलज शून्य हो जाता है तो यह आवश्यक नहीं है कि वह स्थानीय उच्चतम या स्थानीय निम्नतम का बिंदु है। उदाहरणतया यदि $f(x)=x^{3}$ हो तो $f^{\prime}(x)=3 x^{2}$ और इसलिए $f^{\prime}(0)=0$ है। परन्तु 0 न तो स्थानीय उच्चतम और न ही स्थानीय निम्नतम बिंदु है। आकृति 6.15
टिप्पणी फलन $f$ के प्रांत में एक बिंदु $c$, जिस पर या तो $f^{\prime}(c)=0$ है या $f$ अवकलनीय नहीं है, $f$ का क्रांतिक बिंदु (Critical Point) कहलाता है। ध्यान दीजिए कि यदि $f$ बिंदु $c$ पर संतत है और $f^{\prime}(c)=0$ है तो यहाँ एक ऐसे $h>0$ का अस्तित्व है कि अंतराल $(c-h, c+h)$ में $f$ अवकलनीय है।
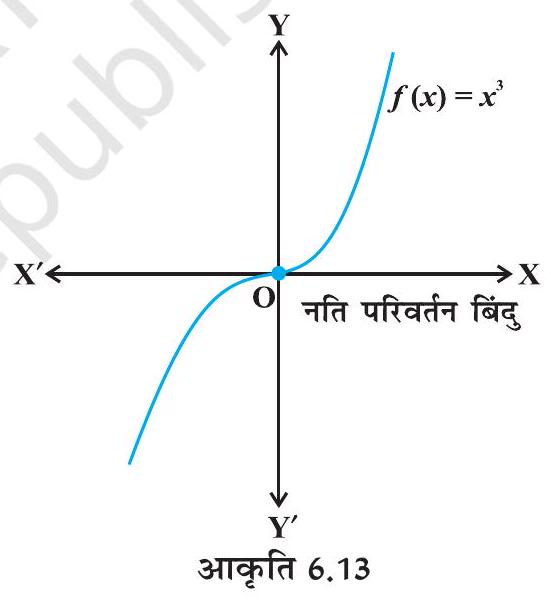
आकृति 6.13
अब हम केवल प्रथम अवकलजों का प्रयोग करके स्थानीय उच्चतम बिंदु या स्थानीय निम्नतम बिंदुओं को ज्ञात करने की क्रियाविधि प्रस्तुत करेंगे।
प्रमेय 3 (प्रथम अवकलज परीक्षण) मान लीजिए कि एक फलन $f$ किसी विवृत्त अंतराल I पर परिभाषित है। मान लीजिए कि $f$ अंतराल $\mathrm{I}$ में स्थित क्रांतिक बिंदु $c$ पर संतत है। तब
(i) $x$ के बिंदु $c$ से हो कर बढ़ने के साथ-साथ, यदि $f^{\prime}(x)$ का चिह्न धन से ऋण में परिवर्तित होता है अर्थात् यदि बिंदु $c$ के बायों ओर और उसके पर्याप्त निकट के प्रत्येक बिंदु पर $f^{\prime}(x)>0$ तथा $c$ के दायीं ओर और पर्याप्त निकट के प्रत्येक बिंदु पर $f^{\prime}(x)<0$ हो तो $c$ स्थानीय उच्चतम एक बिंदु है।
(ii) $x$ के बिंदु $c$ से हो कर बढ़ने के साथ-साथ यदि $f^{\prime}(x)$ का चिह्न ऋण से धन में परिवर्तित होता है, अर्थात् यदि बिंदु $c$ के बायों ओर और उसके पर्याप्त निकट के प्रत्येक बिंदु पर $f^{\prime}(x)<0$ तथा $c$ के दायीं ओर और उसके पर्याप्त निकट के प्रत्येक बिंदु पर $f^{\prime}(x)>0$ हो तो $c$ स्थानीय निम्नतम बिंदु है।
(iii) $x$ के बिंदु $c$ से हो कर बढ़ने के साथ यदि $f^{\prime}(x)$ का चिह्न परिवर्तित नहीं होता है, तो $c$ न तो स्थानीय उच्चतम बिंदु है और न स्थानीय निम्नतम बिंदु। वास्तव में, इस प्रकार के बिंदु को नति परिवर्तन बिंदु (Point of Inflection) (आकृति 6.13) कहते हैं।
टिप्पणी यदि $c$ फलन $f$ का एक स्थानीय उच्चतम बिंदु है तो $f(c)$ फलन $f$ का स्थानीय उच्चतम मान है। इसी प्रकार, यदि $c$ फलन $f$ का एक स्थानीय निम्नतम बिंदु है, तो $f(c)$ फलन $f$ का स्थानीय निम्नतम मान है।
आकृतियाँ 6.13 और 6.14 प्रमेय 3 की ज्यामितीय व्याख्या करती है।
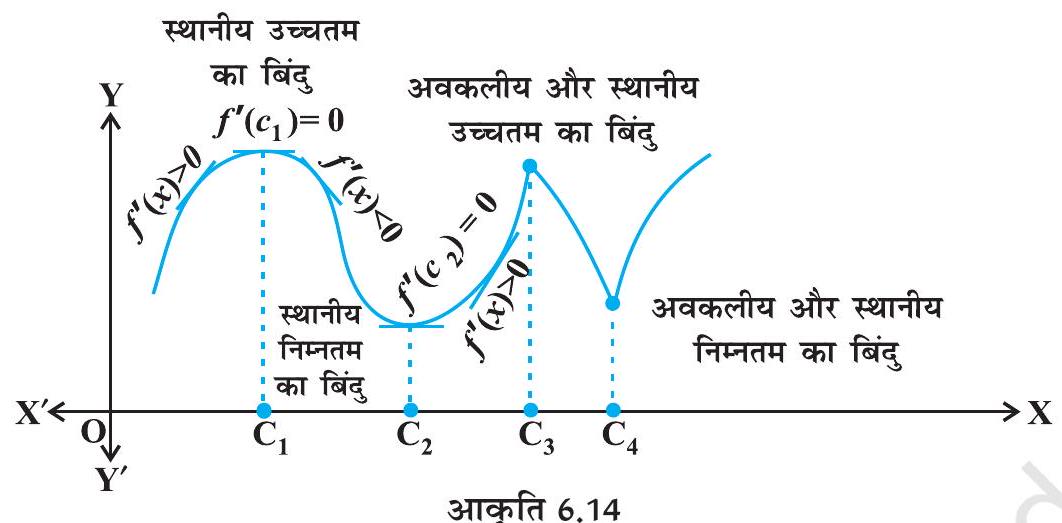
आकृति 6.14
उदाहरण 17 $f(x)=x^{3}-3 x+3$ द्वारा प्रदत्त फलन के लिए स्थानीय उच्चतम और स्थानीय निम्नतम के सभी बिंदुओं को ज्ञात कीजिए।
हल यहाँ
$$ \begin{array}{lrl} & f(x) & =x^3-3 x+3 \\ \text{ या } & f^{\prime}(x) & =3 x^2-3=3(x-1)(x+1) \\ \text{ या } & f^{\prime}(x) &=0 \text{ at } x=1 \text{ and } x=-1 \end{array} $$
इस प्रकार, केवल $x= \pm 1$ ही ऐसे क्रांतिक बिंदु हैं जो $f$ के स्थानीय उच्चतम और/या स्थानीय निम्नतम संभावित बिंदु हो सकते हैं। पहले हम $x=1$ पर परीक्षण करते हैं।
ध्यान दीजिए कि 1 के निकट और 1 के दायीं ओर $f^{\prime}(x)>0$ है और 1 के निकट और 1 के बायीं ओर $f^{\prime}(x)<0$ है। इसलिए प्रथम अवकलज परीक्षण द्वारा $x=1$, स्थानीय निम्नतम बिंदु है और स्थानीय निम्नतम मान $f(1)=1$ है। $x=-1$ की दशा में, -1 के निकट और -1 के बायीं ओर $f^{\prime}(x)>0$ और -1 के निकट और -1 के दायीं ओर $f^{\prime}(x)<0$ है। इसलिए प्रथम अवकलज परीक्षण द्वारा $x=-1$ स्थानीय उच्चतम का बिंदु है और स्थानीय उच्चतम मान $f(-1)=5$ है।
$ \begin{array}{|c|c|c|} \hline & x \text{ के मान } & f^{\prime}(x)=3(x-1)(x+1) \text{ का चिह्न } \\ \hline {\begin{array}{l} \text{ 1 के निकट } \end{array}} & \text{ दायीं ओर (माना 1.1) } & > 0 \\ \hline & \text{ बायीं ओर (माना 0.9) } & <0 \\ \hline \text{ -1 के निकट } & \text{ दायीं ओर (माना - 0.9) } & <0 \\ \hline & \text{ बायीं ओर (माना - 1.1) } & >0 \\ \hline \end{array} $
उदाहरण 18 $f(x)=2 x^{3}-6 x^{2}+6 x+5$ द्वारा प्रदत्त फलन $f$ के स्थानीय उच्चतम और स्थानीय निम्नतम बिंदु ज्ञात कीजिए।
हल यहाँ
$$ \begin{array}{lrl} & f(x) & =2 x^{3}-6 x^{2}+6 x+5 \\ \text{ or }& f^{\prime}(x) & =6 x^{2}-12 x+6=6(x-1)^{2} \\ \text{ or }& f^{\prime}(x) & =0 \quad \Rightarrow \quad x=1 \end{array} $$
इस प्रकार केवल $x=1$ ही $f$ का क्रांतिक बिंदु है। अब हम इस बिंदु पर $f$ के स्थानीय उच्चतम या स्थानीय निम्नतम के लिए परीक्षण करेंगे। देखिए कि सभी $x \in \mathbf{R}$ के लिए $f^{\prime}(x) \geq 0$ और विशेष रूप से 1 के समीप और 1 के बायों ओर और दायीं ओर के मानों के लिए $f^{\prime}(x)>0$ है। इसलिए प्रथम अवकलज परीक्षण से बिंदु $x=1$ न तो स्थानीय उच्चतम का बिंदु है और न ही स्थानीय निम्नतम का बिंदु है। अत: $x=1$ एक नति परिवर्तन (inflection) बिंदु है।
टिप्पणी ध्यान दीजिए कि उदाहरण 30 में $f^{\prime}(x)$ का चिह्न अंतराल $\mathbf{R}$ में कभी भी नहीं बदलता। अतः $f$ के आलेख में कोई भी वर्तन बिंदु नहीं है और इसलिए स्थानीय उच्चतम या स्थानीय निम्नतम का कोई भी बिंदु नहीं है।
अब हम किसी प्रदत्त फलन के स्थानीय उच्चतम और स्थानीय निम्नतम के परीक्षण के लिए एक दूसरी क्रियाविधि प्रस्तुत करेंगे। यह परीक्षण प्रथम अवकलज परीक्षण की तुलना में प्रायः सरल है।
प्रमेय 4 मान लीजिए कि $f$, किसी अंतराल $\mathrm{I}$ में परिभाषित एक फलन है तथा $c \in \mathrm{I}$ है। मान लीजिए कि $f, c$ पर दो बार लगातार अवकलनीय है। तब
(i) यदि $f^{\prime}(c)=0$ और $f^{\prime \prime}(c)<0$ तो $x=c$ स्थानीय उच्चतम का एक बिंदु है।
इस दशा में $f$ का स्थानीय उच्चतम मान $f(c)$ है।
(ii) यदि $f^{\prime}(c)=0$ और $f^{\prime \prime}(c)>0$ तो $x=c$ स्थानीय निम्नतम का एक बिंदु है।
इस दशा में $f$ का स्थानीय निम्नतम मान $f(c)$ है।
(iii) यदि $f^{\prime}(c)=0$ और $f^{\prime \prime}(c)=0$ है तो यह परीक्षण असफल हो जाता है।
इस स्थिति में हम पुनः प्रथम अवकलज परीक्षण पर वापस जाकर यह ज्ञात करते हैं कि $c$ उच्चतम, निम्नतम या नति परिवर्तन का बिंदु है।
टिप्पणी बिंदु $c$ पर $f$ दो बार लगातार अवकलनीय है इससे हमारा तात्पर्य कि $c$ पर $f$ के द्वितीय अवकलज का अस्तित्व है।
उदाहरण 19 $f(x)=3+|x|, x \in \mathbf{R}$ द्वारा प्रदत्त फलन $f$ का स्थानीय निम्नतम मान ज्ञात कीजिए।
हल ध्यान दीजिए कि दिया गया $x=0$ पर अवकलनीय नहीं है। इस प्रकार द्वितीय अवकलज परीक्षण असफल हो जाता है। अब हम प्रथम अवकलज परीक्षण करते हैं। नोट कीजिए कि 0 फलन $f$ का एक क्रांतिक बिंदु है। अब 0 के बायीं ओर, $f(x)=3-x$ और इसलिए $f^{\prime}(x)=-1<0$

है साथ ही 0 के दायीं ओर, $f(x)=3+x$ है और इसलिए $f^{\prime}(x)=1>0$ है। अतएव, प्रथम अवकलज परीक्षण द्वारा $x=0, f$ का स्थानीय निम्नतम बिंदु है तथा $f$ का स्थानीय न्यूनतम मान $f(0)=3$ है।
उदाहरण $20 f(x)=3 x^{4}+4 x^{3}-12 x^{2}+12$ द्वारा प्रदत्त फलन $f$ के स्थानीय उच्चतम और
स्थानीय निम्नतम मान ज्ञात कीजिए।
हल यहाँ
या $ f(x)=3 x^{4}+4 x^{3}-12 x^{2}+12 $
$f^{\prime}(x)=12 x^{3}+12 x^{2}-24 x=12 x(x-1)(x+2)$
या $x=0, x=1$ और $x=-2$ पर $f^{\prime}(x)=0$ है।
अब $ f^{\prime \prime}(x)=36 x^{2}+24 x-24=12\left(3 x^{2}+2 x-2\right) $
$$ \left\{\begin{array}{c} f^{\prime \prime}(0)=-24<0 \\ f^{\prime \prime}(1)=36>0 \\ f^{\prime \prime}(-2)=72>0 \end{array}\right. $$
इसलिए, द्वितीय अवकलज परीक्षण द्वारा $x=0$ स्थानीय उच्चतम बिंदु है और $f$ का स्थानीय उच्चतम मान $f(0)=12$ है। जबकि $x=1$ और $x=-2$ स्थानीय निम्नतम बिंदु है और स्थानीय निम्नतम मान $f(1)=7$ और $f(-2)=-20$ है।
उदाहरण 21 $ f(x)=2 x^{3}-6 x^{2}+6 x+5$ द्वारा प्रदत्त फलन $f$ के स्थानीय उच्चतम और स्थानीय निम्नतम के सभी बिंदु ज्ञात कीजिए।
हल यहाँ पर
$$ \text{या} \qquad \left\{\begin{array}{1}& f(x)=2 x^{3}-6 x^{2}+6 x+5 \\ \end{array}\right. $$
$$ \begin{aligned} & \left\{\begin{array}{l} f^{\prime}(x)=6 x^{2}-12 x+6=6(x-1)^{2} \\ f^{\prime \prime}(x)=12(x-1) \end{array}\right. \end{aligned} $$
अब $f^{\prime}(x)=0$ से $x=-1$ प्राप्त होता है। तथा $f^{\prime \prime}(1)=0$ है। इसलिए यहाँ द्वितीय अवकलज परीक्षण असफल है। अतः हम प्रथम अवकलज परीक्षण की ओर वापस जाएँगे।
हमने पहले ही (उदाहरण 30) में देखा है कि प्रथम अवकलज परीक्षण की दृष्टि से $x=1$ न तो स्थानीय उच्चतम का बिंदु है और न ही स्थानीय निम्नतम का बिंदु है अपितु यह नति परिवर्तन का बिंदु है।
उदाहरण 22 ऐसी दो धन संख्याएँ ज्ञात कीजिए जिनका योग 15 है और जिनके वर्गों का योग न्यूनतम हो।
हल मान लीजिए पहली संख्या $x$ है तब दूसरी संख्या $15-x$ है। मान लीजिए इन संख्याओं के वर्गों का योग $\mathrm{S}(x)$ से व्यक्त होता है। तब
$$ S(x)=x^{2}+(15-x)^{2}=2 x^{2}-30 x+225 $$
$$ \begin{gathered} \left\{\begin{array}{l} S^{\prime}(x)=4 x-30 \\ S^{\prime \prime}(x)=4 \end{array}\right. \end{gathered} $$
अब $\mathrm{S}^{\prime}(x)=0$ से $x=\frac{15}{2}$ प्राप्त होता है तथा $\mathrm{S}^{\prime \prime}\left(\frac{15}{2}\right)=4>0$ है। इसलिए द्वितीय अवकलज परीक्षण द्वारा $S$ के स्थानीय निम्नतम का बिंदु $x=\frac{15}{2}$ है। अतः जब संख्याएँ $\frac{15}{2}$ और $15-\frac{15}{2}=\frac{15}{2}$ हो तो संख्याओं के वर्गों का योग निम्नतम होगा।
टिप्पणी उदाहरण 22 की भाँति यह सिद्ध किया जा सकता है कि ऐसी दो घन संख्याएँ जिनका योग $k$ है और जिनके वर्गों का योग न्यूनतम हो तो ये संख्याएँ $\frac{k}{2}, \frac{k}{2}$ होंगी।
उदाहरण 23 बिंदु $(0, c)$ से परवलय $y=x^{2}$ की न्यूनतम दूरी ज्ञात कीजिए जहाँ $\frac{1}{2} \leq c \leq 5$ है।
हल मान लीजिए परवलय $y=x^{2}$ पर $(h, k)$ कोई बिंदु है। मान लीजिए $(h, k)$ और $(0, c)$ के बीच दूरी $\mathrm{D}$ है। तब
$$ \begin{equation*} \mathrm{D}=\sqrt{(h-0)^{2}+(k-c)^{2}}=\sqrt{h^{2}+(k-c)^{2}} \tag{1} \end{equation*} $$
क्योंकि $(h, k)$ परवलय $y=x^{2}$ पर स्थित है अत: $k=h^{2}$ है। इसलिए (1) से
$$ \mathrm{D} \equiv \mathrm{D}(k)=\sqrt{k+(k-c)^{2}} $$
$ या \qquad \mathrm{D}^{\prime}(k)=\frac{1+2(k-c)}{\sqrt{k+(k-c)^{2}}} $
$ अब \qquad \mathrm{D}^{\prime}(k)=0 \text { से } k=\frac{2 c-1}{2} \text { प्राप्त होता है } $
ध्यान दीजिए कि जब $k<\frac{2 c-1}{2}$, तब $2(k-c)+1<0$, अर्थात् $\mathrm{D}^{\prime}(k)<0$ है तथा जब $k>\frac{2 c-1}{2}तब $2(k-c)+1>0$ है अर्थात् $\mathrm{D}^{\prime}(k)>0$ (इस प्रकार प्रथम अवकलज परीक्षण से $k=\frac{2 c-1}{2}$ पर $k$ निम्नतम है।$
अतः अभीष्ट न्यूनतम दूरी
$$ \mathrm{D}\left(\frac{2 c-1}{2}\right)=\sqrt{\frac{2 c-1}{2}+\left(\frac{2 c-1}{2}-c\right)^{2}}=\frac{\sqrt{4 c-1}}{2} \text { है। } $$
टिप्पणी पाठक ध्यान दें कि उदाहरण 23 में हमने द्वितीय अवकलज परीक्षण के स्थान पर प्रथम अवकलज परीक्षण का प्रयोग किया है क्योंकि यह सरल एवं छोटा है।
उदाहरण 24 मान लीजिए बिंदु $\mathrm{A}$ और $\mathrm{B}$ पर क्रमशः $\mathrm{AP}$ तथा $\mathrm{BQ}$ दो उर्ध्वाधर स्तंभ है। यदि $\mathrm{AP}=16 \mathrm{~m}, \mathrm{BQ}=22 \mathrm{~m}$ और $\mathrm{AB}=20 \mathrm{~m}$ हों तो $\mathrm{AB}$ पर एक ऐसा बिंदु $\mathrm{R}$ ज्ञात कीजिए ताकि $\mathrm{RP}^{2}+\mathrm{RQ}^{2}$ निम्नतम हो।
हल मान लीजिए $\mathrm{AB}$ पर एक बिंदु $\mathrm{R}$ इस प्रकार है कि $\mathrm{AR}=x \mathrm{~m}$ है। तब $\mathrm{RB}=(20-x) \mathrm{m}$ (क्योंकि $\mathrm{AB}=20 \mathrm{~m})$ आकृति 6.16 से
$$ \begin{aligned} & \mathrm{RP}^{2}=\mathrm{AR}^{2}+\mathrm{AP}^{2} \\ \end{aligned} $$
$ \begin{aligned} \text { और } \quad \mathrm{RQ}^{2}=\mathrm{RB}^{2}+\mathrm{BQ}^{2} \end{aligned} $

आकृति 6.16
$इसलिए \qquad \mathrm{RP}^{2}+\mathrm{RQ}^{2}=\mathrm{AR}^{2}+\mathrm{AP}^{2}+\mathrm{RB}^{2}+\mathrm{BQ}^{2}$ $$ \begin{aligned} & =x^{2}+(16)^{2}+(20-x)^{2}+(22)^{2} \\ & =2 x^{2}-40 x+1140 \end{aligned} $$
$ मान लीजिए कि \qquad \mathrm{S} \equiv \mathrm{S}(x)=\mathrm{RP}^{2}+\mathrm{RQ}^{2}=2 x^{2}-40 x+1140$ है।
$ अतः \qquad \mathrm{S}^{\prime}(x)=4 x-40$ है।
अब $\mathrm{S}^{\prime}(x)=0$ से $x=10$ प्राप्त होता है और सभी $x$ के लिए $\mathrm{S}^{\prime \prime}(x)=4>0$ है और इसलिए $\mathrm{S}^{\prime \prime}(10)>0$ है। इसलिए द्वितीय अवकलज परीक्षण से $x=10, \mathrm{~S}$ का स्थानीय निम्नतम का बिंदु है। अतः $\mathrm{AB}$ पर $\mathrm{R}$ की $\mathrm{A}$ से दूरी $\mathrm{AR}=x=10 \mathrm{~m}$ है।
उदाहरण 25 यदि एक समलंब चतुर्भुज के आधार के अतिरिक्त तीनों भुजाओं की लंबायों $10 \mathrm{~cm}$ है तब समलंब चतुर्भुज का अधिकतम क्षेत्रफल ज्ञात कीजिए।
हल अभीष्ट समलंब को आकृति 6.17 में दर्शाया गया है। $\mathrm{AB}$ पर $\mathrm{DP}$ तथा $\mathrm{CQ}$ लंब खींचिए। मान लीजिए $\mathrm{AP}=x \mathrm{~cm}$ है। ध्यान दीजिए कि $\triangle \mathrm{APD} \cong \triangle \mathrm{BQC}$ है इसलिए $\mathrm{QB}=x \mathrm{~cm}$ है। और पाइथागोरस प्रमेय से, $\mathrm{DP}=\mathrm{QC}=\sqrt{100-x^{2}}$ है। मान लीजिए समलंब चतुर्भुज का क्षेत्रफल $\mathrm{A}$ है।

आकृति 6.17 अत:
$$ \begin{aligned} \mathrm{A} & \equiv \mathrm{A}(x) \\ & =\frac{1}{2} \text { (समांतर भुजाओं का योग) (ऊँचाई) } \\ & =\frac{1}{2}(2 x+10+10)\left(\sqrt{100-x^{2}}\right) \\ & =(x+10)\left(\sqrt{100-x^{2}}\right) \end{aligned} $$
या
$$ \begin{aligned} \mathrm{A}^{\prime}(x) & =(x+10) \frac{(-2 x)}{\sqrt{100-x^{2}}}+\left(\sqrt{100-x^{2}}\right) \\ & =\frac{-2 x^{2}-10 x+100}{\sqrt{100-x^{2}}} \end{aligned} $$
अब $\mathrm{A}^{\prime}(x)=0$ से $2 x^{2}+10 x-100=0$, जिससे $x=5$ और $x=-10$
प्राप्त होता है। क्योंकि $x$ दूरी को निरूपित करता है इसलिए यह ऋण नहीं हो सकता है। इसलिए $x=5$ है। अब
$$ \begin{aligned} \mathrm{A}^{\prime \prime}(x) & =\frac{\sqrt{100-x^{2}}(-4 x-10)-\left(-2 x^{2}-10 x+100\right) \frac{(-2 x)}{\sqrt[2]{100-x^{2}}}}{100-x^{2}} \\ & =\frac{2 x^{3}-300 x-1000}{\left(100-x^{2}\right)^{\frac{3}{2}}} \text { (सरल करने पर) } \end{aligned} $$ अतः $ A^{\prime \prime}(5)=\frac{2(5)^{3}-300(5)-1000}{\left(100-(5)^{2}\right)^{\frac{3}{2}}}=\frac{-2250}{75 \sqrt{75}}=\frac{-30}{\sqrt{75}}<0 $
इस प्रकार, $x=5$ पर समलंब का क्षेत्रफल अधिकतम है और अधिकतम क्षेत्रफल
$$ A(5)=(5+10) \sqrt{100-(5)^{2}}=15 \sqrt{75}=75 \sqrt{3} \mathrm{~cm}^{2} \text { है। } $$
उदाहरण 26 सिद्ध कीजिए कि एक शंकु के अंतर्गत महत्तम वक्रपृष्ठ वाले लंब वृत्तीय बेलन की त्रिज्या शंकु की त्रिज्या की आधी होती है।
हल मान लीजिए शंकु के आधार की त्रिज्या $\mathrm{OC}=r$ और ऊँचाई $\mathrm{OA}=h$ है। मान लीजिए कि दिए हुए शंकु के अंतर्गत बेलन के आधार के वृत्त की त्रिज्या $\mathrm{OE}=x$ है (आकृति 6.18)। बेलन की ऊँचाई $\mathrm{QE}$ के लिए:
$ \begin{array}{llll} \quad & \frac{QE}{OA} & =\frac{EC}{OC} \quad(\text { क्योंकि } \Delta QEC \sim \Delta AOC) \\ \text{ or } & \frac{QE}{h} & =\frac{r-x}{r} \\ \text{ or } & QE & =\frac{h(r-x)}{r} \end{array} $
मान लीजिए बेलन का वक्रपृष्ठ $\mathrm{S}$ है । तब
$$ \begin{aligned} & S \equiv S(x)=\frac{2 \pi x h(r-x)}{r}=\frac{2 \pi h}{r}\left(r x-x^{2}\right) \\ & \left\{\begin{array}{l} S^{\prime}(x)=\frac{2 \pi h}{r}(r-2 x) \\ S^{\prime \prime}(x)=\frac{-4 \pi h}{r} \end{array}\right. \end{aligned} $$

अब $\mathrm{S}^{\prime}(x)=0$ से $x=\frac{r}{2}$ प्राप्त होता है। क्योंकि सभी $x$ के लिए $\mathrm{S}^{\prime \prime}(x)<0$ है। अतः $\mathrm{S}^{\prime \prime}\left(\frac{r}{2}\right)<0$ है। इसलिए $x=\frac{r}{2}, \mathrm{~S}$ का उच्चतम बिंदु है। अतः दिए शंकु के अंतर्गत महत्तम वक्र पृष्ठ के बेलन की त्रिज्या शंकु की त्रिज्या की आधी होती है।
6.4.1 एक संवृत्त अंतराल में किसी फलन का उच्चतम और निम्नतम मान (Maximum and Minimum Values of a Function in a Closed Interval)
मान लीजिए $f(x)=x+2, x \in(0,1)$ द्वारा प्रदत्त एक प्रलन $f$ है।
ध्यान दीजिए कि $(0,1)$ पर फलन संतत है और इस अंतराल में न तो इसका कोई उच्चतम मान है और न ही इसका कोई निम्नतम मान है।
तथापि, यदि हम $f$ के प्रांत को संवृत्त अंतराल $[0,1]$ तक बढ़ा दें तब भी $f$ का शायद कोई स्थानीय उच्चतम (निम्नतम) मान नहीं होगा परंतु इसका निश्चित ही उच्चतम मान $3=f(1)$ और निम्नतम मान $2=f(0)$ हैं। $x=1$ पर $f$ का उच्चतम मान $3,[0,1]$ पर $f$ का निरपेक्ष उच्चतम मान (महत्तम मान) (absolute maximum value) या सार्वत्रिक अधिकतम मान (global maximum or greatest value) कहलाता है। इसी प्रकार, $x=0$ पर $f$ का निम्नतम मान $2,[0,1]$ पर $f$ का निरपेक्ष निम्नतम मान (न्यूनतम मान) (absolute minimum value) या सार्वत्रिक न्यूनतम मान (global minimum or least value) कहलाता है।
एक संवृत्त अंतराल $[a, b]$ पर परिभाषित किसी संतत फलन $f$ के संगत आकृति 6.19 में प्रदर्शित आलेख पर विचार कीजिए कि $x=b$ पर फलन $f$ का स्थानीय निम्नतम है
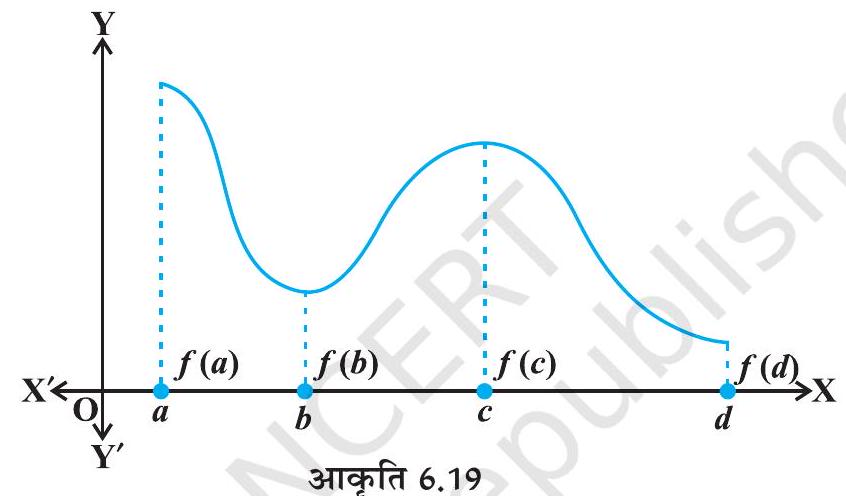
तथा स्थानीय निम्नतम मान $f(b)$ है। फलन का $x=c$ पर स्थानीय उच्चतम बिंदु है तथा स्थानीय उच्चतम मान $f(c)$ है।
साथ ही आलेख से यह भी स्पष्ट है कि $f$ का निरपेक्ष उच्चतम मान $f(a)$ तथा निरपेक्ष निम्नतम मान $f(d)$ है। इसके अतिरिक्त ध्यान दीजिए कि $f$ का निरपेक्ष उच्चतम (निम्नतम) मान स्थानीय उच्चतम (निम्नतम) मान से भिन्न है।
अब हम एक संवृत्त अंतराल I में एक फलन के निरपेक्ष उच्चतम और निरपेक्ष निम्नतम के विषय में दो परिणामों (बिना उपपत्ति) के कथन बताएँगे।
प्रमेय 5 मान लीजिए एक अंतराल $\mathrm{I}=[a, b]$ पर $f$ एक संतत फलन है। तब $f$ का निरपेक्ष उच्चतम मान होता है और $\mathrm{I}$ में कम से कम एक बार $f$ यह मान प्राप्त करता है तथा $f$ का निरपेक्ष निम्नतम मान होता है और $\mathrm{I}$ में कम से कम एक बार $f$ यह मान प्राप्त करता है।
प्रमेय 6 मान लीजिए संवृत्त अंतराल $\mathrm{I}$ पर $f$ एक अवकलनीय फलन है और मान लीजिए कि I का कोई आंतरिक बिंदु $c$ है। तब
(i) यदि $c$ पर $f$ निरपेक्ष उच्चतम मान प्राप्त करता है, तो $f^{\prime}(c)=0$
(ii) यदि $c$ पर $f$ निरपेक्ष निम्नतम मान प्राप्त करता है, तो $f^{\prime}(c)=0$
उपर्युक्त प्रमेयों के विचार से, दिए गए संवृत्त अंतराल में किसी फलन के निरपेक्ष उच्चतम मान और निरपेक्ष निम्नतम मान ज्ञात करने के लिए विधि निम्नलिखित हैं।
व्यावहारिक विधि (Working Rule)
चरण 1: दिए गए अंतराल में $f$ के सभी क्रांतिक बिंदु ज्ञात कीजिए अर्थात् $x$ के वह सभी मान ज्ञात कीजिए जहाँ या तो $f^{\prime}(x)=0$ या $f$ अवकलनीय नहीं है।
चरण 2: अंतराल के अंत्य बिंदु लीजिए।
चरण 3: इन सभी बिंदुओं पर (चरण 1 व 2 में सूचीबद्ध) $f$ के मानों की गणना कीजिए।
चरण 4: चरण 3 में गणना से प्राप्त $f$ के मानों में से उच्चतम और निम्नतम मानों को लीजिए। यही उच्चतम मान, $f$ का निरपेक्ष उच्चतम मान और निम्नतम मान, $f$ का निरपेक्ष निम्नतम मान होंगे।
उदाहरण 27 अंतराल $[1,5]$ में $f(x)=2 x^{3}-15 x^{2}+36 x+1$ द्वारा प्रदत्त फलन के निरपेक्ष उच्चतम और निरपेक्ष निम्नतम मानों को ज्ञात कीजिए।
हल हमें ज्ञात है
$$ \begin{aligned} f(x) & =2 x^{3}-15 x^{2}+36 x+1 \end{aligned} $$ $ \begin{aligned} या f^{\prime}(x) & =6 x^{2}-30 x+36=6(x-3)(x-2) \end{aligned} $
ध्यान दीजिए $f^{\prime}(x)=0$ से $x=2$ और $x=3$ प्राप्त होते हैं।
अब हम इन बिंदुओं और अंतराल $[1,5]$ के अंत्य बिंदुओं अर्थात् $x=1, x=2, x=3$ और $x=5$ पर $f$ के मान का परिकलन करेंगे। अब:
$$ \begin{aligned} & f(1)=2\left(1^{3}\right)-15\left(1^{2}\right)+36(1)+1=24 \\ & f(2)=2\left(2^{3}\right)-15\left(2^{2}\right)+36(2)+1=29 \\ & f(3)=2\left(3^{3}\right)-15\left(3^{2}\right)+36(3)+1=28 \\ & f(5)=2\left(5^{3}\right)-15\left(5^{2}\right)+36(5)+1=56 \end{aligned} $$
इस प्रकार, हम इस निष्कर्ष पर पहुँचते हैं कि अंतराल $[1,5]$ पर फलन $f$ के लिए $x=5$ पर निरपेक्ष उच्चतम मान 56 और $x=1$ पर निरपेक्ष निम्नतम मान 24 है।
उदाहरण 28 $ f(x)=12 x^{\frac{4}{3}}-6 x^{\frac{1}{3}}, x \in[-1,1]$ द्वारा प्रदत्त एक फलन $f$ के निरपेक्ष उच्चतम और निरपेक्ष निम्नतम मान ज्ञात कीजिए।
हल हमें ज्ञात है कि
$$ \begin{aligned} & f(x)=12 x^{\frac{4}{3}}-6 x^{\frac{1}{3}} \end{aligned} $$ $ \begin{aligned} या \qquad & f^{\prime}(x)=16 x^{\frac{1}{3}}-\frac{2}{x^{\frac{2}{3}}}=\frac{2(8 x-1)}{x^{\frac{2}{3}}} \end{aligned} $
इस प्रकार $f^{\prime}(x)=0$ से $x=\frac{1}{8}$ प्राप्त होता है। और ध्यान दीजिए कि $x=0$ पर $f^{\prime}(x)$ परिभाषित नहीं है। इसलिए क्रांतिक बिंदु $x=0$ और $x=\frac{1}{8}$ हैं। अब क्रांतिक बिंदुओं $x=0, \frac{1}{8}$ और अंतराल के अंत्य बिंदुओं $x=-1$ व $x=1$ पर फलन $f$ के मान का परिकलन करने से
$$ \begin{aligned} f(-1) & =12\left(-1^{\frac{4}{3}}\right)-6\left(-1^{\frac{1}{3}}\right)=18 \\ f(0) & =12(0)-6(0)=0 \\ f\left(\frac{1}{8}\right) & =12\left(\frac{1}{8}\right)^{\frac{4}{3}}-6\left(\frac{1}{8}\right)^{\frac{1}{3}}=\frac{-9}{4} \\ f(1) & =12\left(1^{\frac{4}{3}}\right)-6\left(1^{\frac{1}{3}}\right)=6 \end{aligned} $$
प्राप्त होते हैं। इस प्रकार हम इस निष्कर्ष पर पहुँचते है कि $x=-1$ पर $f$ का निरपेक्ष उच्चतम मान 18 है और $x=\frac{1}{8}$ पर $f$ का निरपेक्ष निम्नतम मान $\frac{-9}{4}$ है।
उदाहरण 29 शत्रु का एक अपाचे हेलिकॉप्टर वक्र $y=x^{2}+7$ के अनुदिश प्रदत्त पथ पर उड़ रहा है। बिंदु $(3,7)$ पर स्थित एक सैनिक अपनी स्थिति से न्यूनतम दूरी पर उस हेलिकॉप्टर को गोली मारना चाहता है। न्यूनतम दूरी ज्ञात कीजिए।
हल $x$ के प्रत्येक मान के लिए हेलिकॉप्टर की स्थिति बिंदु $\left(x, x^{2}+7\right)$ है। इसलिए $(3,7)$ पर स्थित सैनिक और हेलिकॉप्टर के बीच दूरी
$\sqrt{(x-3)^{2}+\left(x^{2}+7-7\right)^{2}}$, अर्थात् $\sqrt{(x-3)^{2}+x^{4}}$ है।
$ मान लीजिए कि \qquad f(x)=(x-3)^{2}+x^{4} $
$ या \qquad f^{\prime}(x)=2(x-3)+4 x^{3}=2(x-1)\left(2 x^{2}+2 x+3\right) $
इसलिए $f^{\prime}(x)=0$ से $x=1$ प्राप्त होता है तथा $2 x^{2}+2 x+3=0$ से कोई वास्तविक मूल प्राप्त नहीं होता है। पुन: अंतराल के अंत्य बिंदु भी नहीं है, जिन्हें उस समुच्चय में जोड़ा जाए जिनके लिए $f^{\prime}$ का मान शून्य है अर्थात् केवल एक बिंदु, नामतः $x=1$ ही ऐसा है। इस बिंदु पर $f$ का मान $f(1)=(1-3)^{2}+(1)^{4}=5$ से प्रदत्त है। इस प्रकार, सैनिक एवं हेलिकॉप्टर के बीच की दूरी $\sqrt{f(1)}=\sqrt{5}$ है।
ध्यान दीजिए कि $\sqrt{5}$ या तो उच्चतम मान या निम्नतम मान है। क्योंकि
$$ \sqrt{f(0)}=\sqrt{(0-3)^{2}+(0)^{4}}=3>\sqrt{5} \text { है। } $$
इससे यह निष्कर्ष निकला कि $\sqrt{f(x)}$ का निम्नतम मान $\sqrt{5}$ है। अतः सैनिक और हेलिकॉप्टर के बीच की निम्नतम दूरी $\sqrt{5}$ है।
प्रश्नावली 6.3
1. निम्नलिखित दिए गए फलनों के उच्चतम या निम्नतम मान, यदि कोई तो, ज्ञात कीजिए:
(i) $f(x)=(2 x-1)^{2}+3$
(ii) $f(x)=9 x^{2}+12 x+2$
(iii) $f(x)=-(x-1)^{2}+10$
(iv) $g(x)=x^{3}+1$
Show Answer
#missing2. निम्नलिखित दिए गए फलनों के उच्चतम या निम्नतम मान, यदि कोई हों, तो ज्ञात कीजिए:
(i) $f(x)=|x+2|-1$
(ii) $g(x)=-|x+1|+3$
(iii) $h(x)=\sin (2 x)+5$
(iv) $f(x)=|\sin 4 x+3|$
(v) $h(x)=x+1, x \in(-1,1)$
Show Answer
#missing3. निम्नलिखित फलनों के स्थानीय उच्चतम या निम्नतम, यदि कोई हों तो, ज्ञात कीजिए तथा स्थानीय उच्चतम या स्थानीय निम्नतम मान, जैसी स्थिति हो, भी ज्ञात कीजिए।
(i) $f(x)=x^{2}$
(ii) $g(x)=x^{3}-3 x$
(iii) $h(x)=\sin x+\cos x, 0<x<\frac{\pi}{2}$
(iv) $f(x)=\sin x-\cos x, 0<x<2 \pi$
$\begin{array}{ll}\text { (v) } f(x)=x^{3}-6 x^{2}+9 x+15 & \text { (vi) } g(x)=\frac{x}{2}+\frac{2}{x}, \quad x>0\end{array}$
(vii) $g(x)=\frac{1}{x^{2}+2} \quad$
(viii) $f(x)=x \sqrt{1-x}, 0<x<1$
Show Answer
#missing4. सिद्ध कीजिए कि निम्नलिखित फलनों का उच्चतम या निम्नतम मान नहीं है:
(i) $f(x)=e^{x}$
(ii) $g(x)=\log x$
(iii) $h(x)=x^{3}+x^{2}+x+1$
Show Answer
#missing5. प्रदत्त अंतरालों में निम्नलिखित फलनों के निरपेक्ष उच्चतम मान और निरपेक्ष निम्नतम मान ज्ञात कीजिए।
(i) $f(x)=x^{3}, x \in[-2,2]$
(ii) $f(x)=\sin x+\cos x, x \in[0, \pi]$
(iii) $f(x)=4 x-\frac{1}{2} x^{2}, x \in\left[-2, \frac{9}{2}\right]$
(iv) $f(x)=(x-1)^{2}+3, x \in[-3,1]$
Show Answer
#missing6. यदि लाभ फलन $p(x)=41-72 x-18 x^{2}$ से प्रदत्त है तो किसी कंपनी द्वारा
अर्जित उच्चतम लाभ ज्ञात कीजिए।
Show Answer
#missing7. अंतराल $[0,3]$ पर $3 x^{4}-8 x^{3}+12 x^{2}-48 x+25$ के उच्चतम मान ओर निम्नतम मान ज्ञात कीजिए।
Show Answer
#missing8. अंतराल $[0,2 \pi]$ के किन बिंदुओं पर फलन $\sin 2 x$ अपना उच्चतम मान प्राप्त करता है?
Show Answer
#missing9. फलन $\sin x+\cos x$ का उच्चतम मान क्या है?
Show Answer
#missing10. अंतराल $[1,3]$ में $2 x^{3}-24 x+107$ का महत्तम मान ज्ञात कीजिए। इसी फलन का अंतराल $[-3,-1]$ में भी महत्तम मान ज्ञात कीजिए।
Show Answer
#missing11. यदि दिया है कि अंतराल $[0,2]$ में $x=1$ पर फलन $x^{4}-62 x^{2}+a x+9$ उच्चतम मान प्राप्त करता है, तो $a$ का मान ज्ञात कीजिए।
Show Answer
#missing12. $[0,2 \pi]$ पर $x+\sin 2 x$ का उच्चतम और निम्नतम मान ज्ञात कीजिए।
Show Answer
#missing13. ऐसी दो संख्याएँ ज्ञात कीजिए जिनका योग 24 है और जिनका गुणनफल उच्चतम हो।
Show Answer
#missing14. ऐसी दो धन संख्याएँ $x$ और $y$ ज्ञात कीजिए ताकि $x+y=60$ और $x y^{3}$ उच्चतम हो।
Show Answer
#missing15. ऐसी दो धन संख्याएँ $x$ और $y$ ज्ञात कीजिए जिनका योग 35 हो और गुणनफल $x^{2} y^{5}$ उच्चतम हो।
Show Answer
#missing16. ऐसी दो धन संख्याएँ ज्ञात कीजिए जिनका योग 16 हो और जिनके घनों का योग निम्नतम हो।
Show Answer
#missing17. $18 \mathrm{~cm}$ भुजा के टिन के किसी वर्गाकार टुकड़े से प्रत्येक कोने पर एक वर्ग काटकर तथा इस प्रकार बनें टिन के फलकों को मोड़ कर ढक्कन रहित एक संदूक बनाना है। काटे जाने वाले वर्ग की भुजा कितनी होगी जिससे संदूक का आयतन उच्चतम हो?
Show Answer
#missing18. $45 \mathrm{~cm} \times 24 \mathrm{~cm}$ की टिन की आयताकार चादर के कोनों पर वर्ग काटकर तथा इस प्रकार बनें टिन के फलकों को मोड़कर ढक्कन रहित एक संदूक बनाना है। काटे जाने वाले वर्ग की भुजा कितनी होगी जिससे संदूक का आयतन उच्चतम हो।
Show Answer
#missing19. सिद्ध किजिए कि एक दिए वृत्त के अंतर्गत सभी आयतों में वर्ग का क्षेत्रफल उच्चतम होता है।
Show Answer
#missing20. सिद्ध किजिए कि प्रदत्त पृष्ठ एवं महत्तम आयतन के बेलन की ऊँचाई, आधार के व्यास के बराबर होती है।
Show Answer
#missing21. $100 \mathrm{~cm}^{3}$ आयतन वाले डिब्बे सभी बंद बेलनाकार (लंब वृत्तीय) डिब्बों में से न्यूनतम पृष्ठ क्षेत्रफल वाले डिब्बे की विमाएँ ज्ञात किजिए।
Show Answer
#missing22. एक $28 \mathrm{~cm}$ लंबे तार को दो टुकड़ों में विभक्त किया जाना है। एक टुकड़े से वर्ग तथा दूसरे वे वृत्त बनाया जाना है। दोनों टुकड़ों की लंबायीं कितनी होनी चाहिए जिससे वर्ग एवं वृत्त का सम्मिलित क्षेत्रफल न्यूनतम हो?
Show Answer
#missing23. सिद्ध कीजिए कि $R$ त्रिज्या के गोले के अंतर्गत विशालतम शंकु का आयतन, गोले के आयतन का $\frac{8}{27}$ होता है।
Show Answer
#missing24. सिद्ध कीजिए कि न्यूनतम पृष्ठ का दिए आयतन के लंब वृत्तीय शंकु की ऊँचाई, आधार की त्रिज्या की $\sqrt{2}$ गुनी होती है।
Show Answer
#missing25. सिद्ध कीजिए कि दी हुई तिर्यक ऊँचाई और महत्तम आयतन वाले शंकु का अर्ध शीर्ष कोण $\tan ^{-1} \sqrt{2}$ होता है।
Show Answer
#missing26. सिद्ध कीजिए कि दिए हुए पृष्ठ और महत्तम आयतन वाले लंब वृत्तीय शंकु का अर्ध शीर्ष कोण $\sin ^{-1}\left(\frac{1}{3}\right)$ होता है।
Show Answer
#missingप्रश्न संख्या 27 से 29 में सही उत्तर का चुनाव कीजिए।
27. वक्र $x^{2}=2 y$ पर $(0,5)$ से न्यूनतम दूरी पर स्थित बिंदु है:
(A) $(2 \sqrt{2}, 4)$
(B) $(2 \sqrt{2}, 0)$
(C) $(0,0)$
(D) $(2,2)$
Show Answer
#missing28. $x$, के सभी वास्तविक मानों के लिए $\frac{1-x+x^{2}}{1+x+x^{2}}$ का न्यूनतम मान है:
(A) 0
(B) 1
(C) 3
(D) $\frac{1}{3}$
Show Answer
#missing29. $[x(x-1)+1]^{\frac{1}{3}}, 0 \leq x \leq 1$ का उच्चतम मान है:
(A) $\left(\frac{1}{3}\right)^{\frac{1}{3}}$
(B) $\frac{1}{2}$
(C) 1
(D) 0
Show Answer
#missingविविध उदाहरण
उदाहरण 30 एक कार समय $t=0$ पर बिंदु $\mathrm{P}$ से चलना प्रारंभ करके बिंदु $\mathrm{Q}$ पर रूक जाती है। कार द्वारा $t$ सेकंड में तय की दूरी, $x$ मीटर में
$$ x=t^{2}\left(2-\frac{t}{3}\right) \text { द्वारा प्रदत्त है। } $$
कार को $\mathrm{Q}$ तक पहुँचने में लगा समय ज्ञात कीजिए और $\mathrm{P}$ तथा $\mathrm{Q}$ के बीच की दूरी भी ज्ञात कीजिए।
हल मान लीजिए $t$ सेकंड में कार का वेग $v$ है।
$ \begin{aligned} अब \qquad & x=t^{2}\left(2-\frac{t}{3}\right) \\ & v=\frac{d x}{d t}=4 t-t^{2}=t(4-t) \end{aligned} $
$ या \qquad v=0 \text { से } t=0 \text { या } t=4 \text { प्राप्त होते हैं। } $
अब $\mathrm{P}$ और $\mathrm{Q}$ पर कार का वेग $v=0$ है। इसलिए $\mathrm{Q}$ पर कार 4 सेकंडों में पहुँचेगी। अब 4 सेकंडों में कार द्वारा तय की गई दूरी निम्नलिखित है:
$$ x] _{t=4}=4^{2}\left(2-\frac{4}{3}\right)=16\left(\frac{2}{3}\right)=\frac{32}{3} \mathrm{~m} $$
उदाहरण 32 2 $\mathrm{~m}$ ऊँचाई का आदमी $6 \mathrm{~m}$ ऊँचे बिजली के खंभे से दूर $5 \mathrm{~km} / \mathrm{h}$ की समान चाल से चलता है। उसकी छाया की लंबायीं की वृद्धि दर ज्ञात कीजिए।
हल आकृति 6.21 में, मान लीजिए, $\mathrm{AB}$ एक बिजली का खंभा है। $\mathrm{B}$ बिंदु पर बल्ब है और मान लीजिए कि एक विशेष समय $t$ पर आदमी $\mathrm{MN}$ है। मान लीजिए $\mathrm{AM}=l \mathrm{~m}$ और व्यक्ति की छाया $\mathrm{MS}$ है। और मान लीजिए $\mathrm{MS}=s \mathrm{~m}$ है।
ध्यान दीजिए कि $\triangle \mathrm{ASB} \sim \triangle \mathrm{MSN}$
$ या \qquad \frac{\mathrm{MS}}{\mathrm{AS}}=\frac{\mathrm{MN}}{\mathrm{AB}} $
$ या \qquad \mathrm{AS}=3 s $

आकृति 6.21
[(क्योंकि $\mathrm{MN}=2 \mathrm{~m}$ और $\mathrm{AB}=6 \mathrm{~m}$ (दिया है)]
इस प्रकार $\mathrm{AM}=3 s-s=2 s$ है। परन्तु $\mathrm{AM}=l$ मीटर है।
इसलिए $\quad l=2 s$
अत: $\quad \frac{d l}{d t}=2 \frac{d s}{d t}$
क्योंकि $\frac{d l}{d t}=5 \mathrm{~km} / \mathrm{h}$ है। अतः छाया की लंबायीं में वृद्धि $\frac{5}{2} \mathrm{~km} / \mathrm{h}$ की दर से होती है।
उदाहरण 33 उन अंतरालों को ज्ञात कीजिए जिनमें फलन
$$ f(x)=\frac{3}{10} x^{4}-\frac{4}{5} x^{3}-3 x^{2}+\frac{36}{5} x+11 $$
(a) वर्धमान (b) ह्रासमान है।
हल हमें ज्ञात है कि
$$ \begin{aligned} f(x) & =\frac{3}{10} x^{4}-\frac{4}{5} x^{3}-3 x^{2}+\frac{36}{5} x+11 \\ f^{\prime}(x) & =\frac{3}{10}\left(4 x^{3}\right)-\frac{4}{5}\left(3 x^{2}\right)-3(2 x)+\frac{36}{5} \\ & =\frac{6}{5}(x-1)(x+2)(x-3) \end{aligned} $$ (सरल करने पर)
अब $f^{\prime}(x)=0$ से $x=1, x=-2$, और $x=3$ प्राप्त होते हैं। $x=1,-2$, और 3 वास्तविक रेखा को चार असंयुक्त अंतरालों नामतः $(-\infty,-2),(-2,1),(1,3)$ और $(3, \infty)$ में

आकृति 6.22
अंतराल $(-\infty,-2)$ को लीजिए अर्थात् जब $-\infty<x<-2$ है।
इस स्थिति में हम $x-1<0, x+2<0$ और $x-3<0$ प्राप्त करते हैं।
(विशेष रूप से $x=-3$ के लिए देखिए कि, $f^{\prime}(x)=(x-1)(x+2)(x-3)=(-4)(-1)(-6)<0)$
इसलिए, जब $-\infty<x<-2$ है, तब $f^{\prime}(x)<0$ है।
अतः $(-\infty,-2)$ में फलन $f$ ह्रासमान है।
अंतराल $(-2,1)$, को लीजिए अर्थात् जब $-2<x<1$ है।
इस दशा में $x-1<0, x+2>0$ और $x-3<0$ है।
(विशेष रूप से $x=0$, के लिए ध्यान दीजिए कि, $f^{\prime}(x)=(x-1)(x+2)(x-3)=(-1)(2)$ $(-3)=6>0)$
इसलिए जब $-2<x<1$ है, तब $f^{\prime}(x)>0$ है।
अतः $(-2,1)$ में फलन $f$ वर्धमान है।
अब अंतराल $(1,3)$ को लीजिए अर्थात् जब $1<x<3$ है। इस दशा में कि $x-1>0, x+2>0$ और $x-3<0$ है।
इसलिए, जब $1<x<3$ है, तब $f^{\prime}(x)<0$ है।
अतः $(1,3)$ में फलन $f$ ह्रासमान है।
अंत में अंतराल $(3, \infty)$, को लीजिए अर्थात् जब $3<x<\infty$ है। इस दशा में $x-1>0, x+2>0$ और $x-3>0$ है। इसलिए जब $x>3$ है तो $f^{\prime}(x)>0$ है।
अतः अंतराल $(3, \infty)$ में फलन $f$ वर्धमान है।
उदाहरण 34 सिद्ध कीजिए कि
$f(x)=\tan ^{-1}(\sin x+\cos x), x>0$ से प्रदत्त फलन
$f,\left(0, \frac{\pi}{4}\right)$ में निरंतर वर्धमान फलन है।
हल यहाँ
$ या \qquad \begin{aligned} f(x) & =\tan ^{-1}(\sin x+\cos x), x>0 \\ f^{\prime}(x) & =\frac{1}{1+(\sin x+\cos x)^{2}}(\cos x-\sin x) \\ & =\frac{\cos x-\sin x}{2+\sin 2 x} \end{aligned} $
(सरल करने पर) ध्यान दीजिए कि $\left(0, \frac{\pi}{4}\right)$ में सभी $x$ के लिए $2+\sin 2 x>0$ है।
$ इसलिए \qquad f^{\prime}(x)>0 \text { यदि } \cos x-\sin x>0 $
$ या \qquad f^{\prime}(x)>0 \text { यदि } \cos x>\sin x \text { या } \cot x>1 $
$ अब \qquad \cot x>1 \text { यदि } \tan x<1 \text {, अर्थात्, यदि } 0<x<\frac{\pi}{4} $
इसलिए अंतराल $\left(0, \frac{\pi}{4}\right)$ में $f^{\prime}(x)>0$ है।
अतः $\left(0, \frac{\pi}{4}\right)$ में $f$ एक वर्धमान फलन है।
उदाहरण 35 $3 \mathrm{~cm}$ त्रिज्या की एक वृत्ताकार डिस्क को गर्म किया जाता है। प्रसार के कारण इसकी त्रिज्या $0.05 \mathrm{~cm} / \mathrm{s}$ की दर से बढ़ रही है। वह दर ज्ञात कीजिए जिससे इसका क्षेत्रफल बढ़ रहा है जब इसकी त्रिज्या $3.2 \mathrm{~cm}$ है।
हल मान लीजिए कि दी गई तश्तरी की त्रिज्या $r$ और इसका क्षेत्रफल $\mathrm{A}$ है।
तब $\qquad \mathrm{A}=\pi r^{2}$
या $\qquad \frac{d \mathrm{~A}}{d t}=2 \pi r \frac{d r}{d t} \tag{शृंखलानियमद्वारा} $
अब त्रिज्या की वृद्धि की सन्निकट दर $=d r=\frac{d r}{d t} \Delta t=0.05 \mathrm{~cm} / \mathrm{s}$ है।
इसलिए क्षेत्रफल में वृद्धि की सन्निकट दर निम्नांकित है
$ \begin{aligned} d A & =\frac{d A}{d t}(\Delta t)=2 \pi r(\frac{d r}{d t} \Delta t) \\ & =2 \pi(3.2)(0.05)=0.320 \pi cm^{2} / s \quad(r=3.2 cm) \end{aligned} $
उदाहरण 36 ऐल्यूमिनियम की $3 \mathrm{~m} \times 8 \mathrm{~m}$ की आयताकार चादर के प्रत्येक कोने से समान वर्ग काटने पर बने एल्यूमिनियम के फलकों को मोड़कर ढक्कन रहित एक संदूक बनाना है। इस प्रकार बने संदूक का अधिकतम आयतन ज्ञात कीजिए।
हल मान लीजिए कि अलग किए गए वर्ग की भुजा की लंबायों $x \mathrm{~m}$ है, तब बाक्स की ऊँचाई $x$, लंबायों $8-2 x$ और चौड़ाई $3-2 x$ (आकृति 6.23) है। यदि संदूक का आयतन $\mathrm{V}(x)$ है तब
 आकृति 6.23
आकृति 6.23
$ \begin{aligned} V(x) & =x(3-2 x)(8-2 x) \\ & =4 x^{3}-22 x^{2}+24 x \end{aligned} $
अत: $\qquad \begin{cases}\mathrm{V}^{\prime}(x)=12 x^2-44 x+24=4(x-3)(3 x-2) \\ \mathrm{V}^{\prime \prime}(x)=24 x-44\end{cases}$
अब $\qquad \mathrm{V}^{\prime}(x)=0 \text { से } x=\frac{2}{3} \text { और } x=3 \text { प्राप्त होता है। परन्तु } x \neq 3 \text { (क्यों?) }$
इसलिए $\quad x=\frac{2}{3}$ अब $\qquad V^{\prime \prime}\left(\frac{2}{3}\right)=24\left(\frac{2}{3}\right)-44=-28<0$
इसलिए $x=\frac{2}{3}$ उच्चतम का बिंदु है अर्थात् यदि हम चादर के प्रत्येक किनारे से $\frac{2}{3} \mathrm{~m}$ भुजा के वर्ग हटा दें और शेष चादर से एक संदूक बनाए तो संदूक का आयतन अधिकतम होगा जो निम्नलिखित है:
$\mathrm{V}\left(\frac{2}{3}\right)=4\left(\frac{2}{3}\right)^{3}-22\left(\frac{2}{3}\right)^{2}+24\left(\frac{2}{3}\right)=\frac{200}{27} \mathrm{~m}^{3}$
उदाहरण 37 एक निर्माता $\mathrm{Rs}\left(5-\frac{x}{100}\right)$ प्रति इकाई की दर से $x$ इकाइयाँ बेच सकता है। $x$ इकाइयों का उत्पाद मूल्य $\operatorname{Rs}\left(\frac{x}{5}+500\right)$ है। इकाइयों की वह संख्या ज्ञात कीजिए जो उसे अधिकतम लाभ अर्जित करने के लिए बेचनी चाहिए।
हल मान लीजिए $x$ इकाइयों का विक्रय मूल्य $\mathrm{S}(x)$ है और $x$ इकाइयों का उत्पाद मूल्य $\mathrm{C}(x)$ है। तब हम पाते हैं
$$ S(x)=\left(5-\frac{x}{100}\right) x=5 x-\frac{x^{2}}{100} $$
और $\qquad \mathrm{C}(x)=\frac{x}{5}+500$
इस प्रकार, लाभ फलन $\mathrm{P}(x)$ निम्नांकित द्वारा प्रदत्त है।
अर्थात् $\qquad \mathrm{P}(x)=\mathrm{S}(x)-\mathrm{C}(x)=5 x-\frac{x^{2}}{100}-\frac{x}{5}-500$
$$ \mathrm{P}(x)=\frac{24}{5} x-\frac{x^{2}}{100}-500 $$
या $\qquad \mathrm{P}^{\prime}(x)=\frac{24}{5}-\frac{x}{50}$ अब $\qquad \mathrm{P}^{\prime}(x)=0$ से $x=240$ प्राप्त होता है और $\mathrm{P}^{\prime \prime}(x)=\frac{-1}{50}$. इसलिए $\mathrm{P}^{\prime \prime}(240)=\frac{-1}{50}<0$ है।
इस प्रकार $x=240$ उच्चतम का बिंदु है। अतः निर्माता अधिकतम लाभ अर्जित कर सकता है यदि वह 240 इकाइयाँ बेचता है।
अध्याय 6 पर विविध प्रश्नावली
1. सिद्ध कीजिए कि $f(x)=\frac{\log x}{x}$ द्वारा प्रदत्त फलन $x=e$ पर उच्चतम है।
Show Answer
#missing2. किसी निश्चित आधार $b$ के एक समद्विबाहु त्रिभुज की समान भुजाएँ $3 \mathrm{~cm} / \mathrm{s}$ की दर से घट रहीं है। उस समय जब त्रिभुज की समान भुजाएँ आधार के बराबर हैं, उसका क्षेत्रफल कितनी तेजी से घट रहा है।
Show Answer
#missing3. अंतराल ज्ञात कीजिए जिन पर
$$ f(x)=\frac{4 \sin x-2 x-x \cos x}{2+\cos x} $$
से प्रदत्त फलन $f$ (i) निरंतर वर्धमान (ii) निरंतर ह्रासमान है।
Show Answer
#missing4. अंतराल ज्ञात कीजिए जिन पर $f(x)=x^{3}+\frac{1}{x^{3}}, x \neq 0$ से प्रदत्त फलन
(i) वर्धमान
(ii) ह्रासमान है।
Show Answer
#missing5. दीर्घवृत्त $\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$ के अंतर्गत उस समद्विबाहु त्रिभुज का महत्तम क्षेत्रफल ज्ञात कीजिए जिसका शीर्ष दीर्घ अक्ष का एक सिरा है।
Show Answer
#missing6. आयताकार आधार व आयताकार दीवारों की $2 \mathrm{~m}$ गहरी और $8 \mathrm{~m}^{3}$ आयतन की एक बिना ढक्कन की टंकी का निर्माण करना है। यदि टंकी के निर्माण में आधार के लिए Rs $70 / \mathrm{m}^{2}$ और दीवारों पर $\mathrm{Rs} 45 / \mathrm{m}^{2}$ व्यय आता है तो निम्नतम खर्च से बनी टंकी की लागत क्या है?
Show Answer
#missing7. एक वृत्त और एक वर्ग के परिमापों का योग $k$ है, जहाँ $k$ एक अचर है। सिद्ध कीजिए कि उनके क्षेत्रफलों का योग निम्नतम है, जब वर्ग की भुजा वृत्त की त्रिज्या की दुगुनी है।
Show Answer
#missing8. किसी आयत के ऊपर बने अर्धवृत्त के आकार वाली खिड़की है। खिड़की का संपूर्ण परिमाप $10 \mathrm{~m}$ है। पूर्णतया खुली खिड़की से अधिकतम प्रकाश आने के लिए खिड़की की विमाएँ ज्ञात कीजिए।
Show Answer
#missing9. त्रिभुज की भुजाओं से $a$ और $b$ दूरी पर त्रिभुज के कर्ण पर स्थित एक बिंदु है। सिद्ध कीजिए कि कर्ण की न्यूनतम लंबाई
$\left(a^{\frac{2}{3}}+b^{\frac{2}{3}}\right)^{\frac{3}{2}}$ है।
Show Answer
#missing10. उन बिंदुओं को ज्ञात कीजिए जिन पर $f(x)=(x-2)^{4}(x+1)^{3}$ द्वारा प्रदत्त फलन $f$ का,
(i) स्थानीय उच्चतम बिंदु है
(ii) स्थानीय निम्नतम बिंदु है
(iii) नत परिवर्तन बिंदु है।
Show Answer
#missing11. $f(x)=\cos ^{2} x+\sin x, x \in[0, \pi]$ द्वारा प्रदत्त फलन
$f$ का निरपेक्ष उच्चतम और निम्नतम मान ज्ञात कीजिए।
Show Answer
#missing12. सिद्ध कीजिए कि एक $r$ त्रिज्या के गोले के अंतर्गत उच्चतम आयतन के लंब वृत्तीय शंकु की ऊँचाई $\frac{4 r}{3}$ है।
Show Answer
#missing13. मान लीजिए $[a, b]$ पर परिभाषित एक फलन $f$ है इस प्रकार कि सभी $x \in(a, b)$ के लिए $f^{\prime}(x)>0$ है तो सिद्ध कीजिए कि $(a, b)$ पर $f$ एक वर्धमान फलन है।
Show Answer
#missing14. सिद्ध कीजिए कि एक $R$ त्रिज्या के गोले के अंतर्गत अधिकतम आयतन के बेलन की ऊँचाई $\frac{2 \mathrm{R}}{\sqrt{3}}$ है। अधिकतम आयतन भी ज्ञात कीजिए।
Show Answer
#missing15. सिद्ध कीजिए कि अर्द्धशीर्ष कोण $\alpha$ और ऊँचाई $h$ के लंब वृत्तीय शंकु के अंतर्गत अधिकतम आयतन के बेलन की ऊँचाई, शंकु के ऊँचाई की एक तिहाई है और बेलन का अधिकतम आयतन $\frac{4}{27} \pi h^{3} \tan ^{2} \alpha$ है। 19 से 24 तक के प्रश्नों के सही उत्तर चुनिए।
Show Answer
#missing16. एक $10 \mathrm{~m}$ त्रिज्या के बेलनाकार टंकी में $314 \mathrm{~m}^{3} / \mathrm{h}$ की दर से गेहूँ भरा जाता है। भरे गए गेहूँ की गहराई की वृद्धि दर है:
(A) $1 \mathrm{~m} / \mathrm{h}$
(B) $0.1 \mathrm{~m} / \mathrm{h}$
(C) $1.1 \mathrm{~m} / \mathrm{h}$
(D) $0.5 \mathrm{~m} / \mathrm{h}$
Show Answer
#missingसारांश
-
यदि एक राशि $y$ एक दूसरी राशि $x$ के सापेक्ष किसी नियम $y=f(x)$ को संतुष्ट करते हुए परिवर्तित होती है तो $\frac{d y}{d x}\left(\right.$ या $\left.f^{\prime}(x)\right) x$ के सापेक्ष $y$ के परिवर्तन की दर को निरूपित करता है और $\left.\frac{d y}{d x}\right] _{x=x _{0}}\left(\right.$ या $\left.f^{\prime}\left(x _{0}\right)\right) x=x _{0}$ पर) $x$ के सापेक्ष $y$ के निरूपित की दर को निरूपित करता है।
-
यदि दो राशियाँ $x$ और $y, t$ के सापेक्ष परिवर्तित हो रही हों अर्थात् $x=f(t)$ और $y=g(t)$, तब शृंखला नियम से
$$ \frac{d y}{d x}=\frac{d y}{d t} / \frac{d x}{d t}, \text { यदि } \frac{d x}{d t} \neq 0 $$
- एक फलन $f$
(a) अंतराल $[a, b]$ में वर्धमान है यदि
$$ [a, b] \text { में } x _{1}<x _{2} \Rightarrow f\left(x _{1}\right) \leq f\left(x _{2}\right) \text {, सभी } x _{1}, x _{2} \in(a, b) \text { के लिए } $$
विकल्पतः यदि प्रत्येक $x \in[a, b]$ के लिए $f^{\prime}(x) \geq 0$, है।
(b) अंतराल $[a, b]$ में ह्रासमान है यदि
$$ [a, b] \text { में } x _{1}<x _{2} \Rightarrow f\left(x _{1}\right) \geq f\left(x _{2}\right) \text {, सभी } x _{1}, x _{2} \in(a, b) \text { के लिए } $$
विकल्पतः यदि प्रत्येक $x \in[a, b]$ के लिए $f^{\prime}(x) \leq 0$ है।
-
फलन $f$ के प्रांत में एक बिंदु $c$ जिस पर या तो $f^{\prime}(c)=0$ या $f$ अवकलनीय नहीं है, $f$ का क्रांतिक बिंदु कहलाता है।
-
प्रथम अवकलज परीक्षण मान लीजिए एक विवृत्त अंतराल I पर फलन $f$ परिभाषित है। मान लीजिए $\mathrm{I}$ में एक क्रांतिक बिंदु $c$ पर फलन $f$ संतत है तब
(i) जब $x$ बिंदु $c$ के बायीं ओर से दायीं ओर बढ़ता है तब $f^{\prime}(x)$ का चिह्न धन से ऋण में परिवर्तित होता है अर्थात् $c$ के बायीं ओर और पर्याप्त निकट प्रत्येक बिंदु पर यदि $f^{\prime}(x)>0$ तथा $c$ के दायीं ओर और पर्याप्त निकट प्रत्येक बिंदु पर यदि $f^{\prime}(x)<0$ तब $c$ स्थानीय उच्चतम का एक बिंदु है।
(ii) जब $x$ बिंदु $c$ के बायीं ओर से दायीं ओर बढ़ता है तब $f^{\prime}(x)$ का चिह्न ऋण से धन में परिवर्तित होता है अर्थात् $c$ के बायीं ओर और पर्याप्त निकट प्रत्येक बिंदु पर यदि $f^{\prime}(x)<0$ तथा $c$ के दायीं ओर और पर्याप्त निकट प्रत्येक बिंदु पर यदि $f^{\prime}(x)>$ 0 तब $c$ स्थानीय निम्नतम का एक बिंदु है।
(iii) जब $x$ बिंदु $c$ के बायीं ओर से दायीं ओर बढ़ता है तब $f^{\prime}(x)$ परिवर्तित नहीं होता है तब $c$ न तो स्थानीय उच्चतम का बिंदु है और न ही स्थानीय निम्नतम का बिंदु। वास्तव में इस प्रकार का बिंदु एक नति परिवर्तन बिंदु है।
- द्वितीय अवकलज परीक्षण मान लीजिए एक अंतराल I पर $f$ एक परिभाषित फलन है और $c \in \mathrm{I}$ है। मान लीजिए $f, c$ पर लगातार दो बार अवकलनीय है। तब
(i) यदि $f^{\prime}(c)=0$ और $f^{\prime \prime}(c)<0$ तब $x=c$ स्थानीय उच्चतम का एक बिंदु है। $f$ का स्थानीय उच्चतम मान $f(c)$ है।
(ii) यदि $f^{\prime}(c)=0$ और $f^{\prime \prime}(c)>0$ तब $x=c$ स्थानीय निम्नतम का एक बिंदु है। इस स्थिति में $f$ का स्थानीय निम्नतम मान $f(c)$ है।
(iii) यदि $f^{\prime}(c)=0$ और $f^{\prime \prime}(c)=0$, तब यह परीक्षण असफल रहता है।
इस स्थिति में हम पुनः वापस प्रथम अवकलज परीक्षण का प्रयोग करते हैं और यह ज्ञात करते हैं कि $c$ उच्चतम, निम्नतम या नति परिवर्तन का बिंदु है।
- निरपेक्ष उच्चतम और निरपेक्ष निम्नतम मानों को ज्ञात करने की व्यावहारिक विधि है:
चरण 1: अंतराल में $f$ के सभी क्रांतिक बिंदु ज्ञात कीजिए अर्थात् $x$ के वे सभी मान ज्ञात कीजिए जहाँ या तो $f^{\prime}(x)=0$ या $f$ अवकलनीय नहीं है।
चरण 2: अंतराल के अंत्य बिंदु लीजिए।
चरण 3: (चरण 1 व 2 से प्राप्त) सभी बिंदुओं पर $f$ के मानों की गणना कीजिए।
चरण 4: चरण 3 में गणना से प्राप्त $f$ के सभी मानों में से उच्चतम और निम्नतम मानों को लीजिए। यही उच्चतम मान, $f$ का निरपेक्ष उच्चतम मान और निम्नतम मान, $f$ का निरपेक्ष निम्नतम मान होंगे।










