wave-optics Question 27
Question: Q. 4. (i) State the essential conditions for diffraction of light.
(ii) Explain diffraction of light due to a narrow single slit and the formation of pattern of fringes on the screen. (iii) Find the relation for width of central maxima in terms of wavelength ’ $\lambda$ ‘, width of slit ’ $a$ ‘, and separation between slit and screen ’ $D$ ‘.
(iv) If the width of the slit is made double the original width, how does it affect the size and intensity of the central band?
] [Foreign 2016]
Show Answer
Solution:
Ans. (i) Size of slit/aperture must be smaller than of the
(ii) order of wavelength of light.
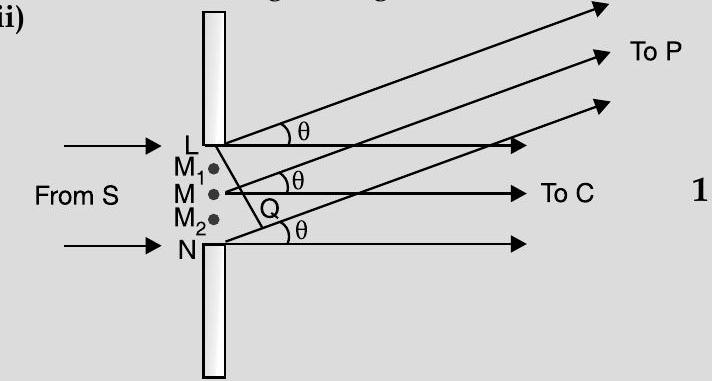
Single slit diffraction is explained by treating different parts of the wavefront at the slit as sources of secondary wavelets.
At the central point $C$ on the screen, $\theta$ is zero. All path differences are zero and hence all the parts of the slit contribute in phase and give maximum intensity at $C$.
At any other point $P$, the path difference between two edges of the slit is
$$ \begin{aligned} N P-L P & =N Q \ & =a \sin \theta \approx a \theta \because \sin \theta \text { is sprall } \end{aligned} $$
Any point $P$, is direction $\theta$, is a location of minima if $\quad a \theta=n \lambda$
This can be explained by dividing the slitinto even number of parts. The path difference between waves from successive parts is $180^{\circ}$ out of phase and hence cancel each leading to minima. $1 / 2$ Any point $P$, in direction $Q$, is alocation of maxima if
$$ a \theta=\left(n+\frac{1}{2}\right) \lambda $$
This can be explained by dividing the slit into odd number of parts. The contributions from successive parts cancel in pairs because of $180^{\circ}$ phase difference. The unpaired part produces intensity at $P$, leading to a maxima.
(iii) If $\theta$ is the direction of first minima, then
$$ \begin{aligned} a \theta & =\lambda \ \Rightarrow \quad \theta & =\frac{\lambda}{a} \end{aligned} $$
Angular width of central maxima
$$ \begin{align*} & =2 \theta \ & =\frac{2 \lambda}{\alpha} \end{align*} $$
Linear width of central maxima,
$$ \beta=\frac{2 \lambda D}{a}=2 \theta \times D $$
(iv) If ’ $a$ ’ is doubled, $\beta$ becomes half Intensity becomes 4 times.
[CBSE Marking Scheme 2016]
