SATHEE: moving-charges-and-magnetism Question 13

moving-charges-and-magnetism Question 13

Question: Q. 5. (i) State Biot-Savart’s law and express this law in the vector form.

(ii) Two identical circular coils, $P$ and $Q$ each of radius $R$ , carrying currents $1 A$ and $\sqrt{3}$ A respectively, are placed concentrically and perpendicular to each other lying in the $X Y$ and $Y Z$ planes. Find the magnitude and direction of the net magnetic field at the centre of the coils.

U] [O.D. I, III 2017]

Show Answer

Solution:

Ans. (i) Statement of Biot-Savart’s law

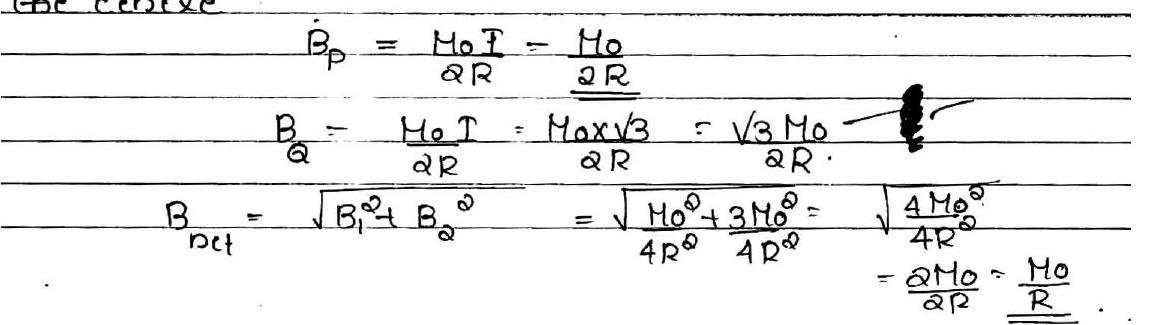
(ii) Magnitude of magnetic field at centre Direction of magnetic field

(i) It states that magnetic field strength, $\vec{d B}$ due to a current element, $I \vec{d}$ at a point, having a position vector $\vec{r}$ relative to the current element, is found to depend (i) directly on the current element, (ii) inversely on the square of the distance $| \vec{r} |$ (iii) directly on the sine of angle between the current element and the position vector $\vec{r}$ .

In vector notation,

$\vec{d B} = \frac{μ_{0}}{4 π} \frac{I \vec{d l} \times \vec{r}}{| \vec{r} |^{3}}$

Alternatively,

$(\vec{d B} = \frac{μ_{0}}{4 π} \cdot \frac{I \vec{d l} \times \hat{r}}{| \vec{r} |^{2}})$

(ii)

$\begin{aligned} B_{P} & = \frac{μ_{0} \times 1}{2 R} = \frac{μ_{0}}{2 R} B_{Q} & = \frac{μ_{0} \times \sqrt{3}}{2 R} = \frac{μ_{0} \sqrt{3}}{2 R} B & = \sqrt{B_{P}^{2} + B_{Q}^{2}} = \frac{μ_{0}}{R} \end{aligned}$

(along Z-direction) $1 / 2$

(along $X$ -direction) $1 / 2$

$∴$

This net magnetic field $B$ , is inclined to the field $B_{P}$ , at an angle $θ$ where

$\begin{aligned} \tan θ = \sqrt{3} & (or θ = \tan^{- 1} \sqrt{3} = 60^{\circ}) \end{aligned}$

(in XZ plane) $1 / 2$

Biot-Savart Law

Beot Bavast’s haw stakes thal the

magnetic fceld due to a cuseent eteroonc dl

a) adestance $\vec{x}$ . from it is gever by

$\sqrt{d β} α d B \propto I$ dBad $d B α sen θ$

10 vectoe from

$\vec{d B} = \frac{140}{4 π} I \frac{(π^{⊤} \times \vec{r})}{x^{3}}$

Beal saciast’s law geves the magnetic fold due to a culenc element $a t$ a porncrdestance $∵$

The magnetic fieid die to ebe coil p at the centre

[Topper’s Answer 2017]

Moving Charges And Magnetism

moving-charges-and-magnetism Question 13

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Moving Charges And Magnetism

moving-charges-and-magnetism Question 13

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu