Solution:

Ans. Rate of change of flux $=\frac{d \phi}{d t}=\left(\pi l^{2}\right) B_{0} \lambda \frac{d z}{d t}$

$$ =I R $$

$$ I=\left(\pi l^{2} \lambda\right) B_{0} \frac{v}{R} $$

Energy lost per second $=I^{2} R=\left(\pi l^{2} \lambda\right)^{2} B_{0}{ }^{2} \frac{v^{2}}{R} \quad 1 / 2$

Rate of change in P.E. $=m g \frac{d z}{d t}=m g v$

$$ \begin{aligned} m g v & =\left(\pi l^{2} \lambda\right)^{2} B_{0}^{2} \frac{v^{2}}{R} \ v & =\frac{m g R}{\left(\pi l^{2} \lambda\right)^{2} B_{0}^{2}} \end{aligned} $$

[CBSE Marking Scheme 2016]

Q1] Q. 3. Figure shows a metallic rod $P Q$ of length $l$, resting on the smooth horizontal rails $A B$ positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer $G$ connects the rails through a switch $K$. Assume the magnetic field to be uniform. Given the resistance of the closed loop containing the rod is $R$.

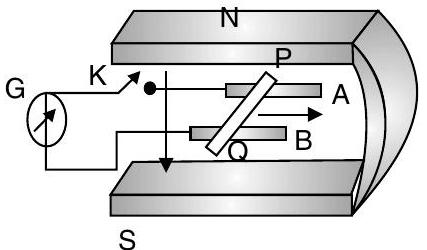
(i) Suppose $K$ is open and the rod is moved with a speed $v$ in the direction shown. Find the polarity and magnitude of induced emf.

(ii) With $K$ open and the rod moving uniformly, there is no net force on the electrons in the $\operatorname{rod} P Q$ even though they do experience magnetic force due to motion of the rod. Explain.

(iii) What is the induced emf in the moving rod if the magnetic field is parallel to the rails instead of being perpendicular?

U] [CBSE SQP 2017-18] [NCERT Exemplar]

Ans. (i) $\quad|\varepsilon|=B v l$

$P$ is positive end

$Q$ is negative end

1

(ii) Magnetic force gets cancelled by electric force that generates due to extra charge of opposite sign at rod ends. (iii) Induced emf is zero as motion of rod not cutting field lines

[CBSE Marking Scheme 2017]