alternating-currents Question 33
Question: Q. 1. An inductor of reactance
[NCERT Exemplar]
Show Answer
Solution:
Ans. Correct option : (c)
Explanation :
Here,
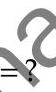
We know that, average power dissipated in the circuit is given by,
Question: Q. 1. An inductor of reactance
[NCERT Exemplar]
Solution:
Ans. Correct option : (c)
Explanation :
Here,
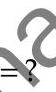
We know that, average power dissipated in the circuit is given by,
© 2024 Copyright SATHEE
Powered by Prutor@IITK
Welcome to SATHEE !
Select from 'Menu' to explore our services, or ask SATHEE to get started. Let's embark on this journey of growth together! 🌐📚🚀🎓
I'm relatively new and can sometimes make mistakes.
If you notice any error, such as an incorrect solution, please use the thumbs down icon to aid my learning.
To begin your journey now, click on "I understand".