Ray Optics and Optical Instruments - Result Question 59
62. For the angle of minimum deviation of a prism to be equal to its refracting angle, the prism must be made of a material whose refractive index :
(a) lies between $\sqrt{2}$ and 1
[2012M]
(b) lies between 2 and $\sqrt{2}$
(c) is less than 1
(d) is greater than 2
Show Answer
Answer:
Correct Answer: 62. (b)
Solution:
- (b)
(b)
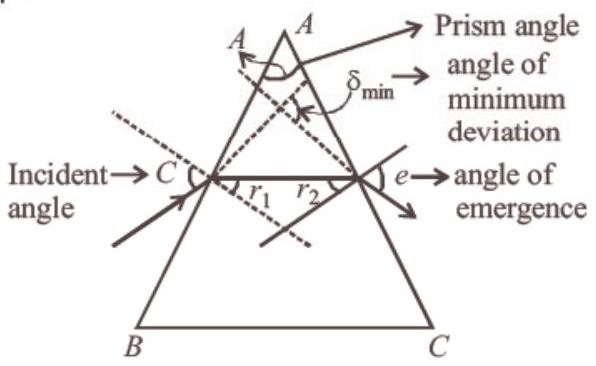
The angle of minimum deviation is given as $\delta _{\text{min }}=i+e-A$
for minimum deviation
$\delta _{\text{min }}=A$ then
$2 A=i+e$
in case of $\delta _{\min }, i=e$
$ \begin{aligned} 2 A & =2 i \quad r_1=r_2=\frac{A}{2} \\ i & =A=90^{\circ} \end{aligned} $
from Snell’s law
$1 \sin i=n \sin r_1$
$\sin A=n \sin \frac{A}{2}$
$2 \sin \frac{A}{2} \cos \frac{A}{2}=n \sin \frac{A}{2}$
$2 \cos \frac{A}{2}=n$
when $A=90^{\circ}=i _{\text{min }}$
then $\quad n _{\text{min }}=\sqrt{2}$
$i=A=0 \quad n _{\text{max }}=2$










