Ray Optics and Optical Instruments - Result Question 50
52. A ray is incident at an angle of incidence $i$ on one surface of a small angle prism (with angle of prism A) and emerges normally from the opposite surface. If the refractive index of the material of the prism is $\mu$, then the angle of incidence is nearly equal to :
[2020]
(a) $\frac{2 A}{\mu}$
(b) $\mu A$
(c) $\frac{\mu A}{2}$
(d) $\frac{A}{2 \mu}$
Show Answer
Answer:
Correct Answer: 52. (b)
Solution:
- (b) Light ray emerges normally from another surface, hence, $e($ angle of emergence $)=0$
$\therefore r_2=0 \quad r_1+r_2=A \Rightarrow r_1=A$
$\mu_1 \cdot \sin i=\mu_2 \cdot \sin r$
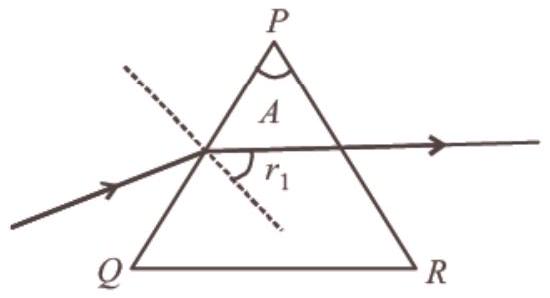
Applying Snell’s law on first surface $P Q$
$\Rightarrow \mu_1 \cdot \sin i=\mu_2 \cdot \sin r_1 \Rightarrow \sin i=\mu \sin A$
For small angles $(\sin \theta \approx \theta$ )
$\therefore i=\mu A$










