Ray Optics and Optical Instruments - Result Question 4
4. A rod of length $10 cm$ lies along the principal axis of a concave mirror of focal length $10 cm$ in such a way that its end closer to the pole is $20 cm$ away from the mirror. The length of the image is :
[2012M]
(a) $10 cm$
(b) $15 cm$
(c) $2.5 cm$
(d) $5 cm$
Show Answer
Answer:
Correct Answer: 4. (d)
Solution:
(d) According to the condition,
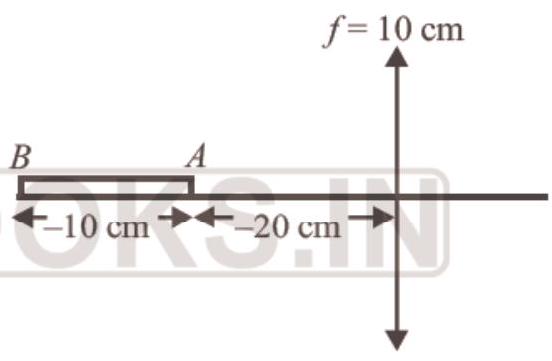
The focal length of the mirror
$-\frac{1}{f}=\frac{1}{v}+\frac{1}{u}$
For $A$ end of the rod the image distance When $u_1=-20 cm$
$\Rightarrow \frac{-1}{10}=\frac{1}{v_1}-\frac{1}{20}$
$\frac{1}{v_1}=\frac{-1}{10}+\frac{1}{20}=\frac{-2+1}{20}$
$v_1=-20 cm$
For when $u_2=-30 cm$
$\frac{1}{f}=\frac{1}{v_2}-\frac{1}{30}$
$\frac{1}{v_2}=\frac{-1}{10}+\frac{1}{30}=\frac{-30+10}{300}=\frac{-20}{300}$
$v_2=-15 cm$
$L=v_2-v_1=-15-(-20)$
$L=5 cm$










