States of Matter 1 Question 47
47. A closed tank has two compartments $A$ and $B$, both filled with oxygen (assumed to be ideal gas). The partition separating the two compartments is fixed and is a perfect heat insulator (Fig. 1). If the old partition is replaced by a new partition which can slide and conduct heat but does not allow the gas to leak across (Fig. 2), the volume (in $\mathrm{m}^{3}$ ) of the compartment $A$ after the system attains equilibrium is
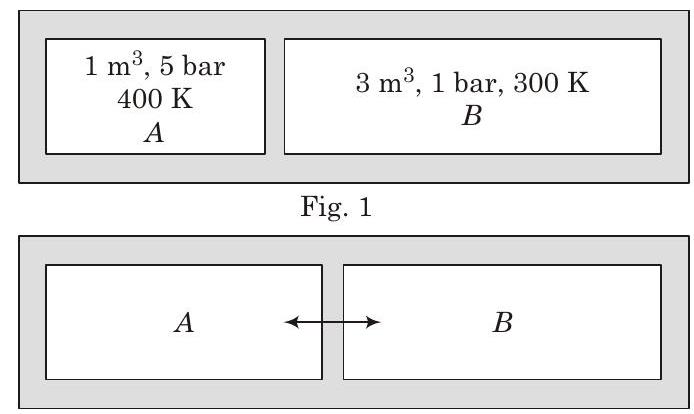
Fig. 2
(2018 Adv)
Assertion and Reason
Read the following questions and answer as per the direction given below :
(a) Statement I is correct; Statement II is correct; Statement II is the correct explanation of Statement I
(b) Statement I is correct; Statement II is correct; Statement II is not the correct explanation of Statement I
(c) Statement I is correct; Statement II is incorrect
(d) Statement I is incorrect; Statement II is correct
Show Answer
Solution:
- Given $p_{1}=5$ bar, $V_{1}=1 \mathrm{~m}^{3}, T_{1}=400 \mathrm{~K}$
So, $\quad n_{1}=\frac{5}{400 R}$
(from $p V=n R T$ )
Similarly, $p_{2}=1$ bar, $V_{2}=3 \mathrm{~m}^{3}, T_{2}=300 \mathrm{~K}, n_{2}=\frac{3}{300 R}$
Let at equilibrium the new volume of $A$ will be $(1+x)$
So, the new volume of $B$ will be $(3-x)$
Now, from the ideal gas equation.
$$ \frac{p_{1} V_{1}}{n_{1} R T_{1}}=\frac{p_{2} V_{2}}{n_{2} R T_{2}} $$
and at equilibrium (due to conduction of heat)
$$ \begin{aligned} \frac{p_{1}}{T_{1}} & =\frac{p_{2}}{T_{2}} \ \text { So, } \quad \frac{V_{1}}{n_{1}} & =\frac{V_{2}}{n_{2}} \text { or } V_{1} n_{2}=V_{2} n_{1} \end{aligned} $$
After putting the values
$$ \begin{aligned} & (1+x) \times \frac{3}{300 R}=(3-x) \times \frac{5}{400 R} \text { or }(1+x)=\frac{(3-x) 5}{4} \ & \text { or } 4(1+x)=15-5 x \text { or } 4+4 x=15-5 x \text { or } x=\frac{11}{9} \end{aligned} $$
Hence, new volume of $A$ i.e., $(1+x)$ will comes as $1+\frac{11}{9}=\frac{20}{9}$ or 2.22










